Skip to main content
Springer Nature Link
Log in

One-Way and Fully-Coupled FE2 Methods for Heterogeneous Elasticity and Plasticity Problems: Parallel Scalability and an Application to Thermo-Elastoplasticity of Dual-Phase Steels

  • Conference paper
  • First Online:
Software for Exascale Computing - SPPEXA 2013-2015

Part of the book series: Lecture Notes in Computational Science and Engineering ((LNCSE,volume 113))

Abstract

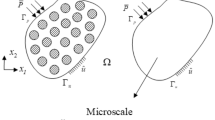
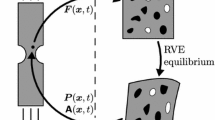
In this paper, aspects of the two-scale simulation of dual-phase steels are considered. First, we present two-scale simulations applying a top-down one-way coupling to a full thermo-elastoplastic model in order to study the emerging temperature field. We find that, for our purposes, the consideration of thermo-mechanics at the microscale is not necessary. Second, we present highly parallel fully-coupled two-scale FE2 simulations, now neglecting temperature, using up to 458, 752 cores of the JUQUEEN supercomputer at Forschungszentrum Jülich. The strong and weak parallel scalability results obtained for heterogeneous nonlinear hyperelasticity exemplify the massively parallel potential of the FE2 multiscale method.

This is a preview of subscription content, log in via an institution to check access.

Access this chapter

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Chapter
USD 29.95
Price excludes VAT (USA)
  • Available as PDF
  • Read on any device
  • Instant download
  • Own it forever

Tax calculation will be finalised at checkout

Purchases are for personal use only

Institutional subscriptions

Similar content being viewed by others

Notes

  1. 1.

    http://www.fz-juelich.de/ias/jsc/EN/Expertise/High-Q-Club/FE2TI/_node.html

References

  1. Amestoy, P.R., Duff, I.S., Koster, J., L’Excellent, J.Y.: A fully asynchronous multifrontal solver using distributed dynamic scheduling. SIAM J. Matrix Anal. Appl. 23 (1), 15–41 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  2. Amestoy, P.R., Guermouche, A., L’Excellent, J.Y., Pralet, S.: Hybrid scheduling for the parallel solution of linear systems. Parallel Comput. 32 (2), 136–156 (2006)

    Article  MathSciNet  Google Scholar 

  3. Balay, S., Brown, J., Buschelman, K., Gropp, W.D., Kaushik, D., Knepley, M.G., McInnes, L.C., Smith, B.F., Zhang, H.: PETSc Web page. http://www.mcs.anl.gov/petsc (2014)

  4. Balzani, D., Gandhi, A., Tanaka, M., Schröder, J.: Numerical calculation of thermo-mechanical problems at large strains based on complex step derivative approximation of tangent stiffness matrices. Comput. Mech. 55, 861–871 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  5. Balzani, D., Scheunemann, L., Brands, D., Schröder, J.: Construction of two- and three-dimensional statistically similar RVEs for coupled micro-macro simulations. Comput. Mech. 54, 1269–1284 (2014)

    Article  MATH  Google Scholar 

  6. Davis, T.A.: Direct Methods for Sparse Linear Systems. SIAM, Philadelphia (2006)

    Book  MATH  Google Scholar 

  7. Falgout, R.D., Jones, J.E., Yang, U.M.: The design and implementation of hypre, a library of parallel high performance preconditioners. In: Bruaset, A.M., Bjorstad, P., Tveito, A. (eds.) Numerical Solution of Partial Differential Equations on Parallel Computers. Lecture Notes in Computational Science and Engineering, vol. 51, pp. 267–294. Springer, Berlin (2006). http://dx.doi.org/10.1007/3-540-31619-1_8

    Chapter  Google Scholar 

  8. Farhat, C., Lesoinne, M., LeTallec, P., Pierson, K., Rixen, D.: FETI-DP: a dual-primal unified FETI method – part I: a faster alternative to the two-level FETI method. Int. J. Numer. Methods Eng. 50, 1523–1544 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  9. Feyel, F., Chaboche, J.: Fe2 multiscale approach for modelling the elastoviscoplastic behaviour of long fibre SiC/Ti composite materials. Comput. Methods Appl. Mech. Eng. 183, 309–330 (2000)

    Article  MATH  Google Scholar 

  10. Fish, J., Shek, K.: Finite deformation plasticity for composite structures: computational models and adaptive strategies. Comput. Methods Appl. Mech. Eng. 172, 145–174 (1999)

    Article  MATH  Google Scholar 

  11. Geers, M., Kouznetsova, V., Brekelmans, W.: Multi-scale first-order and second-order computational homogenization of microstructures towards continua. Int. J. Multiscale Comput. 1, 371–386 (2003)

    Article  Google Scholar 

  12. Golanski, D., Terada, K., Kikuchi, N.: Macro and micro scale modeling of thermal residual stresses in metal matrix composite surface layers by the homogenization method. Comput. Mech. 19, 188–201 (1997)

    Article  Google Scholar 

  13. Griewank, A., Walther, A.: Evaluating Derivatives. Society for Industrial and Applied Mathematics, 2nd edn. (2008). http://epubs.siam.org/doi/abs/10.1137/1.9780898717761

  14. Henson, V.E., Yang, U.M.: BoomerAMG: a parallel algebraic multigrid solver and preconditioner. Appl. Numer. Math. 41, 155–177 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  15. Holzapfel, G.A.: Nonlinear Solid Mechanics. A Continuum Approach for Engineering. John Wiley and Sons, Chichester (2000). http://opac.inria.fr/record=b1132727

    MATH  Google Scholar 

  16. Klawonn, A., Rheinbach, O.: Robust FETI-DP methods for heterogeneous three dimensional elasticity problems. Comput. Methods Appl. Mech. Eng. 196 (8), 1400–1414 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  17. Klawonn, A., Rheinbach, O.: Highly scalable parallel domain decomposition methods with an application to biomechanics. ZAMM Z. Angew. Math. Mech. 90 (1), 5–32 (2010). http://dx.doi.org/10.1002/zamm.200900329

    Article  MathSciNet  MATH  Google Scholar 

  18. Klawonn, A., Widlund, O.B.: Dual-primal FETI methods for linear elasticity. Commun. Pure Appl. Math. 59 (11), 1523–1572 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  19. Klawonn, A., Lanser, M., Rheinbach, O.: EXASTEEL – computational scale bridging using a FE2TI approach with ex_nl/FE2. Technical report FZJ-JSC-IB-2015-01, Jülich Supercomputing Center, Germany (2015). https://juser.fz-juelich.de/record/188191/files/FZJ-2015-01645.pdf. In: Frings, Brian J.N. Wylie (eds.) JUQUEEN Extreme Scaling Workshop 2015. Dirk Brömmel and Wolfgang

  20. Klawonn, A., Lanser, M., Rheinbach, O.: FE2TI: Computational Scale Bridging for Dual-Phase Steels (2015). Accepted for publication to the proceedings of the 16th ParCo Conference, Edinburgh. To be published in Advances in Parallel Computing

    Google Scholar 

  21. Klawonn, A., Lanser, M., Rheinbach, O.: Towards extremely scalable nonlinear domain decomposition methods for elliptic partial differential equations. SIAM J. Sci. Comput. 37 (6), C667–C696 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  22. Miehe, C.: Numerical computation of algorithmic (consistent) tangent moduli in large-strain computational inelasticity. Comput. Methods Appl. Mech. Eng. 134, 223–240 (1996)

    Article  MathSciNet  MATH  Google Scholar 

  23. Miehe, C., Schröder, J., Schotte, J.: Computational homogenization analysis in finite plasticity. simulation of texture development in polycrystalline materials. Comput. Methods Appl. Mech. Eng. 171, 387–418 (1999)

    Google Scholar 

  24. Moulinec, H., Suquet, P.: A numerical method for computing the overall response of nonlinear composites with complex microstructure. Comput. Methods Appl. Mech. Eng. 157, 69–94 (1998)

    Article  MathSciNet  MATH  Google Scholar 

  25. Pérez-Foguet, A., Rodríguez-Ferran, A., Huerta, A.: Numerical differentiation for local and global tangent operators in computational plasticity. Comput. Methods Appl. Mech. Eng. 189, 277–296 (2000)

    Article  MATH  Google Scholar 

  26. Schenk, O., Gärtner, K.: Two-level dynamic scheduling in PARDISO: improved scalability on shared memory multiprocessing systems. Parallel Comput. 28 (2), 187–197 (2002)

    Article  MATH  Google Scholar 

  27. Schröder, J.: A numerical two-scale homogenization scheme: the FE2-method. In: J. Schröder, K. Hackl (eds.) Plasticity and Beyond – Microstructures, Crystal-Plasticity and Phase Transitions. CISM Lecture Notes 550. Springer, Wien (2013)

    Google Scholar 

  28. Simo, J., Miehe, C.: Associative coupled thermoplasticity at finite strains: formulations, numerical analysis and implementation. Comput. Methods Appl. Mech. Eng. 98, 41–104 (1992)

    Article  MATH  Google Scholar 

  29. Smit, R., Brekelmans, W., Meijer, H.: Prediction of the mechanical behavior of nonlinear heterogeneous systems by multi-level finite element modeling. Comput. Methods Appl. Mech. Eng. 155, 181–192 (1998)

    Article  MATH  Google Scholar 

  30. Stephan, M., Docter, J.: JUQUEEN: IBM Blue Gene/QⓇ Supercomputer System at the Jülich Supercomputing Centre. JLSRF 1, A1. http://dx.doi.org/10.17815/jlsrf-1-18 (2015)

  31. Tanaka, M., Fujikawa, M., Balzani, D., Schröder, J.: Robust numerical calculation of tangent moduli at finite strains based on complex-step derivative approximation and its application to localization analysis. Comput. Methods Appl. Mech. Eng. 269, 454–470 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  32. The HDF Group: Hierarchical Data Format, version 5. http://www.hdfgroup.org/HDF5/ (1997-NNNN)

  33. Wriggers, P., Miehe, C., Kleiber, M., Simo, J.: On the coupled thermomechanical treatment of necking problems via finite element methods. Int. J. Numer. Methods Eng. 33, 869–883 (1992)

    Article  MATH  Google Scholar 

  34. Zienkiewicz, O.C., Taylor, R.L.: The Finite Element Method for Solid and Structural Mechanics. Elsevier, Oxford (2005)

    MATH  Google Scholar 

Download references

Acknowledgements

This work was supported by the German Research Foundation (DFG) through the Priority Program 1648 “Software for Exascale Computing” (SPPEXA ), projects BA 2823/8-1, KL 2094/4-1, RH 122/2-1, and SCHR 570/19-1.

The authors gratefully acknowledge the Gauss Centre for Supercomputing (GCS) for providing computing time through the John von Neumann Institute for Computing (NIC) on the GCS share of the supercomputer JUQUEEN [30] at Jülich Supercomputing Centre (JSC). GCS is the alliance of the three national supercomputing centres HLRS (Universität Stuttgart), JSC (Forschungszentrum Jülich), and LRZ (Bayerische Akademie der Wissenschaften), funded by the German Federal Ministry of Education and Research (BMBF) and the German State Ministries for Research of Baden-Württemberg (MWK), Bayern (StMWFK) and Nordrhein-Westfalen (MIWF).

The use of CHEOPS at Universität zu Köln and of the High Performance Cluster at Technische Universität Bergakademie Freiberg are also gratefully acknowledged. Furthermore, the authors D. Balzani and A. Gandhi appreciate S. Prüger for helpful scientific discussions.

Author information

Authors and Affiliations

  1. Faculty of Civil Engineering, Institute of Mechanics and Shell Structures, TU Dresden, Dresden, Germany

    Daniel Balzani & Ashutosh Gandhi

  2. Mathematisches Institut, Universität zu Köln, Köln, Germany

    Axel Klawonn & Martin Lanser

  3. Institut für Numerische Mathematik und Optimierung, Technische Universität Bergakademie Freiberg, Freiberg, Germany

    Oliver Rheinbach

  4. Faculty of Engineering, Department of Civil Engineering, Institute of Mechanics, Universität Duisburg-Essen, Essen, Germany

    Jörg Schröder

Authors
  1. Daniel Balzani
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Ashutosh Gandhi
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Axel Klawonn
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Martin Lanser
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Oliver Rheinbach
    View author publications

    You can also search for this author in PubMed Google Scholar

  6. Jörg Schröder
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding authors

Correspondence to Daniel Balzani , Ashutosh Gandhi , Axel Klawonn , Martin Lanser , Oliver Rheinbach or Jörg Schröder .

Editor information

Editors and Affiliations

  1. Technische Universität München Institut für Informatik, Garching, Bayern, Germany

    Hans-Joachim Bungartz

  2. Technische Universität München Institut für Informatik, Garching, Germany

    Philipp Neumann

  3. Technische Universität Dresden, Dresden, Germany

    Wolfgang E. Nagel

Rights and permissions

Reprints and permissions

Copyright information

© 2016 Springer International Publishing Switzerland

About this paper

Cite this paper

Balzani, D., Gandhi, A., Klawonn, A., Lanser, M., Rheinbach, O., Schröder, J. (2016). One-Way and Fully-Coupled FE2 Methods for Heterogeneous Elasticity and Plasticity Problems: Parallel Scalability and an Application to Thermo-Elastoplasticity of Dual-Phase Steels. In: Bungartz, HJ., Neumann, P., Nagel, W. (eds) Software for Exascale Computing - SPPEXA 2013-2015. Lecture Notes in Computational Science and Engineering, vol 113. Springer, Cham. https://doi.org/10.1007/978-3-319-40528-5_5

Download citation

Publish with us

Policies and ethics