Abstract
This article discusses the implementation of a large scale way of urban waste management in terms of its non-aggressive and productive destination, at low energy costs and with high reuse of byproducts. Aiming at the processing of medical waste, but not excluding other types of residues, present work is primarily focused on the so-called infectious waste, because they have greater virulence, infectivity and concentration, and wastes of type “skin-scissoring-piercing”, which are objects and instruments containing corners, edges or rigid and acute protuberances capable of cutting or drilling. In this paper, it is proposed an industrial solar system to recycling medical waste based on pyrolysis induced by plasma and controlled entropy.
You have full access to this open access chapter, Download conference paper PDF
Similar content being viewed by others
Keywords
1 Introduction
One of the most discussed topics at the tables of environmentalists and ecologists is the solid waste management (SWM). The SWM consists of a set of control procedures, planned and implemented from scientific, technical, regulatory and legal principles to minimize the production of waste and the risks involved in its disposal, and protecting workers and preserving public health, natural resources and the environment in general, at low cost [1]. The implementation of a solid waste management system requires skilled professionals, being essential for the preservation of life quality [2]. It should be noted here the urgency to banning land common in Latin America and other regions of the Third World [1, 3]. This article proposes a possible thermodynamic engineering system for large-scale solid waste eradication based on solar pyrolysis. In present work, the adopted definition of solid waste is provided by the Ministry of Health Brazil [1, 4].
2 Current Situation and the State of the Art
2.1 Hygiene and Sanitation: Weaknesses of the Global World
The dangers of exposure to medical waste are known from very early, although little is heard of it. Current literature indicates that direct and indirect exposure to hazardous waste includes, among many others, carcinogenic and reproductive system damage [4,5,6]. In Brazil, the indifference of the governmental authorities is high. Only in 2000, it was estimated that 76% of the Brazilian cities disposed of domestic and medical wastes together in public landfills. Despite the federal resolutions from ANVISA-2004 (Brazilian Sanitary Surveillance National Agency) and CONAMA-2005 (Brazilian Environmental National Council), requiring the management of waste generated by all healthcare units, medical waste is still being carried on landfills without treatment [1]. Brazilian law is mandatory when it claims the implementation of a medical waste management plan (MWMP), a subset of SWM, to be applied in all medical establishments, but they recognize that such plan is far from the real practice [1, 2].
2.2 The Risks of Conventional Incineration of Medical Waste
Incineration and disposal of its ash scraps by land filling are the most important available procedures to treat and transport medical waste and solid waste in general [7]. The conventional incineration leads to polluting the environment when not treated properly, with molecules that accumulate in the food chain such as dioxins, furans and coplanar polychlorinated biphenyls [3]. Many attempts have been made to perform locally medical waste treatment [3, 4] but failed regarding safety in the process of waste loading and the treatment of emissions. The only valid form to optimize the process and to reduce the formation of these substances is to apply temperatures far above 800 \(^\circ \mathrm{{C}}\), preventing the formation of effluent gases at temperatures within the range of 200 \(^\circ \mathrm{{C}}\) to 400 \(^\circ \mathrm{{C}}\) that occurs in combustion [3]. Only by atomic disruption we could control the ultimate condition of such materials, recombine atoms in ways that make inert substances.
When a gas is heated to high temperatures, there are significant changes in its properties. At about 2,000 \(^\circ \mathrm{{C}}\), the gas molecules begin to dissociate in atomic level. At 3,000 \(^\circ \mathrm{{C}}\), the atoms are ionized by the loss of electrons. This ionized gas is called plasma. In certain conditions, the plasma may induce a pyrolysis. Pyrolysis has been getting attention for many years because of its potential to produce biofuels from organic waste [8]. For our purposes, pyrolysis can be broadly defined as the chemical decomposition of the matter by heat in the absence of oxygen. The idea is to develop an engine dedicated to the disruption of waste, which associates the high temperatures of the plasma with the pyrolysis of the waste.
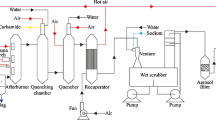
Pyrolysis applied to medical waste is not new [8, 9]. The rest format of pyrolytic gasification introduced commercially in the early 1970’s was the so-called batch-by-batch system which got a certain success to hospital process waste until the early 1980’s. Some problems arose, and one of them was how to maintain continuously throughout the waste. Finally, from a 50 ton-per-day pilot plant, the Balboa Pacific Co. settled an advanced pyrolytic thermal conversion system with a proprietary thermal waste conversion equipment to eliminate organic wastes [10]. What is new in present work is the application in large scale of pyrolysis induced by plasma for nosocomial solid and liquid, organic or not, waste elimination based on the use of solar energy and not electricity. Even though the overall process is cleaner in comparison to conventional burning, there are evolved products which require special controls [9]. Figure 1 shows the fundamental proposed framework from which we shall discuss how we can minimize the problem of the generation of undesirable substances.
The complete scheme of the proposed thermodynamic engine. Note the cicle of energy with a thermoelectric station feeding continually the usine as well as it is powered by solar energy (from Serpa’s Ph.D. thesis in French [11]).
3 The Methods
Solar concentrators have become a reality in the day-to-day response to sanitary, and environmental preservation needs. Our proposed plant unifies fundamental issues in a comprehensive approach to thermal systems engineering. Virtually any material can be carried to the pyrolysis chamber. It is assumed that the preconditioning chamber gets material properly handled and encapsulated. Also, it is reasonable to believe that rubber, plastic, gauze, paper and cotton average out 90% of total medical waste [3, 12]. The waste fills (preconditioning chamber) is connected to a cylindrical graphite chamber. From the preconditioning chamber, we feed the graphite chamber in which pyrolysis shall take place. Internally subjected to a vacuum, this last receives the concentrated sunlight rays from a concave array of mirrors on a quartz window placed at one of the circular bases of the chamber. At high temperatures atomic disruptions produce gasses and liquids that go into the recycling device, inside which the gradient of temperatures \(T_1, T_2, T_3, T_4,...,T_n\) allows to a recovery of products \(P_1, P_2, P_3, P_4,...,P_n\) from the hottest layers to the cooler. In the Operations sector, a computational control system conducts catalytic agents, whose actions enter the processes associated with temperatures to ensure the outputs of programmed materials, and the recombination of remnant atoms into inert substances in the form of usable waste. To reduce the entropy and expand the productivity of the heat generation we introduced an auxiliary piping system for the laminar flow of a nanofluid to establish a convection process of heat transfer [11]. Lastly, products, final residues and usable waste are sent respectively to inventory and appropriate containment, remembering that the so called “pyrolysis ashes” – similar to the dust and blast furnace sludge – which constitute the usable waste can be used in the cement industry. All the energy needed to run the engine is solar, being the possible surplus routed to the public network.
From the hottest layers to the cooler. In the Operations sector, a computational control system conducts catalytic agents, whose actions enter the processes associated with temperatures to ensure the outputs of materials in the form of usable waste. To reduce the entropy and expand the productivity of the heat generation we introduced an auxiliary piping system for the laminar ow of a nanofluid to establish a convection process of heat transfer [11]. The theory and its application to the power platform the econophysics foundations to match operations management and environmental management in a unified operational level [8] to include the waste management supply chain.
Accordingly classical theory, the present model supposes a differential polynomial in \(\xi \), the Lagrangian density \(\mathcal {L}(\xi )\), given by
whose action over a certain region \(\mathcal {D}\) in space and time is
Here, from Serpa’s first proposal, \(\xi \) represents a scalar complex massless caloric field, \(d\mathcal {V}\) an infinitesimal volumn of space, dt an infinitesimal time interval, and \(\gamma \) a real scalar to be defined later which depends on the system’s environment in question [11]. The caloric field obeys the field equation
being the field entropy in generalized coordinates q given by
Thus, field equation includes an entropy term \(- 2{\gamma ^2}\xi \ln {\left| \xi \right| }\) in the dynamics of the field and expression (4) is just a straightforward generalization of Gibbs entropy. It is worth noting that for \(\left| \xi \right| ^2 < 1\) it follows that \(2\ln \left| \xi \right| < 0\); thus, \(S>0\) for every non-trivial system state. The factor \(\left( {1 - {\gamma ^2}} \right) \) in the second term of Eq. (3), the so-called “luminothermic capacity”, reflects the potential power offered by the natural surroundings. Its action under the field shows how field is influenced by the external conditions. Thus, caloric field equation governs the evolution of the thermal energy field and the corresponding entropy produced.
Regarding the efficiency of te engine the logical way to establish an asymptotic relation between the proposed heat engine efficiency and the Carnot machine efficiency is to introduce the irreversible coefficient \(\varphi \), due to the irreversible events during the industry processes. So efficiency can be written as
where \(T_\mathrm{{I}}\) is the temperature associated with the initial state of the system (at which the waste enters the pyrolysis chamber) and \(T_\mathrm{{II}}\) is the temperature associated with the final state of the system (time at which the recycling loop is completed). The heat engine efficiency may be compared to luminothermic capacity in order to measure the relation between the contribution of the environment and the technology of reduction of irreversible outputs. However, this comparison requires empirical results to be recorded and analyzed. Anyway, even if the plant operated very close to a Carnot machine, the efficiency would be limited by the maximum luminothermic capacity allowed by the Earth environments. In addition, while all the actual industry processes are irreversible, \(\varphi \) can only asymptotically approach unit with the minimization of irreversible events. Thereby, the more \(\varphi \) is closer to 1, the less is the number of irreversible processes and the entropy variation \(\varDelta S\); the production becomes cleaner [10].
4 The Plant in Process and the Expected Results
The chosen area for the plant, with eight hectares, is about two km from the large dumpsite of Brasilia, Brazil. The design of the facility, solar concentrator uses linear Fresnel reflectors, that is, individually designed mirrors mounted on two-axis tracking devices, with very similar features in arrangement and operation to the conventional parabolic collectors. It is placed fifteen meters underground (beneath the soil level).
TFirst computational simulations of the concentrator proved capacity to achieve temperatures close to 1588 \(^\circ \mathrm{{C}}\) inside the pyrolysis chamber, on the contrary of the great majority of most industrial heating processes which run below 300 \(^\circ \mathrm{{C}}\). Also, these simulations pointed to an efficiency of the plant between 68,44% and 72,82%. These outcomes are very encouraging in the sense that several persistent pollutants shall be avoided since the achieved temperature is far above 800 \(^\circ \mathrm{{C}}\) and much higher than the temperatures of formation of effluent gases, typically at 200–400 \(^\circ \mathrm{{C}}\). The pyrolysis chamber (the receiver) is a hollow soft carbon cylindrical container —- with initial capacity for 1/2 ton of wastes —- placed east on the ground (soil level). Sunbeam is reflected and concentrated on the back of the chamber (quartz window), and the temperature gradient of the inner caloric field is controlled by thermal exchangers along the chamber. In fact, some improvements in the heat exchanger effectiveness for setting the temperature gradient were attained in simulations using vanes, whose positive influence on heat exchange in industrial air heaters was demonstrated by Atkins et al. for the range of air-side Reynolds numbers [13]. In addition, the heat transfer nanofluid circulates through the pyrolysis chamber, collecting and transporting thermal energy to storage units and power production for the Operations sector in a continuous process of feedback. Indeed, the complete plant shall include another identical soft carbon pyrolysis chamber placed west also on the ground (Fig. 2), so that we shall have one array of mirrors for each chamber. Since the receivers are separate units, the tracking is simple and efficient. As explained previously, caloric field inside the chambers is governed by field Eq. (3), where the constant \(\gamma \) in the second term and in the term of entropy defines the degree of obfuscation of the environment; we call it “blurring” or “opacity”. Opacity depends on some environmental conditions such as the amount of dust in the air, industrial pollution and the local propensity to cloud accumulation. We defined five levels of blurring to be considered: very low, low, moderate, high and very high (Table 1). Braslia was classified having moderate blurring (\(\gamma = 0.6882\)) despite the high annual insolation because of the frequent wind dust spreading in Brazilian Central Plateau from August to October and the intermittent formation of clouds from December to March. The values found in Table 1 were established after observations conducted on the environmental luminosity in different seasons and times at distinct Brazilian localities. Also, the investigation was made considering the estimated daily average density of dust accumulated on the set of reflectors from the expression
where \({\delta _M }\) is the total mass of dust deposition on the surface of the reflector, A is the reflector surface area, i is a counter of reflectors, and j is a counter of days (in present case, from August to October, the period considered as the peak interval of the wind regime during dry season). Measurements were made on site using common mirrors placed at positions provided for reflectors. For each daily measurement, mirrors were well cleaned to remove the mass of dust. Anyway, the shading caused by dust deposits shall also be counteracted with a minimum inclination of mirrors around 12\(^o\) [7].
In the chambers of pyrolysis and recycling, it is necessary that there is a polytropic process, i.e., an intermediate process between an isothermal process and an adiabatic process. In this case, the polytropic index n is equal to 2 for reasons of analytical symmetry and thermodynamical optimization [11]. Suppose a caloric field described by
where the quantity \(\vartheta \) is the theoretical refractive index of the quartz window. One can easily demonstrate that field Eq. (3) gives
From this result, we can establish the values shown in Table 1, where the quartz refractive index is achieved with good approximation to the levels of high and moderate opacity.
5 Conclusion
The system was conceived and prepared to operate at high precision to minimize entropy production, so that an accurate physical analysis was performed. Nevertheless, it would be impossible to make extensive mathematical deductions in this paper, reason why we restrict ourselves to report that all the physical process is summarized in three fundamental equations as follows:
-
1.
About the diffusion model: the combined equation Lane-Emden/Langmuir for \(n = 2\) [11],
$$\begin{aligned} - \frac{1}{{{x^2}}}\left( {2{} x{} \frac{d}{{dx}}y\left( x \right) + {x^2}\frac{{{d^2}}}{{d{x^2}}}y\left( x \right) } \right) + 3{} y\left( x \right) \frac{{{d^2}}}{{d{x^2}}}y\left( x \right) + {\left( {\frac{d}{{dx}}y\left( x \right) } \right) ^2} \end{aligned}$$(9)$$\begin{aligned} + 4{} y\left( x \right) \frac{d}{{dx}}y\left( x \right) - 1 = 0; \end{aligned}$$(10)this equation describes the evolution of the dimensionless caloric density y on a conductive cylindrical symmetry, keeping the density relatively stable over a longer radius measured by x; it serves to establish a curve which defines a useful level of energy balance.
-
2.
About the relationship between the thermodynamic variables S,
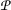 , T and V (entropy, pressure, temperature and volume): Serpa’s equation in partial derivatives [11], $$\begin{aligned} \tilde{c} {{\partial ^2 V} \over {\partial S\partial P}} = -{1 \over P}{{\partial T} \over {\partial P}}; \end{aligned}$$(11)
, T and V (entropy, pressure, temperature and volume): Serpa’s equation in partial derivatives [11], $$\begin{aligned} \tilde{c} {{\partial ^2 V} \over {\partial S\partial P}} = -{1 \over P}{{\partial T} \over {\partial P}}; \end{aligned}$$(11)this second-order equation, with the parameter \(\tilde{c} > 0\) corresponding to the polytropic index (n), describes the variation in volume due to entropy and pressure (assuming
 parameterized with respect to S).
parameterized with respect to S). -
3.
About the control of system’s entropy: Serpa’s caloric field Eq. [11],
$$\begin{aligned} {\partial _q}{\partial ^q}\xi + \left( {1 - {\gamma ^2}} \right) \xi - {\gamma ^2}\xi \ln {\left| \xi \right| ^2} = 0; \end{aligned}$$(12)that is, by definition, the equation that governs the field inside the pyrolysis reactor.
These three equations are crucial to the construction of the production control algorithm to run up in the sector “Operations”. The reader should note that these equations have in common the polytropic index (Table 1) was constructed from this base) because it is always possible to give field \(\xi \) parameterized by n. It is also important to note that all we can do is to work on systems with a good approximation for \(n = 2\).
References
Ministry of Health: Technical Guidelines for Preparation of the Program of Health Education and Social Mobilization. ASCOM/FUNASA, Brasília, Brazil (2004)
ICRC: Medical Waste Management. Geneva, Switzerland (2011)
Ananth, P., Prashanthini, V., Visvanathan, C.: Healthcare waste management in Asia. Waste Manag. 30, 154–161 (2010)
Moreira, A., Günther, W.: Assessment of medical waste management at a primary health-care center in São Paulo - Brazil. Waste Manag. 33, 162–167 (2013)
Tsakona, M., Anagnostopoulou, E., Gidarakos, E.: Hospital waste management and toxicity evaluation: a case study. Waste Manag. 27, 912–920 (2007)
Franka, E., El-Zoka, A., Hussein, A., Elbakosh, M., Arafa, A., Ghenghesh, K.: Hepatitis B virus and hepatitis C virus in medical waste handlers in Tripoli - Libya. J. Hosp. Infect. 72, 258–261 (2009)
El-Shobokshy, M., Hussein, F.: Degradation of photovoltaic cell performance due to dust deposition on to its surface. Renew. Energy 3(6/7), 585–590 (1993)
Deng, N., Zhang, Y., Wang, Y.: Thermogravimetric analysis and kinetic study on pyrolysis of representative medical waste composition. Waste Manag. 28, 1572–1580 (2008)
Zhu, H., Yan, J., Jiang, X., Lai, Y., Cen, K.: Study on pyrolysis of typical medical waste materials by using TG-FTIR analysis. J. Hazard. Mater. 153, 670–676 (2008)
Balboa Pacic Corporation: From Waste to Electricity — A Pyrolysis Technology. Advanced Thermal Conversion Technologies, San Diego, California (2014)
Serpa, N.: Sur l’entropie contrôlée des systèmes – le traitement des déchets médicaux et hospitaliers ou une nouvelle approche de la production plus nette basée. Ph.D. thesis, L’Universit Libre des Sciences de L’Homme de Paris, Sorbonne (2014)
Xiea, R., Li, W., Li, J., Wu, B., Yi, J.: Emissions investigation for a novel medical waste incinerator. J. Hazard. Mater. 166, 365–371 (2009)
Atkins, M., Neale, J., Walmsley, M., Walmsley, T., de Leon, G.: Flow maldistribution in industrial air heaters and its effect on heat transfer. Chem. Eng. Trans. 39, 295–300 (2014)
Author information
Authors and Affiliations
Corresponding author
Editor information
Editors and Affiliations
Rights and permissions
Copyright information
© 2016 IFIP International Federation for Information Processing
About this paper
Cite this paper
Serpa, N., Costa, I., Gonçalves, R.F. (2016). A Thermal System Based on Controlled Entropy for Treatment of Medical Waste by Solar Energy. In: Nääs, I., et al. Advances in Production Management Systems. Initiatives for a Sustainable World. APMS 2016. IFIP Advances in Information and Communication Technology, vol 488. Springer, Cham. https://doi.org/10.1007/978-3-319-51133-7_92
Download citation
DOI: https://doi.org/10.1007/978-3-319-51133-7_92
Published:
Publisher Name: Springer, Cham
Print ISBN: 978-3-319-51132-0
Online ISBN: 978-3-319-51133-7
eBook Packages: Computer ScienceComputer Science (R0)






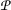 , T and V (entropy, pressure, temperature and volume): Serpa’s equation in partial derivatives [
, T and V (entropy, pressure, temperature and volume): Serpa’s equation in partial derivatives [ parameterized with respect to S).
parameterized with respect to S).