Skip to main content
Springer Nature Link
Log in

Optimal Embedding of Locally Twisted Cubes into Grids

  • Conference paper
  • First Online:
Algorithms and Discrete Applied Mathematics (CALDAM 2017)

Part of the book series: Lecture Notes in Computer Science ((LNTCS,volume 10156))

Included in the following conference series:

Abstract

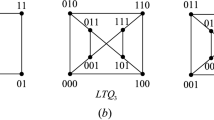
The hypercube has been used in numerous problems related to interconnection networks due to its simple structure and communication properties. The locally twisted cube is an important class of hypercube variants with the same number of nodes and connections per node, but has only half the diameter and better graph embedding capability as compared to its counterpart. The embedding problem plays a significant role in parallel and distributed systems. In this paper we devise an optimal embedding of the n-dimensional locally twisted cube onto a grid network.

This is a preview of subscription content, log in via an institution to check access.

Access this chapter

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Chapter
USD 29.95
Price excludes VAT (USA)
  • Available as PDF
  • Read on any device
  • Instant download
  • Own it forever

Tax calculation will be finalised at checkout

Purchases are for personal use only

Institutional subscriptions

Similar content being viewed by others

References

  1. Arockiaraj, M., Abraham, J., Quadras, J., Shalini, A.J.: Linear layout of locally twisted cubes. Int. J. Comput. Math. (2015). doi:10.1080/00207160.2015.1088943

    Google Scholar 

  2. Battista, G.D., Eades, P., Tamassia, R., Tollis, I.G.: Algorithms for drawing graphs: an annotated bibliography. Comput. Geom. 4, 235–282 (1994)

    Article  MathSciNet  MATH  Google Scholar 

  3. Bezrukov, S.L., Chavez, J.D., Harper, L.H., Röttger, M., Schroeder, U.-P.: The congestion of \(n\)-cube layout on a rectangular grid. Discret. Math. 213(1), 13–19 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  4. Bhatt, S.N., Leighton, F.T.: A framework for solving VLSI graph layout problems. J. Comp. Syst. Sci. 28, 300–343 (1984)

    Article  MathSciNet  MATH  Google Scholar 

  5. Djidjev, H.N., Vrto, I.: Crossing numbers and cutwidths. J. Graph Algorithms Appl. 7, 245–251 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  6. Han, H., Fan, J., Zhang, S., Yang, J., Qian, P.: Embedding meshes into locally twisted cubes. Inform. Sci. 180, 3794–3805 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  7. Harper, L.H.: Optimal assignments of numbers to vertices. J. Soc. Ind. Appl. Math. 12, 131–135 (1964)

    Article  MathSciNet  MATH  Google Scholar 

  8. Harper, L.H.: Global Methods for Combinatorial Isoperimetric Problems. Cambridge University Press, London (2004)

    Book  MATH  Google Scholar 

  9. Lai, Y.L., Williams, K.: A survey of solved problems and applications on bandwidth, edgesum, and profile of graphs. J. Graph Theory 31, 75–94 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  10. Manuel, P., Rajasingh, I., Rajan, B., Mercy, H.: Exact wirelength of hypercube on a grid. Discret. Appl. Math. 157(7), 1486–1495 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  11. Opatrny, J., Sotteau, D.: Embeddings of complete binary trees into grids and extended grids with total vertex-congestion 1. Discret. Appl. Math. 98, 237–254 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  12. Rajasingh, I., Arockiaraj, M.: Linear wirelength of folded hypercubes. Math. Comput. Sci. 5, 101–111 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  13. Rajasingh, I., Arockiaraj, M., Rajan, B., Manuel, P.: Minimum wirelength of hypercubes into n-dimensional grid networks. Inform. Process. Lett. 112, 583–586 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  14. Rajasingh, I., Rajan, R.S., Parthiban, N., Rajalaxmi, T.M.: Bothway embedding of circulant network into grid. J. Discret. Algorithms. 33, 2–9 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  15. Yang, X., Evans, D.J., Megson, G.M.: The locally twisted cubes. Int. J. Comput. Math. 82(4), 401–413 (2005)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Acknowledgement

This work was supported by Project No. 5LCTOI14MAT002, Loyola College - Times of India, Chennai, India.

Author information

Authors and Affiliations

  1. Department of Mathematics, Loyola College, Chennai, 600034, India

    Jessie Abraham & Micheal Arockiaraj

Authors
  1. Jessie Abraham
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Micheal Arockiaraj
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Jessie Abraham .

Editor information

Editors and Affiliations

  1. University of Lethbridge, Lethbridge, Alberta, Canada

    Daya Gaur

  2. Indian Institute of Technology Madras, Chennai, India

    N.S. Narayanaswamy

Rights and permissions

Reprints and permissions

Copyright information

© 2017 Springer International Publishing AG

About this paper

Cite this paper

Abraham, J., Arockiaraj, M. (2017). Optimal Embedding of Locally Twisted Cubes into Grids. In: Gaur, D., Narayanaswamy, N. (eds) Algorithms and Discrete Applied Mathematics. CALDAM 2017. Lecture Notes in Computer Science(), vol 10156. Springer, Cham. https://doi.org/10.1007/978-3-319-53007-9_1

Download citation

  • DOI: https://doi.org/10.1007/978-3-319-53007-9_1

  • Published:

  • Publisher Name: Springer, Cham

  • Print ISBN: 978-3-319-53006-2

  • Online ISBN: 978-3-319-53007-9

  • eBook Packages: Computer ScienceComputer Science (R0)

Publish with us

Policies and ethics