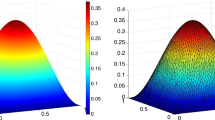
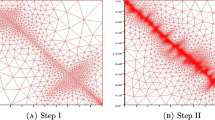
Abstract
In this paper we present a-posteriori error estimator for the mixed formulation of linear parabolic problem, and we use them in designing an efficient adaptive algorithm. Our space-time discretization consist of lowest order Raviart-Thomas finite element over graded meshes, and discontinuous Galerkin method with varying time-steps.
Chapter PDF
Similar content being viewed by others
References
Alonso, A.: Error estimators for a mixed method. Numer. Math. 74(4), 385–395 (1994)
Cartensen, C.: A posteriori error estimate for mixed finite element method. Math. Comp. 66(218), 465–776 (1997)
Eriksson, K., Johnson, C.: Adaptive Finite Element Methods For Parabolic Problems I: A Linear Model Problem. SIAM J. Numer. Anal. 28(1), 43–77 (1991)
Nochetto, R.H., Schmidt, A., Verdi, C.: A posterioi error estimation and adaptivity for degenerate parabolic problems. Math. Comp. 69(229), 1–24 (2000)
Schmidt, A., Siebert, K.G.: ALBERT: An adaptive hierarchical finite element toolbox, Preprint 06/2000, Freiburg (2000)
Author information
Authors and Affiliations
Editor information
Editors and Affiliations
Rights and permissions
Copyright information
© 2004 Springer-Verlag Berlin Heidelberg
About this paper
Cite this paper
Asensio, M.I., Cascón, J.M., Ferragut, L. (2004). A-Posteriori Error Analysis of a Mixed Method for Linear Parabolic Problem. In: Bubak, M., van Albada, G.D., Sloot, P.M.A., Dongarra, J. (eds) Computational Science - ICCS 2004. ICCS 2004. Lecture Notes in Computer Science, vol 3037. Springer, Berlin, Heidelberg. https://doi.org/10.1007/978-3-540-24687-9_78
Download citation
DOI: https://doi.org/10.1007/978-3-540-24687-9_78
Publisher Name: Springer, Berlin, Heidelberg
Print ISBN: 978-3-540-22115-9
Online ISBN: 978-3-540-24687-9
eBook Packages: Springer Book Archive