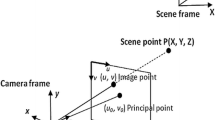
Abstract
Self-calibration for imaging sensors is essential to many computer vision applications. In this paper, a new stratified self-calibration method is proposed for a stereo rig undergoing planar motion with varying intrinsic parameters. We show that the plane at infinity in a projective frame can be identified by (i) a constraint developed from the properties of planar motion for a stereo rig and (ii) a zero-skew assumption of the camera. Once the plane at infinity is identified, the calibration matrices of the cameras and the upgrade to a metric reconstruction can be readily obtained. The proposed method is more flexible than most existing self-calibration methods in that it allows all intrinsic parameters to vary. Experimental results for both synthetic data and real images are provided to show the performance of the proposed method.
The work described in this paper is partially supported by a grant from the Research Grant Council of the Hong Kong Special Administrative Region, China (Project No. HKU 7058/02E) and partially supported by CRCG of The University of Hong Kong
Access this chapter
Tax calculation will be finalised at checkout
Purchases are for personal use only
Preview
Unable to display preview. Download preview PDF.
Similar content being viewed by others
References
Dornaika, F.: Self-calibration of a Stereo Rig using Monocular Epipolar Geometry. In: ICCV 2001. IEEE Top Reference, 0106 BibRef, pp. 467–472 (2001)
Knight, J., Reid, I.: Active visual alignment of a mobile stereo camera platform. In: Proc. International Conference of Robotics and Automation, San Francisco (2000)
Ruf, G.: Csurka, and R. Horaud. Projective Translations and Affine Stereo Calibration. In: Proceedings IEEE Conference on Computer Vision and Pattern Recognition, Santa Barbara, CA, pp. 475–481 (1998)
Zisserman, A., Beardsley, P.A., Reid, I.D.: Metric Calibration of a stereo rig. In: Proc. IEEE Workshop on Representations of Visual Scenes, Boston, pp. 93–100. IEEE Computer Society Press, Los Alamitos (1995)
Horaud, R., Caurka, G.: Self-calibration and Euclidean reconstruction using motions of a stereo rig. In: Proc. Of ICCV, pp. 96–103 (1998)
Knight, J., Reid, I.: Self-calibration of a stereo rig in a planar scene by data combination. In: ICPR, Barcelona, Spain, pp. 1411–1414 (2000)
Tang, W.K., Hung, Y.S.: A Factorization-based method for Projective Reconstruction with minimization of 2-D reprojection errors. In: Van Gool, L. (ed.) DAGM 2002. LNCS, vol. 2449, pp. 387–394. Springer, Heidelberg (2002)
Strum, P.: Camera Self-Calibration: A Case Against Kruppa’s Equations, ICIP, Chicago (1998)
Zisserman, A., et al.: Resolving Ambiguities in Auto-Calibration, Royal Society Typescript (1998)
Fitzgibbon, A.W., et al.: Automatic 3D Model Construction for Turn-Table Sequences. In: Koch, R., Van Gool, L. (eds.) SMILE 1998. LNCS, vol. 1506, pp. 155–170. Springer, Heidelberg (1998)
Seo, Y., Hong, K.S.: Theory and Practice on the Self-calibration of a Rotating and Zooming Camera from Two Views. VISP(148) (3), 166–172 (2001)
Hartley, R.I., Hayman, E., de Agapito, L., Reid, I.: Camera Calibration and the Search for Infinity. In: International Conference on Computer Vision, vol. 1, pp. 510–516 (1999)
Pollefeys, M., Gool, L.V.: Stratified self-Calibration with modulus constraint. IEEE Transactions on Pattern Analysis and Machine Intelligence 21(8), 707–724 (1999)
Dai, S., Ji, Q.: A New Technique for Camera Self-Calibration. In: International Conference on Robotics & Automation, Seoul, Korea, pp. 2165–2170 (2001)
Hartley, R., Zisserman, A.: Multiple View Geometry in Computer Vision. Cambridge University Press, UK (2000)
Author information
Authors and Affiliations
Editor information
Editors and Affiliations
Rights and permissions
Copyright information
© 2004 Springer-Verlag Berlin Heidelberg
About this paper
Cite this paper
Li, Y., Hung, Y.S. (2004). A Stratified Self-Calibration Method for a Stereo Rig in Planar Motion with Varying Intrinsic Parameters. In: Rasmussen, C.E., Bülthoff, H.H., Schölkopf, B., Giese, M.A. (eds) Pattern Recognition. DAGM 2004. Lecture Notes in Computer Science, vol 3175. Springer, Berlin, Heidelberg. https://doi.org/10.1007/978-3-540-28649-3_39
Download citation
DOI: https://doi.org/10.1007/978-3-540-28649-3_39
Publisher Name: Springer, Berlin, Heidelberg
Print ISBN: 978-3-540-22945-2
Online ISBN: 978-3-540-28649-3
eBook Packages: Springer Book Archive