Skip to main content
Springer Nature Link
Log in

HP Distance Via Double Cut and Join Distance

  • Conference paper
Combinatorial Pattern Matching (CPM 2008)

Part of the book series: Lecture Notes in Computer Science ((LNTCS,volume 5029))

Included in the following conference series:

Abstract

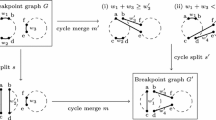
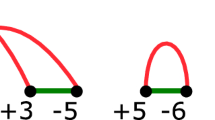
The genomic distance problem in the Hannenhalli-Pevzner theory is the following: Given two genomes whose chromosomes are linear, calculate the minimum number of inversions and translocations that transform one genome into the other. This paper presents a new distance formula based on a simple tree structure that captures all the delicate features of this problem in a unifying way.

This is a preview of subscription content, log in via an institution to check access.

Access this chapter

Log in via an institution

Institutional subscriptions

Preview

Unable to display preview. Download preview PDF.

Unable to display preview. Download preview PDF.

Similar content being viewed by others

References

  1. Bergeron, A.: A very elementary presentation of the hannenhalli-pevzner theory. In: Amir, A., Landau, G.M. (eds.) CPM 2001. LNCS, vol. 2089, pp. 106–117. Springer, Heidelberg (2001)

    Google Scholar 

  2. Bergeron, A., Mixtacki, J., Stoye, J.: Reversal distance without hurdles and fortresses. In: Sahinalp, S.C., Muthukrishnan, S.M., Dogrusoz, U. (eds.) CPM 2004. LNCS, vol. 3109, pp. 388–399. Springer, Heidelberg (2004)

    Google Scholar 

  3. Bergeron, A., Mixtacki, J., Stoye, J.: On sorting by translocations. J. Comput. Biol. 13(2), 567–578 (2006)

    Article  MathSciNet  Google Scholar 

  4. Bergeron, A., Mixtacki, J., Stoye, J.: A unifying view of genome rearrangements. In: Bücher, P., Moret, B.M.E. (eds.) WABI 2006. LNCS (LNBI), vol. 4175, pp. 163–173. Springer, Heidelberg (2006)

    Chapter  Google Scholar 

  5. Bergeron, A., Stoye, J.: On the similarity of sets of permutations and its applications to genome comparison. In: Warnow, T., Zhu, B. (eds.) COCOON 2003. LNCS, vol. 2697, pp. 68–79. Springer, Heidelberg (2003)

    Chapter  Google Scholar 

  6. Hannenhalli, S., Pevzner, P.A.: Transforming men into mice (polynomial algorithm for genomic distance problem). In: Proceedings of FOCS 1995, pp. 581–592. IEEE Press, Los Alamitos (1995)

    Google Scholar 

  7. Jean, G., Nikolski, M.: Genome rearrangements: a correct algorithm for optimal capping. Inf. Process. Lett. 104, 14–20 (2007)

    Article  MathSciNet  Google Scholar 

  8. Ozery-Flato, M., Shamir, R.: Two notes on genome rearrangements. J. Bioinf. Comput. Biol. 1(1), 71–94 (2003)

    Article  Google Scholar 

  9. Tesler, G.: Efficient algorithms for multichromosomal genome rearrangements. J. Comput. Syst. Sci. 65(3), 587–609 (2002)

    Article  MATH  MathSciNet  Google Scholar 

  10. Tesler, G.: GRIMM: Genome rearrangements web server. Bioinformatics 18(3), 492–493 (2002)

    Article  MathSciNet  Google Scholar 

  11. Yancopoulos, S., Attie, O., Friedberg, R.: Efficient sorting of genomic permutations by translocation, inversion and block interchange. Bioinformatics 21(16), 3340–3346 (2005)

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Dépt. d’informatique, Université du Québec à Montréal, Canada

    Anne Bergeron

  2. International NRW Graduate School in Bioinformatics and Genome Research, Universität Bielefeld, Germany

    Julia Mixtacki

  3. Technische Fakultät, Universität Bielefeld, Germany

    Jens Stoye

Authors
  1. Anne Bergeron
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Julia Mixtacki
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Jens Stoye
    View author publications

    You can also search for this author in PubMed Google Scholar

Editor information

Paolo Ferragina Gad M. Landau

Rights and permissions

Reprints and permissions

Copyright information

© 2008 Springer-Verlag Berlin Heidelberg

About this paper

Cite this paper

Bergeron, A., Mixtacki, J., Stoye, J. (2008). HP Distance Via Double Cut and Join Distance. In: Ferragina, P., Landau, G.M. (eds) Combinatorial Pattern Matching. CPM 2008. Lecture Notes in Computer Science, vol 5029. Springer, Berlin, Heidelberg. https://doi.org/10.1007/978-3-540-69068-9_8

Download citation

  • DOI: https://doi.org/10.1007/978-3-540-69068-9_8

  • Publisher Name: Springer, Berlin, Heidelberg

  • Print ISBN: 978-3-540-69066-5

  • Online ISBN: 978-3-540-69068-9

  • eBook Packages: Computer ScienceComputer Science (R0)

Publish with us

Policies and ethics