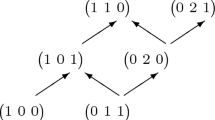
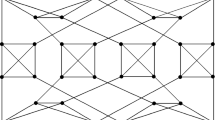
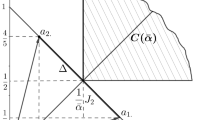
Abstract
Our paper is devoted to the computation of the weight spectra of Conway matrices related to the non-transitive head-or-tail game. We obtained explicit formulas for the spectra containing partial binomial sums. These sums are rather hard to deal with when the methods of classical algebra and number theory are used; but when we used methods of computer algebra, we were able to handle them quite efficiently and could easily produce visualizations. We suggest a recurrence algorithm for efficient calculation of the weight-spectrum matrices, including, as a special case, integer matrices modulo m. The algorithm is implemented with MATHEMATICA and visualizations for some interesting examples are shown.
This is a preview of subscription content, log in via an institution to check access.
Access this chapter
Tax calculation will be finalised at checkout
Purchases are for personal use only
Preview
Unable to display preview. Download preview PDF.
References
Gardner, M.: Time Travel and Other Mathematical Bewilderments. NY (1988)
Guibas, L.J., Odlyzko, A.M.: String Overlaps, Pattern Matching, and Nontransitive Games. Journal of Combinatorial Theory 30, 183–208 (1981)
MacWilliams, F.J., Sloane, N.J.A.: The Theory of Error-Correcting Codes. North-Holland P.C., Amsterdam (1977)
Gogin, N., Mylläri, A.: Choosing the Strategy in non-Transitive Games: Visualization (to be published)
Graham, R.L., Knuth, D.E., Patashnik, O.: Concrete Mathematics. Addison-Wesley, Reading (1989)
Ollerton, R.L.: Partial row-sums of Pascal’s triangle. International Journal of Mathematical Education in Science and Technology 38(1/15 ), 124–127 (2007)
Author information
Authors and Affiliations
Editor information
Rights and permissions
Copyright information
© 2007 Springer-Verlag Berlin Heidelberg
About this paper
Cite this paper
Gogin, N., Mylläri, A. (2007). On the Weight Spectra of Conway Matrices Related to the Non-transitive Head-or-Tail Game. In: Ganzha, V.G., Mayr, E.W., Vorozhtsov, E.V. (eds) Computer Algebra in Scientific Computing. CASC 2007. Lecture Notes in Computer Science, vol 4770. Springer, Berlin, Heidelberg. https://doi.org/10.1007/978-3-540-75187-8_13
Download citation
DOI: https://doi.org/10.1007/978-3-540-75187-8_13
Publisher Name: Springer, Berlin, Heidelberg
Print ISBN: 978-3-540-75186-1
Online ISBN: 978-3-540-75187-8
eBook Packages: Computer ScienceComputer Science (R0)