Computational Aspects of a 2-Player Stackelberg Shortest Paths Tree Game

Abstract

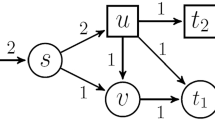
Let a communication network be modelled by a directed graph G = (V,E) of n nodes and m edges. We consider a one-round two-player network pricing game, the Stackelberg Shortest Paths Tree (StackSPT) game. This is played on G, by assuming that edges in E are partitioned into two sets: a set E _F of edges with a fixed positive real weight, and a set E _P of edges that should be priced by one of the two players (the leader). Given a distinguished node r ∈ V, the StackSPT game is then as follows: the leader prices the edges in E _P in such a way that he will maximize his revenue, knowing that the other player (the follower) will build a shortest paths tree of G rooted at r, say S(r), by running a publicly available algorithm. Quite naturally, for each edge selected in the solution, the leader’s revenue is assumed to be equal to the loaded price of an edge, namely the product of the edge price times the number of paths from r in S(r) that use it. First, we show that the problem of maximizing the leader’s revenue is NP-hard as soon as |E _P | = Θ(n). Then, in search of an effective method for solving the problem when the size of E _P is constant, we focus on the basic case in which |E _P | = 2, and we provide an efficient O(n ² logn) time algorithm. Afterwards, we generalize the approach to the case |E _P | = k, and we show that it can be solved in polynomial time whenever k = O(1).

Part of this work has been developed while the third author was visiting ETH.