Skip to main content
Springer Nature Link
Log in

A Perception-Based Portfolio Under Uncertainty: Minimization of Average Rates of Falling

  • Conference paper
Modeling Decisions for Artificial Intelligence (MDAI 2009)

Part of the book series: Lecture Notes in Computer Science ((LNAI,volume 5861))

  • 877 Accesses

Abstract

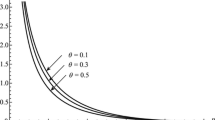
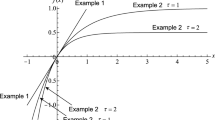
A perception-based portfolio model under uncertainty is discussed. In the proposed model, randomness and fuzziness are evaluated respectively by the probabilistic expectation and the mean values with evaluation weights and λ-mean functions. The means, the variances and the covariances fuzzy numbers/fuzzy random variables are evaluated in the possibility case and the necessity case, and the rate of return with portfolios is estimated by the both random factors and imprecise factors. In the portfolio model, the average rate of falling is minimized using average value-at-risks as a coherent risk measure. By analytical approach, we derive a solution of the portfolio problem to minimize the average rate of falling. A numerical example is given to illustrate our idea.

This is a preview of subscription content, log in via an institution to check access.

Access this chapter

Log in via an institution

Institutional subscriptions

Preview

Unable to display preview. Download preview PDF.

Unable to display preview. Download preview PDF.

Similar content being viewed by others

References

  1. Artzner, P., Delbaen, F., Eber, J.-M., Heath, D.: Coherent measures of risk. Mathematical Finance 9, 203–228 (1999)

    Article  MATH  MathSciNet  Google Scholar 

  2. Campos, L., Munoz, A.: A subjective approach for ranking fuzzy numbers. Fuzzy Sets and Systems 29, 145–153 (1989)

    Article  MATH  MathSciNet  Google Scholar 

  3. El Chaoui, L., Oks, M., Oustry, F.: Worst-case value at risk and robust portfolio optimization: A conic programming approach. Operations Research 51, 543–556 (2003)

    Article  MathSciNet  Google Scholar 

  4. Fortemps, P., Roubens, M.: Ranking and defuzzification methods based on area compensation. Fuzzy Sets and Systems 82, 319–330 (1996)

    Article  MATH  MathSciNet  Google Scholar 

  5. Inuiguchi, M., Tanino, T.: Portfolio selection under independent possibilistic information. Fuzzy Sets and Systems 115, 83–92 (2000)

    Article  MATH  MathSciNet  Google Scholar 

  6. Jorion, P.: Value at Risk: The New Benchmark for Managing Financial Risk, 3rd edn. McGraw-Hill, New York (2007)

    Google Scholar 

  7. Kruse, R., Meyer, K.D.: Statistics with Vague Data. Riedel Publ. Co., Dortrecht (1987)

    MATH  Google Scholar 

  8. Kwakernaak, H.: Fuzzy random variables – I. Definitions and theorem. Inform. Sci. 15, 1–29 (1978)

    Article  MATH  MathSciNet  Google Scholar 

  9. Markowitz, H.: Mean-Variance Analysis in Portfolio Choice and Capital Markets. Blackwell, Oxford (1990)

    Google Scholar 

  10. Pliska, S.R.: Introduction to Mathematical Finance: Discrete-Time Models. Blackwell Publ., New York (1997)

    Google Scholar 

  11. Puri, M.L., Ralescu, D.A.: Fuzzy random variables. J. Math. Anal. Appl. 114, 409–422 (1986)

    Article  MATH  MathSciNet  Google Scholar 

  12. Rockafellar, R.T., Uryasev, S.P.: Optimization of conditional value-at-risk. Journal of Risk 2, 21–42 (2000)

    Google Scholar 

  13. Steinbach, M.C.: Markowitz revisited: Mean-variance model in financial portfolio analysis. SIAM Review 43, 31–85 (2001)

    Article  MATH  MathSciNet  Google Scholar 

  14. Tanaka, H., Guo, P., Türksen, L.B.: Portfolio selection based on fuzzy probabilities and possibilistic distribution. Fuzzy Sets and Systems 111, 397 (2000)

    Article  Google Scholar 

  15. Yoshida, Y.: Mean values, measurement of fuzziness and variance of fuzzy random variables for fuzzy optimization. In: Proceedings of SCIS & ISIS 2006, September 2006, pp. 2277–2282 (2006)

    Google Scholar 

  16. Yoshida, Y.: A risk-minimizing model under uncertainty in portfolio. In: Melin, P., Castillo, O., Aguilar, L.T., Kacprzyk, J., Pedrycz, W. (eds.) IFSA 2007. LNCS (LNAI), vol. 4529, pp. 381–391. Springer, Heidelberg (2007)

    Chapter  Google Scholar 

  17. Yoshida, Y.: Fuzzy extension of estimations with randomness: The perception-based approach. In: Torra, V., Narukawa, Y., Yoshida, Y. (eds.) MDAI 2007. LNCS (LNAI), vol. 4617, pp. 295–306. Springer, Heidelberg (2007)

    Chapter  Google Scholar 

  18. Yoshida, Y.: An estimation model of value-at-risk portfolio under uncertainty. Fuzzy Sets and Systems (to appear) doi:10.1016/j.fss2009.02.007

    Google Scholar 

  19. Zmeškal, Z.: Value at risk methodology of international index portfolio under soft conditions (fuzzy-stochastic approach). International Review of Financial Analysis 14, 263–275 (2005)

    Article  Google Scholar 

  20. Zadeh, L.A.: Fuzzy sets. Inform. and Control 8, 338–353 (1965)

    Article  MATH  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Faculty of Economics and Business Administration, University of Kitakyushu, 4-2-1 Kitagata, Kokuraminami, Kitakyushu, 802-8577, Japan

    Yuji Yoshida

Authors
  1. Yuji Yoshida
    View author publications

    You can also search for this author in PubMed Google Scholar

Editor information

Editors and Affiliations

  1. IIIA-CSIC, Campus UAB s/n, 08193, Bellaterra, Catalonia, Spain

    Vicenç Torra

  2. Toho Gakuen, 3-1-10 Naka, Kunitachi, 186-0004, Tokyo, Japan

    Yasuo Narukawa

  3. Graduate School of Engineering Science, Osaka University, 1-3, Machikaneyama, Toyonaka, 560-8531, Osaka, Japan

    Masahiro Inuiguchi

Rights and permissions

Reprints and permissions

Copyright information

© 2009 Springer-Verlag Berlin Heidelberg

About this paper

Cite this paper

Yoshida, Y. (2009). A Perception-Based Portfolio Under Uncertainty: Minimization of Average Rates of Falling. In: Torra, V., Narukawa, Y., Inuiguchi, M. (eds) Modeling Decisions for Artificial Intelligence. MDAI 2009. Lecture Notes in Computer Science(), vol 5861. Springer, Berlin, Heidelberg. https://doi.org/10.1007/978-3-642-04820-3_14

Download citation

  • DOI: https://doi.org/10.1007/978-3-642-04820-3_14

  • Publisher Name: Springer, Berlin, Heidelberg

  • Print ISBN: 978-3-642-04819-7

  • Online ISBN: 978-3-642-04820-3

  • eBook Packages: Computer ScienceComputer Science (R0)

Publish with us

Policies and ethics