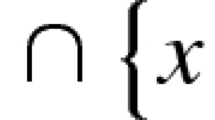Skip to main content
Springer Nature Link
Log in

Integrality Properties of Certain Special Balanceable Families

  • Conference paper
Combinatorial Algorithms (IWOCA 2009)

Part of the book series: Lecture Notes in Computer Science ((LNTCS,volume 5874))

Included in the following conference series:

  • 738 Accesses

Abstract

Balanceable clutters are clutters whose bipartite representation contains no odd wheel and no odd 3-path configuration as induced subgraph (this is Truemper’s characterization of balanceable matrices). In this paper we study a proper subclass of balanceable clutters called quasi-graphical defined by forbidding one-sided even wheels and one-sided even 3-path configurations. We characterize Mengerian quasi-graphical clutters and, as a consequence, we show that a recent conjecture in [5] is true for quasi-graphical clutters.

This is a preview of subscription content, log in via an institution to check access.

Access this chapter

Log in via an institution

Institutional subscriptions

Preview

Unable to display preview. Download preview PDF.

Unable to display preview. Download preview PDF.

Similar content being viewed by others

References

  1. Apollonio, N.: Integrality properties of edge path tree families. Discrete Mathematics 309, 4181–4184 (2009)

    Article  MATH  MathSciNet  Google Scholar 

  2. Apollonio, N., Caramia, M.: A superclass of Edge-Path-Tree graphs with few cliques. Operations Research Letters (in press, 2009), doi:10.1016/j.orl.2009.05.002

    Google Scholar 

  3. Apollonio, N., Caramia, M.: A local structure result for line graphs of strong quasi-graphical matrices. Technical Report DII RR-08.09, University of Rome Tor Vergata, http://dspace.uniroma2.it/dspace/handle/2108/655

  4. Conforti, M., Gerards, A.M.H., Kapoor, A.: A Theorem of Truemper. Combinatorica 20, 15–26 (2000)

    Article  MATH  MathSciNet  Google Scholar 

  5. Cornuéjols, G., Guenin, B., Margot, F.: The Packing Property. Mathematical Programming 89, 113–126 (2000)

    Article  MATH  MathSciNet  Google Scholar 

  6. Cornuéjols, G.: Combinatorial Optimization. Packing and Covering. SIAM, CBMS–NSF, Philadelphia (2001)

    MATH  Google Scholar 

  7. Fournier, J.-C.: Hypergraphes de Chaines d’ Aretes d’un Arbre. Discrete Mathematics 43, 29–36 (1983)

    Article  MATH  MathSciNet  Google Scholar 

  8. Golumbic, M.C., Jamison, R.E.: The Edge Intersection Graphs of Paths in a Tree. Journal of Combinatorial Theory, Series B 38, 8–22 (1985)

    Article  MATH  MathSciNet  Google Scholar 

  9. Schrijver, A.: Theory of Linear and Integer Programming. Wiley, Chichester (1986)

    MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Istituto per le Applicazioni del Calcolo, M. Picone, Via G. Amendola, 122/D, 70126, Bari, Italy

    Nicola Apollonio

  2. Dipartimento di Ingegneria dell’Impresa, Università di Roma “Tor Vergata”, Via del Politecnico 1, 00133, Roma, Italy

    Massimiliano Caramia

Authors
  1. Nicola Apollonio
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Massimiliano Caramia
    View author publications

    You can also search for this author in PubMed Google Scholar

Editor information

Editors and Affiliations

  1. Institute for Theoretical Computer Science and Department of Applied Mathematics, Charles University, Prague, Czech Republic

    Jiří Fiala  & Jan Kratochvíl  & 

  2. School of Electrical Engineering and Computer Science, The University of Newcastle, University Drive, NSW 2308, Callaghan, Australia

    Mirka Miller

Rights and permissions

Reprints and permissions

Copyright information

© 2009 Springer-Verlag Berlin Heidelberg

About this paper

Cite this paper

Apollonio, N., Caramia, M. (2009). Integrality Properties of Certain Special Balanceable Families. In: Fiala, J., Kratochvíl, J., Miller, M. (eds) Combinatorial Algorithms. IWOCA 2009. Lecture Notes in Computer Science, vol 5874. Springer, Berlin, Heidelberg. https://doi.org/10.1007/978-3-642-10217-2_8

Download citation

  • DOI: https://doi.org/10.1007/978-3-642-10217-2_8

  • Publisher Name: Springer, Berlin, Heidelberg

  • Print ISBN: 978-3-642-10216-5

  • Online ISBN: 978-3-642-10217-2

  • eBook Packages: Computer ScienceComputer Science (R0)

Publish with us

Policies and ethics