Abstract
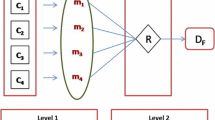
This paper proposes a method to acquire rules for evidential reasoning from multiple decision tables. The knowledge acquisition consists of two steps: the first step derives uncertain rules of the form: if a(u) is x, then d(u) is in Y 1 with r 1 or . . . or d(u) is in Y M with r M , where r j (j = 1, . . . ,M) are beliefs represented by basic belief assignment. The second step derives rules of the form: if a(u) is in X, then d(u) is in Y 1 with r 1 or . . . or d(u) is in Y M with r M , from the rules obtained in the first step. A non-specificity based condition imposed on rules generated in the second step is introduced. It is also shown that the disjunctive combination approach satisfies the condition.
This is a preview of subscription content, log in via an institution.
Buying options
Tax calculation will be finalised at checkout
Purchases are for personal use only
Learn about institutional subscriptionsPreview
Unable to display preview. Download preview PDF.
References
Klir, G.J., Yuan, B.: Fuzzy Sets and Fuzzy Logic. Prentice-Hall Inc., Englewood Cliffs (1995)
Liu, W., et al.: Representing heuristic knowledge and propagating beliefs in the Dempster-Shafer theory of evidence. In: Yager, R.R., et al. (eds.) Advances in the Dempster-Shafer Theory of Evidence, pp. 441–471. John Wiley & Sons, Inc., Chichester (1994)
Nishino, T., Nagamachi, M., Tanaka, H.: Variable precision Bayesian rough set model and its application to human evaluation data. In: Ślęzak, D., Wang, G., Szczuka, M.S., Düntsch, I., Yao, Y. (eds.) RSFDGrC 2005. LNCS (LNAI), vol. 3641, pp. 294–303. Springer, Heidelberg (2005)
Shafer, G.A.: Mathematical Theory of Evidence. Princeton Univ. Press, Princeton (1976)
Skowron, A., Grzymala-Busse, J.: From rough set theory to evidence theory, in the same book as [2], pp. 193–236 (1994)
Slezak, D., Ziarko, W.: The investigation of the Bayesian rough set model. Int. J. of Approximate Reasoning 40, 81–91 (2005)
Smets, P.: Belief functions: the disjunctive rule of combination and the generalized Bayesian theorem. Int. J. of Approximate Reasoning 9, 1–35 (1993)
Pawlak, Z.: Rough sets. Int. J. of Computer and Information Sciences 11, 341–356 (1982)
Ziarko, W.: Variable precision rough set model. Int. J. of Computer and System Sciences 46, 39–59 (1993)
Yamada, K., Kimala, V., Unehara, M.: A new conditioning rule, its generalization and evidential reasoning. In: IFSA/EUSFLAT 2009, Lisbon, pp. 92–98 (2009)
Yamada, K., Kimala, V., Unehara, M.: Knowledge Acquisition from Decision Tables for Evidential Reasoning. In: Proc. 19th Soft Science Workshop, pp. 9–16 (2009) (in Japanese)
Author information
Authors and Affiliations
Editor information
Editors and Affiliations
Rights and permissions
Copyright information
© 2010 Springer-Verlag Berlin Heidelberg
About this chapter
Cite this chapter
Yamada, K., Kimala, V. (2010). Acquiring Knowledge from Decision Tables for Evidential Reasoning. In: Huynh, VN., Nakamori, Y., Lawry, J., Inuiguchi, M. (eds) Integrated Uncertainty Management and Applications. Advances in Intelligent and Soft Computing, vol 68. Springer, Berlin, Heidelberg. https://doi.org/10.1007/978-3-642-11960-6_38
Download citation
DOI: https://doi.org/10.1007/978-3-642-11960-6_38
Publisher Name: Springer, Berlin, Heidelberg
Print ISBN: 978-3-642-11959-0
Online ISBN: 978-3-642-11960-6
eBook Packages: EngineeringEngineering (R0)