Abstract
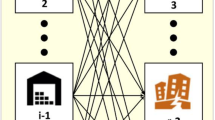
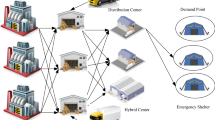
This paper reconsiders the single commodity allocation problem (SCAP) for disaster recovery, which determines where and how to stockpile a commodity before a disaster and how to route the commodity once the disaster has hit. It shows how to scale the SCAP algorithm proposed in [1] to a geographical area with up to 1,000 storage locations (over a million decision variables). More precisely, the paper shows that spatial and objective decompositions are instrumental in solving SCAP problems at the state scale (e.g., for the state of Florida). The practical benefits of these decompositions are demonstrated on large-scale hurricane disaster scenarios generated by Los Alamos National Laboratory using state-of-the-art disaster simulation tools.
Access this chapter
Tax calculation will be finalised at checkout
Purchases are for personal use only
Preview
Unable to display preview. Download preview PDF.
Similar content being viewed by others
References
Van Hentenryck, P., Bent, R., Coffrin, C.: Strategic Planning for Disaster Recovery with Stochastic Last Mile Distribution. In: [17], pp. 318–333
Wassenhove, L.V.: Humanitarian aid logistics: supply chain management in high gear. Journal of the Operational Research Society 57(1), 475–489 (2006)
Beamon, B.: Humanitarian relief chains: Issues and challenges. In: 34th International Conference on Computers & Industrial Engineering, pp. 77–82 (2008)
United-States Government: The Federal Response to Hurricane Katrina: Lessons Learned (2006)
Fritz Institute.: Fritz Institute Website (2008), http://www.fritzinstitute.org
Barbarosoglu, G., Ozdamar, L., Cevik, A.: An interactive approach for hierarchical analysis of helicopter logistics in disaster relief operations. European Journal of Operational Research 140(1), 118–133 (2002)
Duran, S., Gutierrez, M., Keskinocak, P.: Pre-positioning of emergency items worldwide for care international. Interfaces (2009) (to appear)
Balcik, B., Beamon, B., Smilowitz, K.: Last mile distribution in humanitarian relief. Journal of Intelligent Transportation Systems 12(2), 51–63 (2008)
Gunnec, D., Salman, F.: A two-stage multi-criteria stochastic programming model for location of emergency response and distribution centers. In: INOC (2007)
Campbell, A.M., Vandenbussche, D., Hermann, W.: Routing for relief efforts. Transportation Science 42(2), 127–145 (2008)
Griffin, P., Scherrer, C., Swann, J.: Optimization of community health center locations and service offerings with statistical need estimation. IIE Transactions (2008)
Gunes, C., van Hoeve, W.J., Tayur, S.: Vehicle routing for food rescue programs: A comparison of different approaches. In: [17], pp. 176–180
Toth, P., Vigo, D.: The Vehicle Routing Problem. SIAM Monographs on Discrete Mathematics and Applications, Philadelphia, Pennsylvania (2001)
Ignizio, J.P.: A review of goal programming: A tool for multiobjective analysis. The Journal of the Operational Research Society 29(11), 1109–1119 (1978)
FEMA: FEMA HAZUS Overview (2010), http://www.fema.gov/plan/prevent/hazus
Dynadec, Inc.: Comet 2.1 User Manual (2009), http://dynadec.com/
Lodi, A., Milano, M., Toth, P. (eds.): CPAIOR 2010. LNCS, vol. 6140. Springer, Heidelberg (2010)
Author information
Authors and Affiliations
Editor information
Editors and Affiliations
Rights and permissions
Copyright information
© 2011 Springer-Verlag Berlin Heidelberg
About this paper
Cite this paper
Coffrin, C., Van Hentenryck, P., Bent, R. (2011). Spatial and Objective Decompositions for Very Large SCAPs. In: Achterberg, T., Beck, J.C. (eds) Integration of AI and OR Techniques in Constraint Programming for Combinatorial Optimization Problems. CPAIOR 2011. Lecture Notes in Computer Science, vol 6697. Springer, Berlin, Heidelberg. https://doi.org/10.1007/978-3-642-21311-3_8
Download citation
DOI: https://doi.org/10.1007/978-3-642-21311-3_8
Publisher Name: Springer, Berlin, Heidelberg
Print ISBN: 978-3-642-21310-6
Online ISBN: 978-3-642-21311-3
eBook Packages: Computer ScienceComputer Science (R0)