Abstract
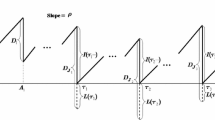
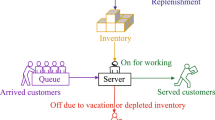
We consider a single-item lost sales inventory model with different classes of customers. Each customer class may have different lost sale penalty costs. We assume that the demands follow a Poisson process and we consider a single replenishment hypoexponential server. We give a Markov decision process associated with this optimal control problem and prove some structural properties of its dynamic programming operator. This allows us to show that the optimal policy is a critical level policy. We then discuss some possible extensions to other replenishment distributions and give some numerical results for the hyperexponential server case.
This is a preview of subscription content, log in via an institution to check access.
Access this chapter
Tax calculation will be finalised at checkout
Purchases are for personal use only
Preview
Unable to display preview. Download preview PDF.
Similar content being viewed by others
References
Altman, E.: Applications of Markov Decision Processes in communication networks. In: Feinberg, E.A., Shwartz, A. (eds.) Handbook of Markov Decision Processes, ch.16. Kluwer, Dordrecht (2002)
Dekker, R., Hill, R., Kleijn, M., Teunter, R.: On the (S-1,S) lost sales inventory model with priority demand classes. Naval Research Logistics 49, 593–610 (2002)
Glasserman, P., Yao, D.: Monotone Structure in Discrete-Event Systems. Wiley, Chichester (1994)
Ha, A.: Inventory rationing in a make-to-stock production system with several demand classes and lost sales. Management Science 43(8), 1093–1103 (1997)
Ha, A.: Stock rationing in an M/E k /1 make-to-stock queue. Management Science 46(1), 77–87 (2000)
Kleywegt, A.J., Papastavrou, J.D.: The dynamic and stochastic knapsack problem. Operations Research 46, 17–35 (1998)
Koole, G.: Monotonicity in Markov reward and decision chains: Theory and applications. Foundation and Trends in Stochastic Systems 1(1) (2006)
Kranenburg, A., van Houtum, G.: Cost optimization in the (S-1, S) lost sales inventory model with multiple demand classes. Oper. Res. Lett. 35(4), 493–502 (2007)
Lippman, S.: Applying a new device in the optimization of exponential queueing systems. Operations Research 23, 687–710 (1975)
Mazzucco, M., Mitrani, I., Palmer, J., Fisher, M., McKee, P.: Web service hosting and revenue maximization. In: Fifth European Conference on Web Services, ECOWS 2007, pp. 45–54 (2007)
Porteus, E.L.: Foundations of Stochastic Inventory Theory. Stanford University Press, Stanford (2002)
Puterman, M.: Markov Decision Processes Discrete Stochastic Dynamic Programming. Wiley, Chichester (2005)
Svoronos, A., Zipkin, P.: Evaluation of one-for-one replenishment policies for multiechelon inventory systems. Management Science 37(1) (1991)
de Véricourt, F., Karaesmen, F., Dallery, Y.: Optimal stock allocation for a capacitated supply system. Management Science 48, 1486–1501 (2002)
Wieczorek, A., Bušić, A., Hyon, E.: Critical level policies in lost sales inventory systems with different demand classes. Tech. rep., INRIA (2011)
Author information
Authors and Affiliations
Editor information
Editors and Affiliations
Rights and permissions
Copyright information
© 2011 Springer-Verlag Berlin Heidelberg
About this paper
Cite this paper
Wieczorek, A., Bušić, A., Hyon, E. (2011). Critical Level Policies in Lost Sales Inventory Systems with Different Demand Classes. In: Thomas, N. (eds) Computer Performance Engineering. EPEW 2011. Lecture Notes in Computer Science, vol 6977. Springer, Berlin, Heidelberg. https://doi.org/10.1007/978-3-642-24749-1_16
Download citation
DOI: https://doi.org/10.1007/978-3-642-24749-1_16
Publisher Name: Springer, Berlin, Heidelberg
Print ISBN: 978-3-642-24748-4
Online ISBN: 978-3-642-24749-1
eBook Packages: Computer ScienceComputer Science (R0)