Skip to main content
Springer Nature Link
Log in

Estimation of Integral Properties of a Planar Closed Curve Based on a Quadratic Spline Quasi-Interpolant

  • Conference paper
Curves and Surfaces (Curves and Surfaces 2010)

Part of the book series: Lecture Notes in Computer Science ((LNTCS,volume 6920))

Included in the following conference series:

  • 3390 Accesses

Abstract

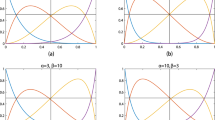
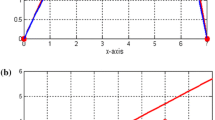
In this paper, we present a method based on a quadratic spline quasi-interpolant for the estimation of integral properties of a planar closed curve. The latter include the length, area, center of gravity and moment of inertia of the given curve. Then, we analyze the error estimates on the approximations of these properties and we validate the theoretical results by numerical examples.

This is a preview of subscription content, log in via an institution to check access.

Access this chapter

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Chapter
USD 29.95
Price excludes VAT (USA)
  • Available as PDF
  • Read on any device
  • Instant download
  • Own it forever

Tax calculation will be finalised at checkout

Purchases are for personal use only

Institutional subscriptions

Preview

Unable to display preview. Download preview PDF.

Unable to display preview. Download preview PDF.

Similar content being viewed by others

References

  1. Brauer, A.: Limits for the characteristic roots of a matrix II. Duke Math. J. 14, 21–26 (1947)

    Article  MathSciNet  MATH  Google Scholar 

  2. Davis, P.J., Vitale, R.A., Ben-Sabar, E.: On the deterministic and stochastic approximation of regions. J. Approx. Theory 21(1), 60–88 (1977)

    Article  MathSciNet  MATH  Google Scholar 

  3. Bailey, D.H., Crandall, R.E.: Experimental Mathematics 11(4), 527–546 (2002)

    Article  MathSciNet  Google Scholar 

  4. Dubuc, S., Merrien, J.-L., Sablonnière, P.: The length of the de Rham curve. J. Math. Anal. Appl. 223, 182–195 (1998)

    Article  MathSciNet  MATH  Google Scholar 

  5. Floater, M.: Arc length estimation and the convergence of parametric polynomial interpolation. Preprint CMA, Oslo (2005)

    Google Scholar 

  6. Floater, M.: Chordal cubic spline interpolation is fourth order accurate. IMA J. Numer. Anal. 26, 25–33 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  7. Floater, M., Rasmussen, A.F.: Point-based methods for estimating the length of a parametric curve. J. Comput. Applied Math. 196, 512–522 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  8. Kreyszig, E.: Differential Geometry. Dover, New York (1991)

    MATH  Google Scholar 

  9. Rasmussen, A.F., Floater, M.S.: A point-based method for estimating surface area. In: Field, D., Gonsor, D., Neamtu, M. (eds.) Electronic Proceedings of the SIAM Conference on Geometric Design, Phoenix (2005)

    Google Scholar 

  10. Sablonnière, P.: Comparison of some approximation methods for computing arc lengths. Congrés NTA (Nouvelles Tendances en Approximation). University of Oujda (October 2009)

    Google Scholar 

  11. Sablonnière, P.: Univariate spline quasi-interpolants and applications to numerical analysis. Rend. Sem. Mat. Univ. Pol. Torino 63(2), 107–118 (2005)

    MathSciNet  MATH  Google Scholar 

Download references

Authors
  1. C. Allouch
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. P. Sablonnière
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. D. Sbibih
    View author publications

    You can also search for this author in PubMed Google Scholar

Editor information

Editors and Affiliations

  1. INRIA Sophia-Antipolis, 2004 Route des Lucioles, BP 93, 06902, Sophia Antipolis, France

    Jean-Daniel Boissonnat

  2. Laboratoire LJK, University Joseph Fourier, BP 53, 38420, Grenoble Cedex 9, France

    Patrick Chenin

  3. Laboratoire Jacques-Louis Lions, Université Pierre et Marie Curie, 4, Place Jussieu, 75005, Paris, France

    Albert Cohen

  4. Laboratoire de Mathématiques, INSA Rouen, Avenue de l’Université, 76800, St. Etienne du Rouvray, France

    Christian Gout

  5. University of Oslo, DMA, Blindern, P.O.Box 1053, 0316, Oslo, Norway

    Tom Lyche

  6. Laboratoire LJK, Université Joseph Fourier, BP 53, 38041, Grenoble Cedex 9, France

    Marie-Laurence Mazure

  7. Department of Mathematics, Vanderbilt University, 37240, Nashville, TN, USA

    Larry Schumaker

Rights and permissions

Reprints and permissions

Copyright information

© 2012 Springer-Verlag Berlin Heidelberg

About this paper

Cite this paper

Allouch, C., Sablonnière, P., Sbibih, D. (2012). Estimation of Integral Properties of a Planar Closed Curve Based on a Quadratic Spline Quasi-Interpolant. In: Boissonnat, JD., et al. Curves and Surfaces. Curves and Surfaces 2010. Lecture Notes in Computer Science, vol 6920. Springer, Berlin, Heidelberg. https://doi.org/10.1007/978-3-642-27413-8_5

Download citation

  • DOI: https://doi.org/10.1007/978-3-642-27413-8_5

  • Publisher Name: Springer, Berlin, Heidelberg

  • Print ISBN: 978-3-642-27412-1

  • Online ISBN: 978-3-642-27413-8

  • eBook Packages: Computer ScienceComputer Science (R0)

Publish with us

Policies and ethics