Abstract
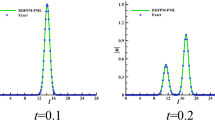
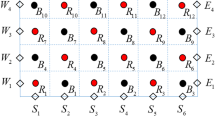
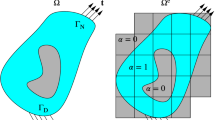
This paper presents a new hybrid solver based on Schur complement method, in which computations are distributed between multiple CPUs and GPUs. In this solver, the Schur complement is computed either on CPUs (for small problem size) or on GPUs (for large problem sizes). To solve the interface system, we propose a new multi-GPU algorithm that implements conjugate gradient method with explicit preconditioning. Experiments with wrap spring simulation on hybrid multi-core multi-GPU cluster demonstrate efficiency of the proposed method.
This is a preview of subscription content, log in via an institution to check access.
Access this chapter
Tax calculation will be finalised at checkout
Purchases are for personal use only
Preview
Unable to display preview. Download preview PDF.
Similar content being viewed by others
References
Giraud, L., Haidar, A., Saad, Y.: Sparse approximations of the Schur complement for parallel algebraic hybrid solvers in 3D. Numerical Mathematics 3, 276–294 (2010)
Gaidamour, J., Henon, P.: A parallel direct/iterative solver based on a Schur complement approach. In: IEEE 11th International Conference on Computational Science and Engineering, Sao Paulo, Brazil, pp. 98–105 (2008)
Agullo, E., Giraud, L., Guermouche, A., Roman, J.: Parallel hierarchical hybrid linear solvers for emerging computing platforms. Comptes Rendus Mecanique 339(2-3), 96–103 (2011)
Yamazaki, I., Li, X.S.: On techniques to improve robustness and scalability of a parallel hybrid linear solver. In: Palma, J.M.L.M., Daydé, M., Marques, O., Lopes, J.C. (eds.) VECPAR 2010. LNCS, vol. 6449, pp. 421–434. Springer, Heidelberg (2011)
Rajamanickam, S., Boman, E.G., Heroux, M.A.: Shylu: A hybrid-hybrid solver for multicore platforms. In: IEEE 26th International Parallel and Distributed Processing Symposium, IPDPS, pp. 631–643 (2012)
Przemieniecki, J.: Theory of Matrix Structural Analysis. McGaw-Hill, New York (1968)
Kopysov, S.P., Krasnoperov, I.V., Rychkov, V.N.: An object-oriented method for domain decomposition. Numerical Methods and Programming 4, 176–193 (2003)
Kopysov, S.P., Krasnopyorov, I.V., Novikov, A.K., Rychkov, V.N.: Parallel Distributed Object-Oriented Framework for Domain Decomposition, pp. 605–614. Springer (2006)
Saad, Y.: Iterative Methods for Sparse Linear Systems. SIAM (2003)
Kopysov, S.: Optimal domain decomposition for parallel sustructuring method. In: Mesh Methods for Boundary-Value Problems and Applications. Proceedings of 5th Russian Seminar, pp. 121–124. Kazan University, Kazan (2004)
Kopysov, S.P., Novikov, A.K.: Parallel adaptive mesh refinement with load balancing on heterogeneous cluster, pp. 425–432. Nova Science Publishers (2006)
Kopysov, S.P., Krasnoperov, I.V., Rychkov, V.N.: Implementation of an object-oriented model of domain decomposition on the basis of parallel distributed corba-components. Numerical Methods and Programming 4(1), 19–36 (2003)
Kopysov, S.P., Novikov, A.K., Sagdeeva, Y.A.: Solving of discontinuous galerkin method systems on gpu. Bulletin of Udmurt University. Mathematics. Mechanics. Computer Science (4), 121–131 (2011)
Karypis, G., Kumar, V.: Parallel multilevel k-way partitioning scheme for irregular graphs. SIAM Review 41(2), 278–300 (1999)
Author information
Authors and Affiliations
Editor information
Editors and Affiliations
Rights and permissions
Copyright information
© 2013 Springer-Verlag Berlin Heidelberg
About this paper
Cite this paper
Kopysov, S., Kuzmin, I., Nedozhogin, N., Novikov, A., Sagdeeva, Y. (2013). Hybrid Multi-GPU Solver Based on Schur Complement Method. In: Malyshkin, V. (eds) Parallel Computing Technologies. PaCT 2013. Lecture Notes in Computer Science, vol 7979. Springer, Berlin, Heidelberg. https://doi.org/10.1007/978-3-642-39958-9_6
Download citation
DOI: https://doi.org/10.1007/978-3-642-39958-9_6
Publisher Name: Springer, Berlin, Heidelberg
Print ISBN: 978-3-642-39957-2
Online ISBN: 978-3-642-39958-9
eBook Packages: Computer ScienceComputer Science (R0)