Abstract
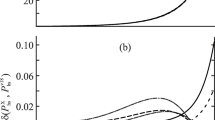
A simple formalism is proposed for a quantitative analysis of interatomic voids inside and outside a solute molecule in solution. It can be applied for the interpretation of volumetric data, obtained in studies of protein folding and unfolding in water. In particular, it helps to divide the partial molar volume of the solute into several components. The method is based on the Voronoi-Delaunay tessellation of molecular-dynamic models of solutions. It is suggested to select successive Voronoi shells, starting from the interface between the solute molecule and the solvent, and continuing to the outside (into the solvent) as well as into the inner of the molecule. Similarly, successive Delaunay layers, consisting of Delaunay simplexes, can also be constructed. Geometrical properties of the selected shells and layers are discussed. The temperature behavior of inner, boundary and outer shells is discussed by the example of a molecular-dynamic model of an aqueous solution of the polypeptide hIAPP.
Access this chapter
Tax calculation will be finalised at checkout
Purchases are for personal use only
Preview
Unable to display preview. Download preview PDF.
Similar content being viewed by others
References
Chalikian, T.V.: Volumetric properties of proteins: Annu. Rev. Biophys. Biomol. Struct. 32, 207–235 (2003)
Van der Spoel, D., Lindahl, E., Hess, B., Groenhof, G., Mark, A.E., Berendsen, H.J.C.: GROMACS: Fast, Flexible, and Free. J. Comp. Chem. 26(16), 1701–1718 (2005)
Medvedev, N.N.: Computational porosimetry. In: Engel, P., Syta, H. (eds.) Voronoi’s Impact on Modern Science, pp. 165–175. Institute of Math National Acad. of Sciences of Ukraine, Kiev (1998)
Sastry, S., Truskett, T.M., Debenedetti, P.G., Torquato, S., Stillinger, F.H.: Free Volume in the Hard-Sphere Liquid. Molecular Physics 95, 289–297 (1998)
Malavasi, G., Menziani, M.C., Pedone, A., Segre, U.: Void size distribution in MD-modelled silica glass structures. Journal of Non-Crystalline Solids 352, 285–296 (2006)
Luchnikov, V.A., Gavrilova, M.L., Medvedev, N.N., Voloshin, V.P.: The Voronoi-Delaunay approach for the free volume analysis of a packing of balls in a cylindrical container. Future Generation Computer Systems, Special Issue on Computer Modeling, Algorithms and Supporting Environments 18, 673–679 (2002)
Rémond, S., Gallias, J.L., Mizrahi, A.: Characterization of voids in spherical particle systems by Delaunay empty spheres. Granular Matter 10, 329–334 (2008)
Haw, M.D.: Void structure and cage fluctuations in simulations of concentrated suspensions. Soft Matter 2, 950–956 (2006)
Sung, B.J., Yethiraj, A.: Structure of void space in polymer solutions. Phys. Rev. E 81, 031801 (2010)
Alinchenko, M.G., Anikeenko, A.V., Medvedev, N.N., Voloshin, V.P., Mezei, M., Jedlovszky, P.: Morphology of voids in molecular systems. A Voronoi-Delaunay analysis of a simulated DMPC membrane. J. Phys. Chem. B 108(49), 19056–19067 (2004)
Anikeenko, A.V., Alinchenko, M.G., Voloshin, V.P., Medvedev, N.N., Gavrilova, M.L., Jedlovszky, P.: Implementation of the Voronoi-Delaunay Method for Analysis of Intermolecular Voids. In: Laganá, A., Gavrilova, M.L., Kumar, V., Mun, Y., Tan, C.J.K., Gervasi, O. (eds.) ICCSA 2004. LNCS, vol. 3045, pp. 217–226. Springer, Heidelberg (2004)
Edelsbrunner, H., Facello, M., Liang, J.: On the definition and construction of pockets in macromolecules. Discr. Appl. Math. 88, 83–102 (1998)
Liang, J., Edelsbrunner, H., Fu, P., Sudhakar, P., Subramaniam, S.: Analytical shape computation of macromolecules: II. Inaccessible cavities in proteins. Proteins: Struct. Func. Genet. 33, 18–29 (1998)
Kim, D., Cho, C.-H., Cho, Y., Ryu, J., Bhak, J., Kim, D.-S.: Pocket extraction on proteins via the Voronoi diagram of spheres. Journal of Molecular Graphics and Modelling 26(7), 1104–1112 (2008)
Raschke, T.M., Levitt, M.: Nonpolar solutes enhance water structure within hydration shells while reducing interactions between them. PNAS 102(19), 6777–6782 (2005)
Schröder, C., Rudas, T., Boresch, S., Steinhausera, O.: Simulation studies of the protein-water interface. I.Properties at the molecular resolution. J. Chem. Phys. 124, 234907 (2006)
Bouvier, B., Grünberg, R., Nilges, M., Cazals, F.: Shelling the Voronoi interface of protein-protein complexes predicts residue activity and conservation. Proteins: Structure, Function, and Bioinformatics 76(3), 677–692 (2008)
Neumayr, G., Rudas, T., Steinhausera, O.: Global and local Voronoi analysis of solvation shells of proteins. J. Chem. Phys. 133, 084108 (2010)
Voloshin, V.P., Medvedev, N.N., Andrews, M.N., Burri, R.R., Winter, R., Geiger, A.: Volumetric Properties of Hydrated Peptides: Voronoi-Delaunay Analysis of Molecular Simulation Runs. J. Phys. Chem. B 115(48), 14217–14228 (2011)
Okabe, A., Boots, B., Sugihara, K., Chiu, S.: Spatial tessellations - concepts and applications of Voronoi diagrams. John Wiley & Sons, New York (2000)
Medvedev, N.N.: Voronoi-Delaunay method for non-crystalline structures. SB of Russian Academy of Science, Novosibirsk (2000) (in Russian)
Richards, F.M.: Calculation of molecular volumes and areas for structures of known geo-metry. Methods in Enzymology 115, 440–464 (1985)
Gellatly, B.J., Finney, J.L.: Calculation of protein volumes: an alternative to the Voronoi procedure. J. Mol. Biol. 161, 305–322 (1982)
Anishchik, S.V., Medvedev, N.N.: Three-dimensional Apollonian packing as a model for dense granular systems. Phys.Rev.Lett. 75(23), 4314–4317 (1995)
Medvedev, N.N., Voloshin, V.P., Luchnikov, V.A., Gavrilova, M.L.: An algorithm for three-dimensional Voronoi S-network. J. Comput. Chem. 27, 1676–1692 (2006)
Aurenhammer, F.: Power diagrams: properties, algorithms and applications. SIAM J. Comput. 16, 78–96 (1987)
Kim, D.-S., Cho, Y., Sugihara, K.: Quasi-worlds and Quasi-operators on Quasi-triangulations. Computer-Aided Design 42(10), 874–888 (2010)
Aste, T., Szeto, K.Y., Tam, W.Y.: Statistical properties and shell analysis in random cellular structures. Phys.Rev.E 54(5), 5482–5492 (1996)
Andrews, M.N., Winter, R.: Comparing the Structural Properties of Human and Rat Islet Amyloid Polypeptide by MD Computer Simulations. Biophys. Chem. 156, 43–50 (2011)
Mitra, L., Smolin, N., Ravindra, R., Royer, C., Winter, R.: Pressure perturbation calorimetric study of the solvation properties and the thermal unfolding of proteins in solution - experiment and theoretical interpretation. Phys.Chem. Chem. Phys. 8, 1249–1265 (2006)
Imai, T.: Molecular theory of partial molar volume and its application to biomolecular systems. Cond. Matter Physics 10, 3(51), 343–361 (2007)
Author information
Authors and Affiliations
Editor information
Editors and Affiliations
Rights and permissions
Copyright information
© 2013 Springer-Verlag Berlin Heidelberg
About this chapter
Cite this chapter
Kim, A.V., Voloshin, V.P., Medvedev, N.N., Geiger, A. (2013). Decomposition of a Protein Solution into Voronoi Shells and Delaunay Layers: Calculation of the Volumetric Properties. In: Gavrilova, M.L., Tan, C.J.K., Kalantari, B. (eds) Transactions on Computational Science XX. Lecture Notes in Computer Science, vol 8110. Springer, Berlin, Heidelberg. https://doi.org/10.1007/978-3-642-41905-8_5
Download citation
DOI: https://doi.org/10.1007/978-3-642-41905-8_5
Publisher Name: Springer, Berlin, Heidelberg
Print ISBN: 978-3-642-41904-1
Online ISBN: 978-3-642-41905-8
eBook Packages: Computer ScienceComputer Science (R0)