Skip to main content
SpringerLink
Log in

Exact Soliton Solutions to the Nano-Bioscience and Biophysics Equations Through the Modified Simple Equation Method

  • Conference paper
  • First Online:
Proceedings of the Sixth International Conference on Mathematics and Computing

Part of the book series: Advances in Intelligent Systems and Computing ((AISC,volume 1262))

Abstract

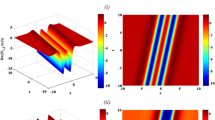
The nano-ionic currents along microtubules (MTs) and the equation of microtubules in nano-biosciences as nonlinear RLC transmission line are very significant nonlinear evolution equations (NLEEs) in biological physics and applied mathematics. The modified simple equation (MSE) method is useful, functional, and efficacious to extract exact soliton solutions. But, when the balance number is greater than one, it is challenging to find out the solutions. In this article, we have put in use the MSE method to ascertain some solutions accessible in the literature and establish some new soliton solutions to the equations described earlier each of which has a balance number two. In the first instance, we have established a general solution comprising some subjective parameters. We analyze the solitary wave properties of the solutions by depicting 3D graphs.

This is a preview of subscription content, log in via an institution to check access.

Access this chapter

Log in via an institution
Chapter
USD 29.95
Price excludes VAT (USA)
  • Available as PDF
  • Read on any device
  • Instant download
  • Own it forever
eBook
USD 169.00
Price excludes VAT (USA)
  • Available as EPUB and PDF
  • Read on any device
  • Instant download
  • Own it forever
Softcover Book
USD 219.99
Price excludes VAT (USA)
  • Compact, lightweight edition
  • Dispatched in 3 to 5 business days
  • Free shipping worldwide - see info

Tax calculation will be finalised at checkout

Purchases are for personal use only

Institutional subscriptions

Similar content being viewed by others

References

  1. Tala-Tebue E, Zayed EME (2018) New Jacobi elliptic function solutions solitons and other solutions for the (2+1)-dimensional nonlinear electrical transmission line equation. European Phys. J. Plus 133:314

    Article  Google Scholar 

  2. Ganji DD, Afrouzi GA, Talarposhti RA (2007) Application of variational iteration method and homotopy perturbation method for nonlinear heat diffusion and heat transfer equations. Phys. Lett. A 368:450–457

    Article  Google Scholar 

  3. Zayed EME, Amer YA, Shohib RMA (2014) The improved generalized Riccati equation mapping method and its application for solving a nonlinear partial differential equation (PDE) describing the dynamics of ionic currents along microtubules. Academic J. 9(8):238–248

    Google Scholar 

  4. Jawad AJM, Petkovic MD, Laketa P, Biswas A (2013) Dynamics of shallow water waves with Boussinesq equation, Scientia Iranica. Trans. B: Mech. Engr. 20(1):179–184

    Google Scholar 

  5. Guo AL, Lin J (2010) Exact solutions of (2+1)-dimensional HNLS equation. Commun. Theor. Phys. 54:401–406

    Article  MathSciNet  Google Scholar 

  6. Ablowitz MJ, Clarkson PA (1991) Soliton, nonlinear evolution equations and inverse scattering. Cambridge University Press, New York

    Book  Google Scholar 

  7. Hirota R (2004) The direct method in soliton theory. Cambridge University Press, Cambridge

    Book  Google Scholar 

  8. Akbar MA, Ali NHM, Zayed EME (2012) Abundant exact traveling wave solutions of the generalized Bretherton equation via \((G^\prime /G)\)-expansion method. Commun. Theor. Phys. 57:173–178

    Article  MathSciNet  Google Scholar 

  9. Alam MN, Akbar MA, Mohyud-Din ST (2014) General traveling wave solutions of the strain wave equation in microstructured solids via the new approach of generalized \((G^\prime /G)\)-expansion method. Alexandria Engg. J. 53:233–241

    Article  Google Scholar 

  10. K.K. Ali, A.M. Wazwaz and M.S. Osman, Optical soliton solutions to the generalized nonautonomous nonlinear Schrodinger equations in optical fibers via the Sine-Gordon expansion method, Optik, https://doi.org/10.1016/j.ijleo.2019.164132(in press-available online 26 December 2019)

  11. Helal MA, Mehana MS (2006) A comparison between two different methods for solving Boussinesq-Burgers equation. Chaos, Solitons Fract. 28:320–326

    Article  Google Scholar 

  12. Akbar MA, Ali NHM, Hussain J (2019) Optical soliton solutions to the (2+1) Chaffee-Infante equation and dimensionless form of the Zakharov equation. Adv. Differ. Equ. 2019:446

    Article  MathSciNet  Google Scholar 

  13. Islam MS, Akbar MA, Khan K (2018) Analytical Solutions of nonlinear Klein-Gordon equation using the F-expansion method. Opt. Quant. Electron. 50:224

    Article  Google Scholar 

  14. Triki H, Kara AH, Biswas A (2014) Domain walls to Boussinesq type equations in (2+1)-dimensions. Indian J. Phys. 88(7):751–755

    Article  Google Scholar 

  15. H. Naher, A.F. Abdullah and M.A. Akbar, New traveling wave solutions of the higher dimensional nonlinear partial differential equation by the Exp-function method, J. Appl. Math., 2012 (2012) Article ID 575387, 14 pages

    Google Scholar 

  16. Wang M (1995) Solitary wave solutions for variant Boussinesq equations. Phy. Lett. A 199:169–172

    Article  MathSciNet  Google Scholar 

  17. M.G. Hafez, M.N. Alam and M.A. Akbar, Traveling wave solutions for some important coupled nonlinear physical models via the coupled Higgs equation and the Maccari system, J. King Saud Univ.-Sci., 27 (2015), 105-112

    Google Scholar 

  18. Bock TL, Kruskal MD (1979) A two-parameter Miura transformation of the Benjamin-One equation. Phys. Lett. A 74:173–176

    Article  MathSciNet  Google Scholar 

  19. Sekulic DL, Sataric MV, Zivanov MB (2011) Symbolic computation of some new nonlinear partial differential equations of nano-biosciences using modified extended tanh-function method. Appl. Math. Comput. 218:3499–3506

    MathSciNet  MATH  Google Scholar 

  20. Khan K, Akbar MA, Alam MN (2013) Traveling wave solutions of the nonlinear Drinfel’d-Sokolov-Wilson equation and modified Benjamin-Bona-Mahony equations. J. Egyptian Math. Soc. 21:233–240

    Article  MathSciNet  Google Scholar 

  21. Kamruzzaman Khan and M. Ali Akbar, Exact and solitary wave solutions for the Tzitzeica-Dodd-Bullough and the modified Boussinesq-Zakharov-Kuznetsov equations using the modified simple equation method, Ain Shams Engr. J., 4 (2013) 903-909

    Google Scholar 

  22. K. Khan and M.A. Akbar, Traveling wave solutions of some coupled nonlinear evolution equations, ISRN Math. Phys., 2013 (2013) Article ID 685736, 8 pages

    Google Scholar 

  23. Islam MS, Roshid MM, Rahman AKML, Akbar MA (2019) Solitary wave solutions in plasma physics and acoustic gravity waves of some nonlinear evolution equations through enhanced MSE method. J. Phys. Commun. 3(12):125011

    Article  Google Scholar 

  24. Sataric MV, Sekulic D, Zivanov M (2010) Solitonic ionic currents along microtubules. J. Comp. Th. Nanosc. 7(11):1–10

    Google Scholar 

  25. Sekulic DL, Sataric BM, Tuszynski JA, Sataric MV (2011) Nonlinear ionic pulses along microtubules. Eur. Phys. J. E. 34(5):1–11

    Article  Google Scholar 

Download references

Acknowledgment

The authors acknowledge the research grant No. A-1220/5/52/RU/Science-37/2019–2020.

Author information

Authors and Affiliations

  1. Department of Applied Mathematics, University of Rajshahi, Rajshahi, Bangladesh

    Md. Abdul Kayum, Hemonta Kumar Barman & M. Ali Akbar

Authors
  1. Md. Abdul Kayum
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Hemonta Kumar Barman
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. M. Ali Akbar
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to M. Ali Akbar .

Editor information

Editors and Affiliations

  1. Department of Information Technology, Maulana Abul Kalam Azad University of Technology, Haringhata, West Bengal, India

    Debasis Giri

  2. School of Computing and Information Systems, University of Melbourne, Melbourne, VIC, Australia

    Rajkumar Buyya

  3. Department of Mathematics, Indian Institute of Technology Madras, Chennai, India

    S. Ponnusamy

  4. Department of Computer Science and Engineering, Maulana Abul Kalam Azad University of Technology, Haringhata, West Bengal, India

    Debashis De

  5. Unconventional Computing Laboratory, Department of Computer Science and Creative Technologies, University of the West of England, Bristol, UK

    Andrew Adamatzky

  6. Faculty of Science, Engineering and Built Environment, Deakin University Geelong, Geelong, VIC, Australia

    Jemal H. Abawajy

Rights and permissions

Reprints and permissions

Copyright information

© 2021 The Author(s), under exclusive license to Springer Nature Singapore Pte Ltd.

About this paper

Check for updates. Verify currency and authenticity via CrossMark

Cite this paper

Kayum, M.A., Barman, H.K., Akbar, M.A. (2021). Exact Soliton Solutions to the Nano-Bioscience and Biophysics Equations Through the Modified Simple Equation Method. In: Giri, D., Buyya, R., Ponnusamy, S., De, D., Adamatzky, A., Abawajy, J.H. (eds) Proceedings of the Sixth International Conference on Mathematics and Computing. Advances in Intelligent Systems and Computing, vol 1262. Springer, Singapore. https://doi.org/10.1007/978-981-15-8061-1_38

Download citation

Publish with us

Policies and ethics

Search

Navigation