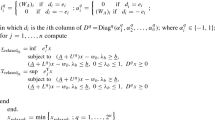Abstract
Several authors have used interval arithmetic to deal with parametric or sensitivity analysis in mathematical programming problems. Several reported computational experiments have shown how interval arithmetic can provide such results. However, there has not been a characterization of the resulting solution interval in terms of the usual sensitivity analysis results. This paper presents a characterization of perturbed convex programs and the resulting solution intervals.
Interval arithmetic was developed as a mechanism for dealing with the inherent error associated with numerical computations using a computational device. Here it is used to describe error in the parameters. We show that, for convex programs, the resulting solution intervals can be characterized in terms of the usual sensitivity analysis results. It has been often reported in the literature that even well behaved convex problems can exhibit pathological behavior in the presence of data perturbations. This paper uses interval arithmetic to deal with such problems, and to characterize the behavior of the perturbed problem in the resulting interval. These results form the foundation for future computational studies using interval arithmetic to do nonlinear parametric analysis.
Similar content being viewed by others
References
E. Adams and R. Lohner, “Error bounds and sensitivity analysis,” in: R. Stepleman et al. eds.,Scientific Computing (North-Holland, Amsterdam, 1983) pp. 213–222.
J. Dinkel and M. Tretter, “An interval arithmetic approach to sensitivity analysis in geometric programming,”Operations Research 35 (1987) 859–866.
J. Dinkel, M. Tretter and D. Wong, “Interval Newton methods and perturbed problems,”Applied Mathematics and Computation 28 (1988) 211–222.
A.V. Fiacco,Introduction to Sensitivity and Stability Analysis in Non-linear Programming (Academic Press, New York, 1983
E.R. Hansen, “Global optimization using interval analysis—The multi-dimensional case”,Numerische Mathematik 34 (1980) 247–270.
E.R. Hansen, “Global optimization with data perturbations,”Computers and Operations Research 11 (1984) 97–104.
L.J. Mancini and G.P. McCormick, “Bounding global minima,”Mathematics of Operations Research 1 (1976) 50–53.
L.J. Mancini and G.P. McCormick, “Bounding global minima with interval arithmetic,”Operations Research 27 (1979) 743–754.
R.E. Moore,Interval Analysis (Prentice-Hall, Englewood Cliffs, NJ, 1966).
R.E. Moore,Methods and Applications of Interval Analysis (SIAM, Philadelphia, PA, 1979).
H. Ratschek and J. Rokne,New Computer Methods for Global Optimization (Ellis Horwood, Chichester, UK, 1988).
S.M. Robinson, “Computable error bounds for nonlinear programming,”Mathematical Programming 5 (1973) 235–242.
S. Zlobec, “Characterizing an optimal input in perturbed convex programming,”Mathematical Programming 25 (1983) 109–121.
Author information
Authors and Affiliations
Rights and permissions
About this article
Cite this article
Dinkel, J.J., Tretter, M.J. Characterization of perturbed mathematical programs and interval analysis. Mathematical Programming 61, 377–384 (1993). https://doi.org/10.1007/BF01582158
Received:
Revised:
Issue Date:
DOI: https://doi.org/10.1007/BF01582158