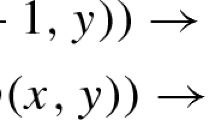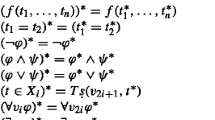Abstract
IΣ n andBΣ n are well known fragments of first-order arithmetic with induction and collection forΣ n formulas respectively;IΣ 0n andBΣ 0n are their second-order counterparts. RCA0 is the well known fragment of second-order arithmetic with recursive comprehension;WKL 0 isRCA 0 plus weak König's lemma. We first strengthen Harrington's conservation result by showing thatWKL 0 +BΣ 0 n is Π 11 -conservative overRCA 0 +BΣ 0 n . Then we develop some model theory inWKL 0 and illustrate the use of formalized model theory by giving a relatively simple proof of the fact thatIΣ 1 provesBΣ n+1 to be Π n+2-conservative overIΣ n . Finally, we present a proof-theoretic proof of the stronger fact that theΠ n+2 conservation result is provable already inIΔ 0 + superexp. ThusIΣ n+1 proves 1-Con (BΣ n+1) andIΔ 0 +superexp proves Con (IΣ n )↔Con(BΣ n+1).
Similar content being viewed by others
References
Bell, J., Machover, M.: A course in mathematical, logic, 2nd edn. Amsterdam New York Oxford Tokyo: North-Holland 1988
Buss, S.R.: Bounded arithmetic. Studies in proof theory. Napoli: Bibliopolis 1986
Clote, P.G.: Partition relations in arithmetic. In: DiPrisco, C.A. (ed.) Methods in mathematical logic. Proceedings, Caracas 1983. (Lect Notes Math., vol. 1130, pp. 32–68. Berlin Heidelberg New York:Springer 1985
Clote, P.G.: Applications of the low basis theorem in arithmetic. In: Ebbinghaus, H.-D., Müller, G.H., Sacks, G.E. (eds.) Recursion theory week. Proceedings, Oberwolfach, 1984. (Lect. Notes Math., vol. 1141, pp. 65–88). Berlin Heidelberg New York: Springer 1985
Clote, P.G.: Ultrafilters on definable sets in arithmetic. In: Paris, J.B., Wilkie, A.J., Wilmers, G.M. (eds.) Methods in mathematical logic. Logic Colloquium '84, pp. 37–58. Amsterdam New York Oxford Tokyo: North-Holland 1986
Dimitracopoulos, C.: Matijasevic's theorem and fragments of arithmetic. Ph.D. thesis, University of Manchester, 1980
Friedman, H.M.: Some systems of second-order arithmetic and their use. Proceedings of the International Congress of Mathematicians in Vacounver 1974, pp. 235–242 (1975)
Friedman, H.M.: Systems of second-order arithmetic with limited induction (Abstract). J. Symb. Logic41, 557–559 (1976)
Gaifman, H., Dimitracopoulos, C.: Fragments of Peano's arithmetic and the MRDP theorem. In: Logic and Algorithm. Monogr. No. 30, l'Enseignement Mathématique, Université de Genève, Geneva, pp. 187–206 (1982)
Girard, J.-Y.: Proof theory and logical complexity, vol. 1, p. 503. Napoli: Bibliopolis 1987, 503 pages
Hájek, P., Paris, J.B.: Combinatorial principles concerning approximation of functions. Arch. Math. Logic,26, 13–28 (1986)
Jockusch, C.G., Jr., Soare, R.I.: Π 01 -classes and degrees of theories. Trans. Am. Math. Soc.173, 33–56 (1972)
Hájek, P., Kučera, A.: On recursion theory in IΣ1. J. Symb. Logic,54, 576–589 (1989)
Paris, J.B.: A hierarchy of cuts in models of airthmetic. In: Pacholski, L., Wierzejewski, J., Wilkie, A.J. (eds.). Model theory of algebra and arithmetic. Proceedings, Karpacz, Poland 1979. (Lect. Notes Math., vol. 834, pp. 312–337). Berlin Heidelberg New York: Springer 1979
Paris, J.B.: Some conservation results for fragments of arithmetic. In: Berline, C., McAloon, K., Ressayre, J.-P. (eds.) Model theory and arithmetic. Proceedings, Paris 1979/80. (Lect. Notes Math., vol. 890, pp. 251–262). Berlin Heidelberg New York: Springer 1981
Paris, J., Kirby, L.:Σ n -collection schemas in arithmetic. In: Logic Colloquium '77, pp. 199–209. Amsterdam New York Oxford Tokyo: North-Holland 1978
Robinson, R.M.: An essentially undecidable axiom system. Proc. Int. Cong. Math., vol. I, pp. 729–730. Cambridge, MA 1950
Shoenfield, J.R.: Mathematical Logic, 2nd edn. Addison-Wesley 1967
Sieg, W.: Provably recursive functionals of theories with König's lemma, (to appear in Rend. Sem. Mat. Univers. Politecn. Torino, Fasc. Speciale Logic & Comp. Sc.: some of the results of this article were announed in the Logic Colloquium '85 in Orsay, France)
Takeuti, G.: Proof. theory, 2nd edn., vol. 81. Studies in logic and the foundation of mathematics. Amsterdam New York Oxford Tokyo: North-Holland 1987
Wilkie, A.J., Paris, J.B.: On the scheme of induction for bounded formulas in arithmetic. Ann. Pure Appl. Logic35, 261–302 (1987)
Woods, A.: Some problems in logic and number theory. Ph.D. thesis, University of Manchester (1981)
Author information
Authors and Affiliations
Additional information
The first author was partially supported by NSF Grant #DCR-860615
Rights and permissions
About this article
Cite this article
Clote, P., Hájek, P. & Paris, J. On some formalized conservation results in arithmetic. Arch Math Logic 30, 201–218 (1990). https://doi.org/10.1007/BF01792983
Received:
Revised:
Issue Date:
DOI: https://doi.org/10.1007/BF01792983