Skip to main content
Springer Nature Link
Log in

On distance-regular graphs with fixed valency, II

  • Published:
Graphs and Combinatorics Aims and scope Submit manuscript

Abstract

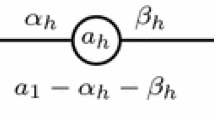
LetГ be a distance-regular graph of valencyk ≥ 3 and diameterd. Suppose the intersection array hast columns different fromt(1, 0,k − 1). Then it is shown thatd is bounded from above by a certain functionf(k, t) depending only onk andt. As an application, this theorem eliminates certain cubic distance-regular graphs to complete the classification of such graphs by Biggs et al.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Bannai, E., Ito, T.: On distance-regular graphs with fixed valency. Graphs and Combinatorics3, 95–109 (1987)

    Google Scholar 

  2. Bannai, E., Ito, T.: Algebraic Combinatorics, I (Benjamin/Cummings Lecture Note Series) California: Menlo Park 1984

    Google Scholar 

  3. Biggs, N.L.: Algebraic Graph Theory. Cambridge: Cambridge University Press 1974

    Google Scholar 

  4. Biggs, N.L., Boshier, A.G., Shawe-Taylor, J.: Cubic distance-regular graphs. J. London Math. Soc. (2)33, 385–394 (1986)

    Google Scholar 

  5. Cameron, P.J., Praeger, C.E., Saxl, J., Seitz, G.M.: On the Sims conjecture and distance-transitive graphs. Bull. Lond. Math. Soc.15, 499–506 (1983)

    Google Scholar 

  6. Chihara, T.S.: An Introduction to Orthogonal Polynomials. NY: Gordon and Breach 1978

    Google Scholar 

  7. Ito, T.: Bipartite distance-regular graphs of valency 3. Linear Algebra Appl.46, 195–213 (1982)

    Google Scholar 

  8. Ivanov, A.A.: Bounding the diameter of a distance-regular graph. Sov. Math. Dokl.28, 149–152 (1983)

    Google Scholar 

  9. Szegö, G.: Orthogonal Polynomials. Amer. Math. Soc. Colloq. Publ.23, AMS, Providence, R.I., 4th edition 1975, (1st edition in 1939)

    Google Scholar 

  10. Weiss, R.: The nonexistence of 8-transitive graphs. Combinatorica1, 309–311 (1981)

    Google Scholar 

  11. Weiss, R.: Distance-transive graphs and generalized polygons. Arch. Math.45, 186–192 (1985)

    Google Scholar 

  12. Weiss, R.: On distance-transive graphs. Bull. Lond. Math. Soc.17, 253–256 (1985)

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. School of Mathematical Sciences, Queen Mary College, University of London, Mile End Road, E1, 4NS, London, U.K.

    Eiichi Bannai

  2. Department of Mathematics, Joetsu University of Education, 943, Joetsu, Niigata, Japan

    Tatsuro Ito

  3. Department of Mathematics, Ohio State University, 43210, Columbus, OH, USA

    Eiichi Bannai

Authors
  1. Eiichi Bannai
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Tatsuro Ito
    View author publications

    You can also search for this author inPubMed Google Scholar

Additional information

Supported in part by NSF grant MCS-8301826 and by the British SERC grant.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Bannai, E., Ito, T. On distance-regular graphs with fixed valency, II. Graphs and Combinatorics 4, 219–228 (1988). https://doi.org/10.1007/BF01864162

Download citation

  • Received:

  • Revised:

  • Issue Date:

  • DOI: https://doi.org/10.1007/BF01864162

Keywords