Abstract
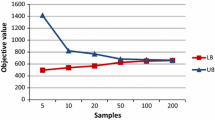
In many service systems, the primary objective is to provide continuous service and/or service within a prespecified time interval. In the public sector, emergency service systems fit into this category. In the private sector, systems providing repair service to critical production facilities and computers constitute another example. In these systems, the concept of multiple service facilities providing backup to each other becomes an important element in the design process. In this paper, we study the capacitated facility siting problem with multiple levels of backup coverage. The problem is formulated as a mathematical program; an efficient solution procedure is developed and computational experiments are reported.
Similar content being viewed by others
References
M.S. Bazaara and J.J. Goode, A survey of various tactics for generating Lagrangrian multipliers in the context of Lagrangian duality, Eur. J. Oper. Res. 3(1979)322–338.
G.N. Berlin, Facility location and vehicle allocation for provision of emergency service, Ph.D. Dissertation, The Johns Hopkins University (1972).
C.H. Chung, D.A. Schilling and R. Carbone, The capacitated maximal covering problem: A heuristic, in:Proc. 14th Annual Pittsburgh Conf. on Modeling and Simulation (1983), pp. 1423–1428.
R.L. Church and C.S. ReVelle, The maximal covering location problem, Papers of the Regional Sci. Assoc. 32(1974)101–118.
R.L. Church and C.S. ReVelle, Theoretical and computational links between thep-median, location set-covering, and the maximal covering location problem, Geograph. Anal. 8(1976)406–415.
J. Current and J. Storbeck, Capacitated covering models, Ohio State University Working Paper Series WPS 87–112 (Oct. 1987).
M.S. Daskin and E.N. Stern, A hierarchical objective set covering model for emergency medical service deployment, Transp. Sci. 15(1981)137–152.
H. Everett, Generalized Lagrange multipliers for solving problems of optimum allocation of resources, Oper. Res. 11(1963)399–417.
M.L. Fisher, The Lagrangian relaxation method for solving integer programming problems, Manag. Sci. 27(1981)1–18.
M.L. Fisher, An applications oriented guide to Lagrangian relaxation, Interfaces 15(1985)10–21.
M.R. Garey and D.S. Johnson,Computers and Intractability: A Guide to the Theory of NP-Completeness (Freeman, San Francsico, 1979).
B. Gavish and H. Pirkul, Computer and database location in distributed computer systems, IEEE Trans. Comput. C-35(1986)583–590.
B. Gavish and H. Pirkul, Distributed computer system design for large decentralized organizations,Proc. Int. Conf. on Information Systems, San Diego (1986).
A.M. Geoffrion and R. McBride, Lagrangian relaxation applied to capacitated facility location problems, AIIE Trans. 10(1978)40–47.
M. Held and R.M. Karp, The traveling salesman problem and minimum spanning trees, Oper. Res. 18(1970)1138–1162.
M. Held, P. Wolfe and H.B. Crowder, Validation of subgradient optimization, Math. Progr. 6(1974)62–88.
K. Hogan and C.S. ReVelle, Concepts and applications of backup coverage, Manag. Sci. 32(1986)1434–1444.
E.L. Johnson and M.W. Padberg, A note on the knapsack problem with special ordered sets, Oper. Res. Lett. 1(1981)18–22.
R.M. Nauss and R.E. Markland, Theory and applications of an optimization procedure for lock box location analysis, Manag. Sci. 27(1981)855–865.
H. Pirkul, Allocating users and their databases among processing sites of a distributed computer system, Eur. J. Oper. Res. 26(1986)401–411.
H. Pirkul, Efficient algorithms for the capacitated concentrator location problem, Comput. Oper. Res. 14(1987)197–208.
H. Pirkul and D. Schilling, The siting of emergency service facilities with workload capacities and backup services, Manag. Sci. 34(1988)896–908.
H. Pirkul and D. Schilling, The capacitated maximal covering location problem with backup service, Ann. Oper. Res. 18(1989)141–154.
H. Pirkul and D. Schilling, The maximal covering location problem with capacities on total service, Manag. Sci. 37(1991)233–248.
B.T. Poljack, A general method of solving extremum problems, Sov. Math. Dokl. 8 (1967).
T.W. Ruefli and J.E. Storbeck, Behaviorally linked location hierarchies, Environ. Planning B9(1982)257–268.
J.R. Weaver and R.L. Church, A median location model with nonclosest facility service, Transp. Sci. 19(1985)58–74.
Author information
Authors and Affiliations
Rights and permissions
About this article
Cite this article
Narasimhan, S., Pirkul, H. & Schilling, D.A. Capacitated emergency facility siting with multiple levels of backup. Ann Oper Res 40, 323–337 (1992). https://doi.org/10.1007/BF02060485
Issue Date:
DOI: https://doi.org/10.1007/BF02060485