Skip to main content
Springer Nature Link
Log in

On the complexity of simultaneous price-quantity adjustment processes

  • Published:
Annals of Operations Research Aims and scope Submit manuscript

Abstract

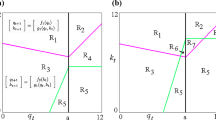
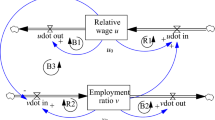
While the Walrasian price tâtonnement represents the traditional dynamic process in the general equilibrium context with and without production, Walras and other classics designed the process exclusively for pure exchange economies. In productive economies, the short-run output adjustment of existing firms and the entry/exit of firms should be modeled as well. So-called cross-dual processes which represent the classical approach to the dynamics of productive economies are discussed and extended. Complex motion can emerge in a discrete-time version of the original two-dimensional system when the aggregate demand function has a non-standard shape. A simultaneous process of price and short-run quantity adjustment with free entry and exit of competitive firms in a single market with a continuum of firms can generate closed orbits via a Hopf bifurcation when the slope of the demand function is positive at equilibrium. When the continuum economy is replaced by an economy with a finite number of firms, noisy limit cycles and complicated behavior can be observed.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. K.J. Arrow, Toward a theory of price adjustment, in:The Allocation of Economic Resources, ed. M. Abramowitz (Stanford University Press, Stanford, 1959) pp. 41–51.

    Google Scholar 

  2. K.J. Arrow and L. Hurwitz, On the stability of the competitive equilibrium. I, Econometrica 26(1958)522–552.

    Google Scholar 

  3. R. Aumann, Markets with a continuum of traders, Econometrica 32(1964)39–50.

    Google Scholar 

  4. M.J. Beckmann and H.E. Ryder, Simultaneous price and quantity adjustment in a single market, Econometrica 37(1969)470–484.

    Google Scholar 

  5. J.P. Benassy,The Economics of Market Disequilibrium (Academic Press, New York, 1982).

    Google Scholar 

  6. V. Böhm,Disequilibrium and Macroeconomics (Basil, Oxford, 1989).

    Google Scholar 

  7. D.G. Davies, A note on Marshallian versus Walrasian stability conditions, Can. J. Econ. Political Sci. 29(1963)535–540.

    Google Scholar 

  8. G. Debreu, Excess demand functions, J. Math. Econ. 1(1974)15–23.

    Google Scholar 

  9. G. Dumenil and D. Levy, The dynamics of competition: A restoration of the classical analysis, Cambridge J. Econ. 11(1987)133–164.

    Google Scholar 

  10. P. Flaschel, Stability — independent of economic structure? A prototype analysis, Mimeo, University of Bielefeld, (1989).

  11. P. Flaschel and W. Semmler, Classical and neoclassical competitive adjustment processes, The Manchester School 55(1987)13–37.

    Google Scholar 

  12. P. Flaschel and W. Semmler, On the integration of dual and cross-dual adjustment processes in Leontief systems, Ricerche Economiche 12(1988)403–432.

    Google Scholar 

  13. W. Fukuda, The output adjustment mechanism in a multisectoral economy, Kobe University Econ. Rev. 21(1975)53–62.

    Google Scholar 

  14. G. Gandolfo, G. Martinengo and P.C. Padoan,Qualitative Analysis and Econometric Estimation of Continuous Time Dynamic Models (North-Holland, Amsterdam, 1981).

    Google Scholar 

  15. R.M. Goodwin, Static and dynamic general equilibrium models (1953). Reprinted in: R.M. Goodwin,Essays in Linear Economic Structures (MacMillan, London, 1983).

    Google Scholar 

  16. R.M. Goodwin,Elementary Economics from the Higher Standpoint (Cambridge University Press, Cambridge, 1970).

    Google Scholar 

  17. F. Hahn, Stability, in:Handbook of Mathematical Economics, Vol. 2, ed. K.J. Arrow and M.D. Intriligator (North-Holland, Amsterdam, 1984) pp. 745–793.

    Google Scholar 

  18. F. Hahn and T. Negishi, A theorem on non-tâtonnement stability, Econometrica 30(1962)463–469.

    Google Scholar 

  19. H. Haken,Advanced Synergetics (Springer, Berlin-Heidelberg-New York, 1983).

    Google Scholar 

  20. J.R. Hicks,Value and Capital, 2nd ed. (Clarendon Press, Oxford, 1946).

    Google Scholar 

  21. W. Hildenbrand, On the "Law of Demand", Econometrica 51(1983)997–1020.

    Google Scholar 

  22. H.-W. Lorenz,Nonlinear Dynamical Economics and Chaotic Motion (Springer, Berlin-Heidelberg-New York, 1989).

    Google Scholar 

  23. H.-W. Lorenz,Determinismus, nichtlineare Dynamik und wirtschaftliche Evolution (University of Göttingen, 1990).

  24. F.R. Marotto, Snap-back repellers imply chaos in IRn, J. Math. Anal. Appl. 72(1978)199–223.

    Google Scholar 

  25. A. Marshall,Principles of Political Economy, 8th ed. (MacMillan, London, 1938).

    Google Scholar 

  26. A. Marshall, The pure theory of foreign trade (1879). Reprinted in:The Early Economic Writings of Alfred Marshall, 1867–1890, ed. J.K. Whitaker (MacMillan, London, 1975) pp. 117–181.

    Google Scholar 

  27. A. Mas-Colell, Notes on price and quantity tâtonnement dynamics, in:Models of Economic Dynamics, ed. H. Sonnenschein (Springer, Berlin-Heidelberg-New York, 1986) pp. 49–68.

    Google Scholar 

  28. A. Medio, Synergetics and dynamic economic models, in:Nonlinear Models of Fluctuating Growth, ed. R.M. Goodwin, M. Krüger and A. Vercelli (Springer, Berlin-Heidelberg-New York, 1984) pp. 166–191.

    Google Scholar 

  29. M. Morishima, A reconsideration of the Walras-Cassel-Leontief model of general equilibrium, in:Mathematical Models in the Social Sciences, ed. K.J. Arrow, S. Karlin and P. Suppes (Stanford University Press, Stanford, 1960), pp. 63–76.

    Google Scholar 

  30. P. Newman,The Theory of Exchange (Prentice-Hall, Englewood Cliffs, 1965).

    Google Scholar 

  31. W. Novshek and H. Sonnenschein, Quantity adjustment in an Arrow-Debreu-McKenzie type model, in:Models of Economic Dynamics, ed. H. Sonnenschein (Springer, Berlin-Heidelberg-New York, 1986) pp. 148–156.

    Google Scholar 

  32. W. Novshek and H. Sonnenschein, General equilibrium with free entry, J. Econ. Literature 25(1987)1281–1306.

    Google Scholar 

  33. P.A. Samuelson,Foundations of Economic Analysis (Harvard University Press, Cambridge, 1947).

    Google Scholar 

  34. W. Shafer and H. Sonnenschein, Market demand and excess demand functions, in:Handbook of Mathematical Economics, Vol. 2, ed. K.J. Arrow and M.D. Intriligator (North-Holland, Amsterdam, 1982) pp. 672–693.

    Google Scholar 

  35. H. Sonnenschein, Price dynamics and the disappearance of short-run profits, J. Math. Econ. 8(1981)201–206.

    Google Scholar 

  36. H. Sonnenschein, Price dynamics based on the adjustment of firms, Amer. Econ. Rev. 72(1982)1088–1096.

    Google Scholar 

  37. C. Sparrow, Bifurcation and chaotic behaviour in simple feedback systems, J. Theor. Biol. 83(1980)93–105.

    Google Scholar 

  38. L.E. Svensson, Walrasian and Marshallian stability, J. Econ. Theory 34(1984)371–379.

    Google Scholar 

  39. A. Takayama,Mathematical Economics (The Dryden Press, Hinsdale).

  40. H. Uzawa, The stability of dynamic processes, Econometrica 29(1961)617–631.

    Google Scholar 

  41. D.A. Walker, Walras's theories of tâtonnement, J. Political Econ. 95(1987)758–774.

    Google Scholar 

  42. L. Walras,Elements of Pure Economics (George Allen and Unwin, London, 1954).

    Google Scholar 

  43. A. Wolf, J.B. Swift, H.L. Swinney and J.A. Vastano, Determining Lyapunov exponents from a time series, Physica 16D(1985)285–317.

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Economics, Georg-August-Universität Göttingen, D.W. 3400, Göttingen, Germany

    Hans-Walter Lorenz

Authors
  1. Hans-Walter Lorenz
    View author publications

    You can also search for this author inPubMed Google Scholar

Rights and permissions

Reprints and permissions

About this article

Cite this article

Lorenz, HW. On the complexity of simultaneous price-quantity adjustment processes. Ann Oper Res 37, 51–71 (1992). https://doi.org/10.1007/BF02071048

Download citation

  • Issue Date:

  • DOI: https://doi.org/10.1007/BF02071048

Keywords