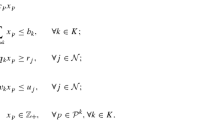Abstract
We consider the objective function of a simple integer recourse problem with fixed technology matrix and discretely distributed right-hand sides. Exploiting the special structure of this problem, we devise an algorithm that determines the convex hull of this function efficiently. The results are improvements over those in a previous paper. In the first place, the convex hull of many objective functions in the class is covered, instead of only one-dimensional versions. In the second place, the algorithm is faster than the one in the previous paper. Moreover, some new results on the structure of the objective function are presented.
Similar content being viewed by others
References
R.L. Graham, An efficient algorithm for determining the convex hull of a finite planar set, Information Processing Letters 1, 1972, 132–133.
W.K. Klein Haneveld, L. Stougie and M.H. van der Vlerk, On the convex hull of the simple integer recourse objective function, Annals of Operations Research 56, 1995, 209–224.
W.K. Klein Haneveld, L. Stougie and M.H. van der Vlerk, Some theorems on the convex hull of a function with an application to stochastic integer programming, Discussion Paper TI 94-81, Tinbergen Institute, Amsterdam-Rotterdam, 1994.
F.V. Louveaux and M.H. van der Vlerk, Stochastic programming with simple integer recourse, Mathematical Programming 61, 1993, 301–325.
R.T. Rockafellar,Convex Analysis, Princeton University Press, Princeton, NJ, 1970.
R.T. Rockafellar and R.J-B Wets,Variational Analysis, Springer, Berlin, in preparation.
R. Schultz, Continuity and stability in two-stage stochastic integer programming, inLecture Notes in Economics and Mathematical Systems, vol. 379, K. Marti, ed., Springer, Berlin, 1992, pp. 81–92.
M.H. van der Vlerk, Stochastic programming with integer recourse, Ph.D. Thesis, University of Groningen, The Netherlands, 1995.
Author information
Authors and Affiliations
Rights and permissions
About this article
Cite this article
Haneveld, W.K.K., Stougie, L. & van der Vlerk, M.H. An algorithm for the construction of convex hulls in simple integer recourse programming. Ann Oper Res 64, 67–81 (1996). https://doi.org/10.1007/BF02187641
Issue Date:
DOI: https://doi.org/10.1007/BF02187641