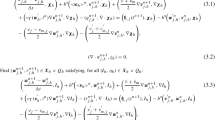Abstract
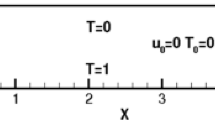
In this paper, we consider the successive overrelaxation method with projection for obtaining the finite element solutions under the nonlinear radiation boundary conditions. In particular we establish the convergence of the successive overrelaxation method with projection. Some numerical results are also given to illustrate the usefulness.
Zusammenfassung
In dieser Arbeit betrachten wir das sukzessive Überrelaxationsverfahren mit Projektion zu finiten Elementlösungen unter nichtlinearen Radiationsrandbedingungen, insbesondere den Nachweis einer Konvergenz des sukzessiven Überrelaxationsverfahrens mit Projektion. An einigen numerischen Ergebnissen soll die Anwendbarkeit illustriert werden.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
Explore related subjects
Discover the latest articles, news and stories from top researchers in related subjects.References
Aubin, J. P.: Applied Functional Analysis. New York: John Wiley 1979.
Brewster, M. E., Kannan, R.: Nonlinear successive over-relaxation. Numer. Math.44, 309–315 (1984).
Brewster, M. E., Kannan, R.: Global convergence of nonlinear successive overrelaxation via linear theory. Computing34, 73–79 (1985).
Ciarlet, P. G.: Discrete maximum principle for finite-difference operators. Aequationes Math.4, 338–352 (1970).
Ciarlet, P. G.: Introduction à l'Analyse Numérique Matricielle et à l'Optimisation. Paris: Masson 1982.
Ciarlet, P. G., Raviart, P. A.: Maximum principle and uniform convergence for the finite element method. Comput. Methods Appl. Mech. Engrg.2, 17–31 (1973).
Cohen, D. S.: Generalized radiation cooling of a convex solid. J. Math. Anal. Appl.35, 503–511 (1971).
Concus, P.: Numerical solution of the minimal surface equation. Math. Comp.21, 340–350 (1967).
Concus, P.: Numerical solution of the nonlinear magnetostatic-field equation in two dimensions. J. Comput. Phys.1, 330–342 (1967).
Cryer, C. W.: The solution of a quadratic programming problem using systematic overrelaxation. SIAM J. Control9, 385–392 (1971).
Glowinski, R.: Numerical Methods for Nonlinear Variational Problems. New York: Springer-Verlag 1984.
Glowinski, R., Marrocco, A.: Analyse numérique du champ magnétique d'un altenateur par éléments finis et sur-relaxation ponctuelle non linéaire. Compt. Methods Appl. Mech. Engrg.3, 55–85 (1974).
Goldstein, A. A.: Constructive Real Analysis. New York: Harper & Row 1967.
Ishihara, K.: Finite element solutions for radiation cooling problems with nonlinear boundary conditions. RAIRO Modélisation Mathématique et Analyse Numérique20, 461–477 (1986).
Ishihara, K.: Explicit monotone iterations providing upper and lower bounds for finite element solution with nonlinear radiation boundary conditions. Computing37, 137–149 (1986).
Olmstead, W. E.: Temperature distribution in a convex solid with nonlinear radiation boundary condition. J. Math. Mech.15, 899–908 (1966).
Ortega, J. M., Rheinboldt, W. C.: Iterative Solution of Nonlinear Equations in Several Variables. New York: Academic Press 1970.
Ortega, J. M., Rockoff, M. L.: Nonlinear difference equations and Gauss-Seidel type iterative methods. SIAM J. Numer. Anal.3, 497–513 (1966).
Schechter, S.: Iteration methods for nonlinear problems. Trans. Amer. Math. Soc.104, 179–189 (1962).
Varga, R. S.: Matrix Iterative Analysis. Englewood Cliffs, N. J.: Prentice-Hall 1962.
White, R. E.: An Introduction to the Finite Element Method with Applications to Nonlinear Problems. New York: John Wiley 1985.
Author information
Authors and Affiliations
Rights and permissions
About this article
Cite this article
Ishihara, K. Successive overrelaxation method with projection for finite element solutions of nonlinear radiation cooling problems. Computing 38, 117–132 (1987). https://doi.org/10.1007/BF02240177
Received:
Issue Date:
DOI: https://doi.org/10.1007/BF02240177