Skip to main content
SpringerLink
Log in

Numerical solution of the obstacle problem by the penalty method

Numerische Lösung des Hindernis-Problems mit der Penalty-Methode

  • Contibuted Papers
  • Published:
Computing Aims and scope Submit manuscript

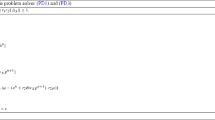
Abstract

The penalty method is used to compute approximations of the solution of the obstacle problem. By coupling the penalty parameter ε and the discretization parameterh quasi-optimal error estimates in the energy-norm are derived.

Zusammenfassung

Die Penalty-Methode wird benutzt, um Approximationen für die Lösung des Hindernis-Problems zu berechnen. Durch Kopplung des Penalty-Parameters ε und des Diskretisierungs-Parametersh werden quasi-optimale Fehlerabschätzungen in der Energie-Norm hergeleitet.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Brezis, H., Sibony, M.: Méthodes d'approximation et d'itération pour les opérateurs monotones. Archive Rat. Mech. Anal.28, 59–82 (1968).

    Google Scholar 

  2. Brezis, H., Stampacchia, G.: Sur la régularité de la solution d'inéquations elliptiques. Bull. Soc. Math. France96, 153–180 (1968).

    Google Scholar 

  3. Brezzi, F., Hager, W. W., Raviart, P. A.: Error estimates for the finite element solution of variational inequalities. Part I: Primal theory. Numer. Math.28, 431–443 (1977).

    Google Scholar 

  4. Brezzi, F., Hager, W. W., Raviart, P. A.: Error estimates for the finite element solution of variational inequalities. Part II: Mixed methods. Numer. Math.31, 1–16 (1978).

    Google Scholar 

  5. Falk, R. S.: Error estimates for the approximation of a class of variational inequalities. Math. of Comp.28, 963–971 (1974).

    Google Scholar 

  6. Fučik, S., Kufner, A.: Nonlinear differential equations. Amsterdam-Oxford-New York: Elsevier 1980.

    Google Scholar 

  7. Gilbarg, D., Trudinger, N. S.: Elliptic partial differential equations of second order. Berlin-Heidelberg-New York: Springer 1977.

    Google Scholar 

  8. Glowinski, R.: Finite elements and variational inequalities. The Mathematics of Finite Elements and Applications III. Mafelap 1978. Proc. Conf. Brunel Univ., 1978, pp. 135–171. London-New York-San Francisco: Academic Press 1979.

    Google Scholar 

  9. Kinderlehrer, D., Stampacchia, G.: An introduction to variational inequalities and their applications. New York-London-Toronto-Sydney-San Francisco: Academic Press 1980.

    Google Scholar 

  10. Lions, J. L.: Quelques méthodes de résolution des problèmes aux limites non linéaires. Paris: Dunod-Gauthier-Villars 1969.

    Google Scholar 

  11. Nitsche, J.:L ∞-convergence of finite element approximations. Math. Aspects of Finite Element Methods. Rome, 1975, pp. 261–274. Berlin-Heidelberg-New York: Springer 1977.

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Institut für Angewandte Mathematik, Albert-Ludwig-Universität, Hermann-Herder-Strasse 10, D-7800, Freiburg i. Breisgau, Federal Republic of Germany

    R. Scholz

Authors
  1. R. Scholz
    View author publications

    You can also search for this author in PubMed Google Scholar

Additional information

Dedicated to Professor Dr. K. Nickel on the occasion of his 60th birthday.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Scholz, R. Numerical solution of the obstacle problem by the penalty method. Computing 32, 297–306 (1984). https://doi.org/10.1007/BF02243774

Download citation

  • Received:

  • Issue Date:

  • DOI: https://doi.org/10.1007/BF02243774

AMS Subject Classifications

Key words

Search

Navigation