Abstract
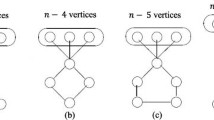
The interval numberi (G) of a graphG withn vertices is the lowest integerm such thatG is the intersection graph of some family of setsI 1, ...,I n with everyI i being the union of at mostm real intervals.
In this article, an idea is presented for the algorithmic determination ofi (G), ifG is triangle-free. An example for the application of these considerations is given.
Zusammenfassung
Als Intervallzahli (G) eines GraphenG mitn Ecken bezeichnet man die kleinste natürliche Zahlm, so daßG der Schnittgraph einer Familie von MengenI 1, ...,I n ist, bei der jedesI i aus der Vereinigung von höchstensm reellen Intervallen besteht. Für den Fall, daßG dreikreisfrei ist, wird eine Idee zur algorithmischen Bestimmung voni (G) angegeben. Ein Anwendungsbeispiel wird ebenfalls angegeben.
Similar content being viewed by others
Explore related subjects
Discover the latest articles, news and stories from top researchers in related subjects.References
Golumbic, M. C.: Algorithmic graph theory and perfect graphs. New York-London: Academic Press 1980.
Griggs, J. R.: Extremal values of the interval number of a graph II. Disc. Math.28, 37–47 (1979).
Griggs, J. R., West, D. B.: Extremal values of the interval number of a graph. SIAM J. Alg. Disc. Meth.1, 1–7 (1980).
Maas, C.: Some results about the interval number of a graph. Discrete Applied Mathematics6, 99–102 (1983).
Maas, C.: A lower bound for the interval number of a graph. (To appear.)
Roberts, F. S.: Discrete mathematical models. Englewood Cliffs, N. J.: Prentice-Hall 1976.
Trotter, W. T., Harary, F.: On double and multiple interval graphs. J. Graph Theory3, 205–211 (1979).
Author information
Authors and Affiliations
Rights and permissions
About this article
Cite this article
Maas, C. Determining the interval number of a triangle-free graph. Computing 31, 347–354 (1983). https://doi.org/10.1007/BF02251237
Received:
Issue Date:
DOI: https://doi.org/10.1007/BF02251237