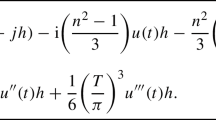Abstract
Quadrature formulas based on the “practical” abscissasx k=cos(k π/n),k=0(1)n, are obtained for the numerical evaluation of the weighted Cauchy principal value integrals
where α,β>−1 andaε(−1, 1). An interesting problem concerning these quadrature formulas is their convergence for a suitable class of functions. We establish convergence of these quadrature formulas for the class of functions which are Hölder-continuous on [−1, 1].
Zusammenfassung
Ermittelt werden die auf den “praktischen” Abszissenx k=cos(k π/n),k=0(1)n, basierten Quadraturformeln für die numerische Berechnung von Cauchyschen gewichteten Hauptwerten
wobei α,β>−1 undaε(−1, 1). Ein interessantes Problem bezüglich dieser Quadraturformeln ist ihre Konvergenz für die Klasse von Funktionen, die auf [−1, 1] Hölder-kontinuierlich sind.
Similar content being viewed by others
Explore related subjects
Discover the latest articles, news and stories from top researchers in related subjects.References
Chawla, M. M., Jayarajan N.: Quadrature formulas for Cauchy principal value integrals. Computing15, 347–355 (1975).
Elliott, D., Paget, D. F.: On the convergence of a quadrature rule for evaluating certain Cauchy principal value integrals. Numer. Math.23, 311–319 (1975).
Elliott, D., Paget, D. F.: On the convergence of a quadrature rule for evaluating certain Cauchy principal value integrals: An addendum. Numer. Math.25, 287–289 (1976).
Kalandiya, A. I.: On a direct method of solution of an equation in wing theory and its application to the theory of elasticity.Mat. sb.42, 249–272 (1957).
Muskhelishvili, N. I.: Singular integral equations. Groningen, Holland: Noordhoff 1953.
McCabe, J. H., Phillips, G. M.: On a certain class of Lebesgue constants. B. I. T.13, 434–442 (1973).
Paget, D. F., Elliott, D.: An algorithm for the numerical evaluation of certain Cauchy principal value integrals. Numer. Math.19, 373–385 (1972).
Rainville, E. D.: Special functions. New York: Macmillan 1960.
Author information
Authors and Affiliations
Rights and permissions
About this article
Cite this article
Chawla, M.M., Sheo Kumar Convergence of quadratures for Cauchy principal value integrals. Computing 23, 67–72 (1979). https://doi.org/10.1007/BF02252614
Received:
Issue Date:
DOI: https://doi.org/10.1007/BF02252614