Skip to main content
Springer Nature Link
Log in

Lotka's law reconsidered: The evolution of publication and citation distributions in scientific fields

  • Published:
Scientometrics Aims and scope Submit manuscript

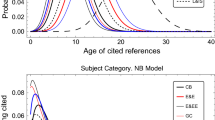
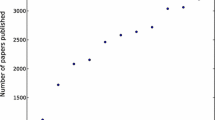
Abstract

This paper reports early steps in research that seeks to clarify how publications of scientists interact dynamically with citations and reputation in shaping the evolution of scientific fields. We assume that Lotka's modified law holds for scientific fields. A primary approach to model publication productivity was published by Yablonsky. In contrast to Yablonsky's unfinished mathematical approach, our simulation approach is not predominantly driven by insight into the formal generation mechanisms of certain processes but more theory driven. It considers the evolution of publication and citation distributions over the histories of scientific fields using both simulated and real historical data.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  • Bookstein, A. (1990a), Informetric distributions. Part I: Unified overview.Journal of the American Society for Information Science, 41, 368–375.

    Article  Google Scholar 

  • Bookstein, A. (1990b), Informetric distributions. Part II: Resilience to ambiguity.Journal of the American Society for Information Science, 41, 376–388.

    Article  Google Scholar 

  • Bookstein, A. (1997), Informetric distributions. Part III: Ambiguity and randomness.Journal of the American Society for Information Science, 48, 2–10.

    Article  Google Scholar 

  • Bookstein, A., B. Wright (1997), Ambiguity in measurement.Scientometrics, 40, 369–384.

    Article  Google Scholar 

  • Braun, T., W. Glänzel, A. Schubert (1990), Publication productivity. From frequency distributions to scientometric indicators.Journal of Information Science, 16, 37–44.

    Google Scholar 

  • Chattoe, E., N. J. Saam, M. Möhring (forthcoming): Sensitivity analysis in the social sciences: problems and prospects. In:G. N. Gilbert, U. Mueller, R. Suleiman, K. G. Troitzsch (Eds.):Social Science Microsimulation: Tools for Modeling, Parameter Optimization, and Sensitivity Analysis. Berlin: Springer.

  • Fahrmeir, L. (1997),Statistik—der Weg zur Datenanalyse. Berlin: Springer.

    Google Scholar 

  • Fox, M. F. (1983), Publication productivity among scientists. A critical review.Social Studies of Science, 13, 285–305.

    Google Scholar 

  • Glänzel, W., A. Schubert (1990), The Cumulated Advantage Function. A mathematical formulation based on conditional expectations and its application to scientometric distributions. In:L. Egghe, R. Rousseau (Eds.).Informetrics 89/90. Proceedings of the 2nd International Conference on Bibliometrics, Scientometrics and Informetrics, held in London (Canada), 4–7 July, 1989. Amsterdam: Elsevier Science Publishers: 139–147.

    Google Scholar 

  • Hogg, R. V. (1974), Adaptive robust procedures. A partial review and some suggestions for future applications and theory.Journal of the American Statistical Assiciation, 69, 909–927.

    Article  MATH  MathSciNet  Google Scholar 

  • Ijiri, Y., H. Simon (1977),Skew distributions and the sizes of business firms. Amsterdam: North-Holland.

    Google Scholar 

  • Lotka, A. J. (1926). The frequency distribution of scientific productivity.Journal of the Washington Academy of Science, 16, 317–323.

    Google Scholar 

  • MacRoberts, M. H., B. R. MacRoberts (1982), A re-evaluation of Lotka's Law of scientific productivity,Social Studies of Science, 12, 449–450.

    Google Scholar 

  • Mandelbrot, B. (1959), A note on a class of skew distribution functions: analysis and critique of a paper by H. A. Simon.Information and Control, 2, 90–99.

    Article  MATH  MathSciNet  Google Scholar 

  • Mayer, K. U. Ed. (1993),Generationsdynamik in der Forschung. Frankfurt: Campus.

    Google Scholar 

  • Merton, R. K. (1968). The Matthew effect in science,Science, 159, 56–63.

    Google Scholar 

  • Möhring, M. (1996), Social science multilevel simulation with MIMOSE. In:K. G. Troitzsch, U. Mueller, G. N. Gilbert, J. E. Doran (Eds.).Social Science Microsimulation. Berlin: Springer: 123–137.

    Google Scholar 

  • Möhring, M., R. Ostermann (1996),MIMOSE. Eine funktionale Sprache zur Beschreibung und Simulation individuellen Verhaltens in interagierenden Populationen. Einführung in die Modellierung. Univ. Koblenz.

  • Price, D. J. de Solla (1986a),Little science, big science—and beyond. New York: Columbia Univ. Press. 2nd edition. Originally published 1963.

    Google Scholar 

  • Price, D. J. de Solla (1986b), Studies in Scientometrics, Part 1: Transience and Continuance in Scientific Authorship. In:Price 1986a: 206–226. Originally published 1975.

  • Price, D. J. de Solla (1976), A general theory of bibliometric and other cumulative advantage processes.Journal of the American Society for Information Science, 27, 292–306.

    Google Scholar 

  • Price, D. J. de Solla (1963),Little science, big science. New York: Columbia Univ. Press.

    Google Scholar 

  • Rao, R. I. K. (1995), A stochastic approach to analysis of distribution of papers in mathematics: Lotka's Law revisited. In:M. E. D. Koenig, A. Bookstein (Eds).Proceedings of the 5th International Conference on Scientometrics & Informetrics, June 7–10, 1995, Chicago/Illinois: 455–464.

  • Rao, R. I. K. (1988), Probability distributions and inequality measures for analyses of circulation data. In:L. Egghe, R. Rousseau (Eds.).Informetrics 87/88. Selected proceedings of the First International Conference on Bibliometrics and Theoretical Aspects of Information Retrieval held at Diepenbeek, Belgium, 25–28 August 1987. Amsterdam: Elsevier Science Publishers: 231–248.

    Google Scholar 

  • Reiter, L. (1995), Das Konzept der „Klinischen Nützlichkeit”. Theoretische Grundlagen und Praxisbezug.Zeitschrift für systemische Therapie, 13, 193–211.

    Google Scholar 

  • Reiter, L. (1994), Leitfiguren der Familientherapie und systemischen Therapie.Psychotherapieforum, 2, 137–143.

    Google Scholar 

  • Reiter, L., E. Steiner, U. Werner (1997), Ordnungsstrukturen im Wissenschaftsbetrieb. Untersuchungen Psychotherapie. In:G. Schiepek, W. Tschacher (Eds).Synergetik in Psychologie und Psychiatrie, Braunschweig: Vieweg. S. 328–343.

    Google Scholar 

  • Saam, N. J. (1996),Computergestützte Theoriekonstruktion in den Sozialwissenschaften. Konzeptbasierte Simulation eines theoretischen Modells am Beispiel militärischer Staatsstreiche in Thailand. Unter Anwendung des Mehrebenen-Ansatzes der Synergetik. San Diego, Erlangen: Society for Computer Simulation International.

    Google Scholar 

  • Saam, N. J. Simulating the Micro-Macro Link: New Approaches to an Old Problem and an Application to Military Coups.Sociological Methodology, to be published.

  • Schubert, A., W. Glänzel (1991), Publication dynamics. Models and indicators.Scientometrics, 20, 317–331.

    Article  Google Scholar 

  • Sachs, L. (1992),Angewandte Statistik, 7. ed. Berlin: Springer.

    Google Scholar 

  • Sen, S. K., S. K. Chatterjee (1995), Mean Relative Scatter (MRS)—A linear equation for bibliometric distributions: Further empirical tests. In:M. E. D. Koenig, A. Bookstein (Eds).Proceedings of the 5th International Conference on Scientometrics & Informetrics, June 7–10, 1995, Chicago, Illinois: 505–514.

  • Stephan, P. E., S. G. Levin (1991), Inequality in scientific performance: Adjustment for attribution and journal impact.Social Studies of Science, 21, 351–368.

    Google Scholar 

  • Tague, J. (1981), The success-breeds-success phenomenon and bibliometric processes. In:Journal of the American Society for Information Science, 32, 280–286.

  • Weidlich, W., G. Haag (1983),Quantitative Sociology, Berlin: Springer.

    Google Scholar 

  • Whitley, R. D. (1972), Kommunikationsnetze in der Wissenschaft. Status und Zitierungsmuster in der Tierphysiologie. In:P. Weingart (Ed.),Wissenschaftssoziologie. Frankfurt: Athäneum Fischer: 188–202.

    Google Scholar 

  • Yablonsky, A. I. (1980), On fundamental regularities of the distribution of scientific productivity.Scientometrics, 2, 3–34.

    Article  Google Scholar 

  • Zuckerman, H. (1993), Die Werdegänge von Nobelpreisträgern. In:K. U. Mayer (Ed.).Generationsdynamik in der Forschung. Frankfurt: Campus: 59–79.

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Institut für Soziologie, Universität München, Konradstr. 6, D-80801, München, (Germany)

    Nicole J. Saam

  2. Universitätsklinik für Tiefenpsychologie und Psychotherapie Wien, Universität Wien, Währinger Gürtel 18-20, A-1090, Wien, (Austria)

    L. Reiter

Authors
  1. Nicole J. Saam
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. L. Reiter
    View author publications

    You can also search for this author inPubMed Google Scholar

Rights and permissions

Reprints and permissions

About this article

Cite this article

Saam, N.J., Reiter, L. Lotka's law reconsidered: The evolution of publication and citation distributions in scientific fields. Scientometrics 44, 135–155 (1999). https://doi.org/10.1007/BF02457376

Download citation

  • Received:

  • Issue Date:

  • DOI: https://doi.org/10.1007/BF02457376

Keywords