The eigenvalues of random symmetric matrices

Abstract

LetA=(a _ij ) be ann ×n matrix whose entries fori≧j are independent random variables anda _ji =a _ij . Suppose that everya _ij is bounded and for everyi>j we haveEa _ij =μ,D ² a _ij =σ² andEa _ii =v.

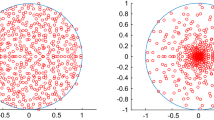
E. P. Wigner determined the asymptotic behavior of the eigenvalues ofA (semi-circle law). In particular, for anyc>2σ with probability 1-o(1) all eigenvalues except for at mosto(n) lie in the intervalI=(−c√n,c√n).

We show that with probability 1-o(1)all eigenvalues belong to the above intervalI if μ=0, while in case μ>0 only the largest eigenvalue λ₁ is outsideI, and

$$\lambda _1 = \frac{{\Sigma _{i,j} a_{ij} }}{n} + \frac{{\sigma ^2 }}{\mu } + O\left( {\frac{I}{{\sqrt n }}} \right)$$

i.e. λ₁ asymptotically has a normal distribution with expectation (n−1)μ+v+(σ²/μ) and variance 2σ² (bounded variance!).