Abstract
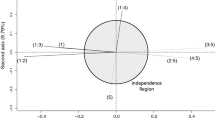
An exploration of the application of S-PLUS code designed to perform classical correspondence analysis is made in this paper. This code allows for the “classical” analysis to be performed on categorical data consisting of two or more variables. For multi-way contingency tables, correspondence analysis can be performed by considering either the indicator matrix or the Burt matrix. The code also allows the user to incorporate into the analysis confidence circles (to identify categorical responses that deviate from the hypothesis of independence) and for an asymmetrical analysis to be performed. The function includes various warnings and stoppages to help the user properly analyse their data.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
Beh, E. J. (2003), CORR: S-PLUS code for simple and multiple correspondence analysis (Version I), Research Report QMMS2003-04, School of Quantitative Methods and Mathematical Sciences, University of Western Sydney, Australia. Internet address:http://www.uws.edu.au/qmms/research/reports
Beh, E. J. (2004a), Simple correspondence analysis: A bibliographic review,International Statistical Review,72, 257–284.
Beh, E. J. (2005), S-PLUS code for ordinal correspondence analysis, Computational Statistics, (to appear).
Beh, E. J. and Davy, P. J. (1998), Partitioning Pearson’s chi-squared statistic for a completely ordered three-way contingency table,Australian and New Zealand Journal of Statistics,40, 465–477.
Beh, E. J. and Davy, P. J. (1999), Partitioning Pearson’s chi-squared statistic for a partially ordered three-way contingency table,Australian and New Zealand Journal of Statistics,41, 233–246.
Burt, C. (1950), The factorial analysis of qualitative data,Journal of Statistical Psychology,3, 166–185.
Carroll, J. D., Kumbasar, E. and Romney, K. (1997), An equivalence relation between correspondence analysis and classical metric multidimensional scaling for the recovery of Euclidean distances,British Journal of Mathematical and Statistical Psychology,50, 81–92.
Clogg, C. C. (1982), Some models for the analysis of association in multi-way cross-classifications having ordered categories,Journal of the American Statistical Association,77, 803–815.
Davis, J. A. (1977), Codebook for the 1977 General Social Survey, National Opinion Research Center, Chicago.
Everitt, B. S. (1994), A Handbook of Statistical Analysis using S-PLUS, Chapman and Hall, London.
Goodman, L. A. (1986), Some useful extensions of the usual correspondence analysis approach and the usual log-linear models approach in the analysis of contingency tables,International Statistical Review,54, 243–309.
Greenacre, M. J. (1984), Theory and Application of Correspondence Analysis, Academic Press, London.
Lebart, L., Morineau, A. and Warwick, L. (1984), Multivariate Descriptive Statistical Analysis, Wiley, New-York.
Srole, L., Langner, T. S., Michael, S. T., Opler M. K. and Rennie, T. A. C. (1962), Mental Health in the Metropolis: The Midtown Manhattan Study, McGraw-Hill, New-York.
Venables, W. N. and Ripley, B. D. (1999), Modern Applied Statistics with S-PLUS (Third Edition), Springer, New-York.
Weiler, S. C. and Romney, A. K. (1990), Metric Scaling: Correspondence Analysis, Sage University Paper Series on Quantitative Applications in the Social Sciences, 07–075. Newbury Park, CA: Sage.
Young, F. W. and Hamer, R. M. (1987), Multidimensional Scaling: History, Theory, and Applications, Hillsdale, NJ: Lawrence Erlbaum Associates.
Author information
Authors and Affiliations
Rights and permissions
About this article
Cite this article
Beh, E.J. S-PLUS code for simple and multiple correspondence analysis. Computational Statistics 20, 415–438 (2005). https://doi.org/10.1007/BF02741306
Published:
Issue Date:
DOI: https://doi.org/10.1007/BF02741306