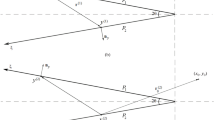
Abstract
In this work the scattering of plane waves from a finite sized perfectly conducting wedge as a function of its opening angle and the width of its faces is studied using the combination of physical optics (po) and the physical theory of diffraction (ptd). To find out under which circumstances the ptd contribution is significant compared to the PO, the ratio of the ptd field and the po field is evaluated as a maximum and a mean value over every direction of observation in the Keller cone, as well as in the special direction of backscattering. We employ the incremental length diffraction coefficients for a wedge with finite sized faces based on equivalent edge currents derived recently for truncated wedge strips. The numerical behaviour in the limiting cases of the diffraction coefficients are discussed extensively.
Résumé
Dans ce travail nous étudions la diffraction ďondes planes par un dièdre conducteur parfait, dont les dimensions des faces sont limitées, par une combinaison de ľoptique physique (po) et de la théorie physique de la diffraction (ptd) en fonction de ľangle du dièdre et de la largeur des faces. Afin de déterminer les conditions ďutilisation efficace de la ptd, les valeurs maximales et les valeurs moyennes du rapport du champ de la ptd au champ de la po sont évaluées pour chaque direction ďobservation dans le cône de Keller et dans la direction spéciale de rétrodiffusion. Nous employons les facteurs de diffraction incrémentaux du dièdre, fondé sur des expressions des courants de bord équivalents ďun dièdre fini récemment présentées. Le comportement numérique des facteurs de diffraction dans les cas limites est examiné en détail.
Similar content being viewed by others
References
Knott (E.F.),Shaffer (J. F.),Tuley (M.T.), Radar Cross Section,Artech House (1985);2 nd Ed.,Norwood (1993).
Shore (R.A.), Yaghjian (A.D.), Incremental Diffraction Coefficients for Planar Surfaces,ieee Trans. AP (1988),36, pp. 55–70.
Johansen (P.M.), Uniform physical theory of diffraction equivalent edge currents for truncated wedge strips,ieee Trans, AP (1996),44, n° 7, pp. 989–995.
Mitzer (K.M.), Incremental length diffraction coefficients, Techn. Rept. afal-TR-73-296, Northrop Corp., (1974).
Knott (E.F.), The relationship between Mitzner’s ildc and Michaeli’s equivalent currents,ieee Trans. AP (1985),33, pp. 112–114.
Michaeli (A.), Equivalent edge currents for arbitrary aspects of observation,ieee Trans, AP (1984),32, pp. 252–258.
Maffett (A.L.), Topicsfor a Statistical Description of Radar Cross Section, J. Wiley & Sons, (1989).
Michaeli (A.), Elimination of infinities in equivalent edge currents, Part 1: Fringe current components,ieee Trans. AP 1986, 34, pp. 912–918.
Michaeli (A.), Equivalent currents for second-order diffraction by the edges of perfectly conducting polygonal surfaces,ieee Trans. AP (1987), 35, pp. 183–190.
Senior (T.B.A.), Volakis (J. L.), Approximate boundary conditions in electromagnetics,iee Electromagnetic Waves Series,41, (1995).
Abramowitz (M.),Stegun (I.A.), Handbook of Mathematical Functions, Dover (1972).
Author information
Authors and Affiliations
Corresponding authors
Rights and permissions
About this article
Cite this article
Hujanen, A., Sten, J.C.E. Study of scattering by a perfectly conducting wedge with finite sized faces. Ann. Télécommun. 56, 587–594 (2001). https://doi.org/10.1007/BF03008835
Issue Date:
DOI: https://doi.org/10.1007/BF03008835
Key words
- Electromagnetic wave propagation
- Wave diffraction
- Wedge
- Perfect conduction
- Plane wave
- Wave optics
- Backscattering