Abstract
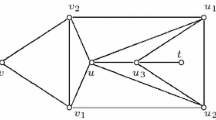
A graph G is called Berge if neither G nor its complement contains a chordless cycle with an odd number of nodes. The famous Berge’s Strong Perfect Graph Conjecture asserts that every Berge graph is perfect. A chair is a graph with nodes {a, b, c, d, e} and edges {ab, bc, cd}, eb. We prove that a Berge graph with no induced chair (chair-free) is perfect or, equivalently, that the Strong Perfect Graph Conjecture is true for chair-free graphs.
Similar content being viewed by others
References
Berge, C.: Färbung von Graphen, deren sämlitche bzw, ungerade Kreise starr sind (Zusammenfassung), Wiss, Z. Mar-tin Luther Univ. Halle Wittenberg, Math. Nat. Reihe (1961) 114.
Berge, C., Chvátal, V. Eds.: Topics on Perfect Graphs. Annals of Discrete Mathematics, Vol 21 Amsterdam: North Holland 1984
Conforti, M., Cornuéjols, G., Kapoor, A., Vušković, K.: A Mickey Mouse Decomposition Theorem, Proceedings IPCO IV, Copenhagen, (May 1995)
Chvátal, V.: On certain polytopes associated with graphs. J. Comb. Theory ser. B 18, 138–154 (1975)
Chvátal, V.: Star cutsets and perfect graphs. J. Comb. Theory ser. B 39, 189–299 (1985)
Chvátal, V., Sbihi, N.: Bull-free Berge graphs are perfect. Graphs and Comb. 3, 127–139 (1987)
De Simone, C., Galluccio, A.: New classes of Berge perfect graphs. Discrete Math. 131, 67–79 (1994)
De Simone, C., Sassano, A.: Stability Number of Bull and Chair Free Graphs. Discrete Appl. Math. 41, 121–129 (1993)
Galluccio, A., Sassano, A.: The rank facets of the stable set polytope for claw-free graphs. Technical Report n. 340, IASI-CNR, Rome (1994) J. Comb. Theory ser. B (1997) (to be published)
Lovász, L.: Normal Hypergraphs and the perfect graph conjecture. Discrete Math. 2, 253–267 (1972)
Lovász, L.: A Characterization of Perfect Graphs, J. Comb. Theory ser. B 13, 95–98 (1972)
Lovász, L., Plummer, M.: Matching Theory. Annals of Discrete Mathematics, Vol 29 Amsterdam: North Holland 1986
Mannino, C., Sassano, A.: Edge Projection and the Maximum Cardinality Stable Set Problem. In: D.S. Johnson, M.A. Trick: Cliques, Coloring, and Satisfiability: Second DIMACS Implementation Challenge. DIMACS Series in Discrete Mathematics and Theoretical Computer Science (to be published)
Olariu, S.: On the strong perfect graph conjecture. J. Graph Theory 12, 169–176 (1988)
Olariu, S.: Paw-free graphs. Information Processing Letters 28, 53–54 (1988)
Olariu, S.: The Strong Perfect Graph Conjecture for Pan-free graphs, J. Comb. Theory ser. B 47, 187–191 (1989)
Padberg, M.W.: Perfect zero-one Matrices. Mathematical Programming, 6, 180–196 (1974)
Parthasarathy, K.R., Ravindra, G.: The strong perfect graph conjecture is true for (K 1, 3)-free graphs. J. Comb. Theory ser. B 21 212–223 (1976)
Parthasarathy, K.R., Ravindra, G.: The validity of the strong perfect graph conjecture for (K 4 − e)-free graphs. J. Comb. Theory ser. B 26, 98–100 (1979)
Sassano, A.: Reducible cliques and the Strong Perfect Graph Conjecture, IASI Technical Report R.257, (March 1989)
Sebő, A.: On critical edges in minimal imperfect graphs. Proceedings IPCO III, Erice (May 1993).
Seinsche, D.: On a property of the class of n-colorable graphs. J. Comb. Theory ser. B 16, 191–193 (1974)
Sun, Liping: Two classes of perfect graphs. J. Comb. Theory ser. B 53, 273–292 (1991)
Tucker, A.: Coloring perfect (K 4 − e)-free graphs. J. Comb. Theory ser. B 42, 313–318 (1987).
Tucker, A.: Critical perfect graphs and perfect 3-chromatic graphs. J. Comb. Theory ser. B 23, 143–149 (1977)
Author information
Authors and Affiliations
Corresponding author
Additional information
This work was partially supported by MURST, Roma, Italy and Progetto Finalizzato Trasporti II, CNR, Italy
Rights and permissions
About this article
Cite this article
Sassano, A. Chair-Free Berge Graphs Are Perfect. Graphs and Combinatorics 13, 369–395 (1997). https://doi.org/10.1007/BF03353015
Received:
Revised:
Published:
Issue Date:
DOI: https://doi.org/10.1007/BF03353015