Analysis of a class of quasi-monotone and conservative semi-Lagrangian advection schemes

Summary.

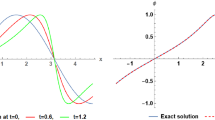
We analyze in the $L^\infty-$norm a class of semi-Lagrangian advective schemes introduced by the author and A. Staniforth in 1992 to improve the solution produced by numerical models for weather prediction and climate studies that use semi-Lagrangian advective schemes. The new quasi-monotone and conservative semi-Lagrangian schemes are $L^\infty-$stable and converge optimally when the solution is sufficiently smooth.