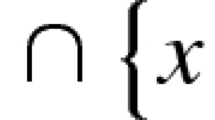Skip to main content
Springer Nature Link
Log in

The packing property

  • Published:
Mathematical Programming Submit manuscript

Abstract.

A clutter (V, E) packs if the smallest number of vertices needed to intersect all the edges (i.e. a minimum transversal) is equal to the maximum number of pairwise disjoint edges (i.e. a maximum matching). This terminology is due to Seymour 1977. A clutter is minimally nonpacking if it does not pack but all its minors pack. An m×n 0,1 matrix is minimally nonpacking if it is the edge-vertex incidence matrix of a minimally nonpacking clutter. Minimally nonpacking matrices can be viewed as the counterpart for the set covering problem of minimally imperfect matrices for the set packing problem. This paper proves several properties of minimally nonpacking clutters and matrices.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

Author information

Authors and Affiliations

  1. GSIA, Carnegie Mellon Univ., Pittsburgh PA 15213, USA, , , , , , US

    Gérard Cornuéjols

  2. Faculty of Mathematics, Department of Combinatorics and Optimization, University of Waterloo, Waterloo, Ontario, Canada N2L 3G1, , , , , , CA

    Bertrand Guenin

  3. Department of Mathematics, University of Kentucky, Lexington KY, USA, , , , , , US

    François Margot

Authors
  1. Gérard Cornuéjols
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Bertrand Guenin
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. François Margot
    View author publications

    You can also search for this author inPubMed Google Scholar

Additional information

Received: December 1, 1997 / Accepted: April 6, 1999¶Published online October 18, 2000

Rights and permissions

Reprints and permissions

About this article

Cite this article

Cornuéjols, G., Guenin, B. & Margot, F. The packing property. Math. Program. 89, 113–126 (2000). https://doi.org/10.1007/PL00011389

Download citation

  • Issue Date:

  • DOI: https://doi.org/10.1007/PL00011389