On a Non-linear Electronic Circuit Filtering

Abstract

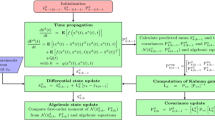
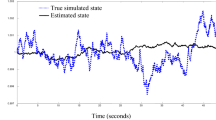
The stochastic versions of non-linear dynamic circuits are formalized using non-linear stochastic differential equations. Stochastic differential equations (SDEs) are exploited to analyse dynamical systems in noisy environments. A potential application of the SDEs can be regarded as ‘stochastic processes in electronic circuits’. The noisy sampling mixer, a component of digital wireless communications, is an appealing and standard case from the dynamical systems’ viewpoint. It assumes the structure of a non-linear SDE, and its linearized version becomes time-varying bilinear SDE. This paper derives the filtering equations for the noisy non-linear sampling mixer circuit utilizing the filtering density evolution equation. The filtering model for the stochastic problem of concern here comprises the following: (1) a non-linear SDE describing the noisy sampling mixer and (2) a non-linear noisy observation equation. It is interesting to note that the filtered estimate accounts for observations. On the other hand, the predicted estimate does not account for the observation terms in evolution equations. As a result of this, the filtered estimate confirms the greater accuracy of estimated state trajectory in contrast to the predicted trajectory. The filtering equation of this paper can be further utilized for control of the noisy sampling mixer, where the observations are available.

Appendix

From the system-theoretic viewpoint, Eq. (4) of the paper, which is stated as Eq. (7) of Yu and Leung [20], is regarded as a time-varying scalar ‘bilinear’ stochastic differential system. Consider a structure of a bilinear time-varying stochastic differential equation,

$$\begin{aligned} \hbox {d}x_t =(\mu _t +\alpha _t x_t )\hbox {d}t+(\beta _t +\gamma _t x_t )\hbox {d}B_t , \end{aligned}$$

where $B_t$ is the Brownian motion process, a stochastic process. The parameter vector $\left( {\mu _{t,} \alpha _t ,\beta _t ,\gamma _t } \right) ^{T}$ of the above stochastic differential equation is time varying, and the term $\gamma _t x_t \hbox {d}B_t$ denotes the input-state time-varying coupling term. The input-state coupling term leads to the notion of bilinearity, see the last term of Eq. (4). The bilinear system is a nearly linear system yet non-linear system (Bruni et al. [1]). Bilinear systems have found applications in population models, communications, echo cancellations, electrical circuits and electronic circuits under noise influence. Bilinear systems make a connection to the Volterra theory (Schetzen [15]), see Lee and Mathew [8] as well. Here, the Authors explain briefly a time-varying Itô bilinear stochastic differential equation as well as Itô bilinear stochastic differential equation with constant coefficients.

A sampling mixer time-varying Itô bilinear SDE is

$$\begin{aligned} \hbox {d}x_t= & {} (\mu _t +\alpha _t x_t )\hbox {d}t+(\beta _t +\gamma _t x_t ) \hbox {d}B_t\nonumber \\= & {} \left( \frac{g_t }{C}u_t -\frac{g_t }{C}x_t \right) \hbox {d}t+\left( \frac{g_t }{C}-\frac{g_t }{C} x_t \right) \hbox {d}B_t . \end{aligned}$$

(25)

The closed-form solution to the above SDE can be obtained using the solution stated in Pugachev and Sinitsyn ([14], pp. 181–182).

Thus

$$\begin{aligned} x_t= & {} x_{t_0 } \exp \left( \int \limits _{t_0 }^t \left( \alpha _\tau -\frac{\gamma _\tau ^2 }{2} \right) \hbox {d}\tau +\int \limits _{t_0 }^t {\gamma _\tau } \hbox {d}B_\tau \right) +\int \limits _{t_0 }^t {\mu _\tau \exp } \left( \int \limits _\tau ^t \left( \alpha _s -\frac{\gamma _s^2 }{2}\right) \hbox {d}s\right. \nonumber \\&\left. +\int \limits _\tau ^t {\gamma _s \hbox {d}B_s } \right) \hbox {d}\tau +\int \limits _{t_0 }^t {\beta _\tau \exp } \left( \int \limits _\tau ^t {\left( \alpha _s -\frac{\gamma _s^2 }{2}\right) \hbox {d}s+\int \limits _\tau ^t {\gamma _s \hbox {d}B_s } } \right) \hbox {d}B_\tau . \end{aligned}$$

(26)

From Eqs. (25) and (26), we get

$$\begin{aligned} x_t= & {} x_{t_0 } \exp \left( \int _{t_0 }^t \left( \alpha _\tau -\frac{\gamma _\tau ^2 }{2} \right) \hbox {d}\tau +\int _{t_0 }^t {\gamma _\tau } \hbox {d}B_\tau \right) +\int _{t_0 }^t {\mu _\tau \exp } \left( \int _\tau ^t \left( \alpha _s -\frac{\gamma _s^2 }{2}\right) \hbox {d}s\right. \\&\left. +\int _\tau ^t {\gamma _s \hbox {d}B_s } \right) \hbox {d}\tau +\int _{t_0 }^t {\beta _\tau \exp } \left( \int _\tau ^t {\left( \alpha _s -\frac{\gamma _s^2 }{2}\right) \hbox {d}s+\int _\tau ^t {\gamma _s \hbox {d}B_s } } \right) \hbox {d}B_\tau \\= & {} x_{t_0 } \exp \left( \int _{t_0 }^t \left( -\frac{g_\tau }{C}-\frac{g_\tau ^2 }{2C^{_2 }} \right) \hbox {d}\tau -\int _{t_0 }^t {\frac{g_\tau }{C}} \hbox {d}B_\tau \right) \\&+\int _{t_0 }^t {\frac{g_\tau }{C}u_\tau \exp } \left( \int _\tau ^t {\left( -\frac{g_\mathrm{s} }{C}-\frac{g_\mathrm{s}^2 }{2C^{2}}\right) \hbox {d}s-\int _\tau ^t {\frac{g_\mathrm{s} }{C}\hbox {d}B_\mathrm{s} } } \right) \hbox {d}\tau .\\&+\int _{t_0 }^t {\frac{g_\tau }{C}\exp } \left( \int _\tau ^t {\left( -\frac{g_\mathrm{s} }{C}-\frac{g_\mathrm{s}^2 }{2C^{2}}\right) \hbox {d}s-\int _\tau ^t {\frac{g_\mathrm{s} }{C}\hbox {d}B_\mathrm{s} } } \right) \hbox {d}B_\tau . \end{aligned}$$

For constant coefficients, Eq. (26) becomes

$$\begin{aligned} x_t= & {} x_{t_0 } \exp \left( \left( \alpha -\frac{\gamma ^{2}}{2}\right) (t-t_0 )+\gamma (B_t -B_{t_0 } )\right) +\mu \int _{t_0 }^t \exp \left( \left( \alpha -\frac{\gamma ^{2}}{2}\right) (t-\tau )\right. \nonumber \\&\left. +\;\gamma (B_t -B_\tau )\right) \hbox {d}\tau .\nonumber \\&+\;\beta \int _{t_0 }^t \exp \left( \left( \alpha -\frac{\gamma ^{2}}{2}\right) (t-\tau )+\gamma (B_t -B_\tau )\right) \hbox {d}B_\tau . \end{aligned}$$

(27)

The term within the last integral sign of the right-hand side of the above can be further simplified using stochastic differential rule, i.e.

$$\begin{aligned}&\exp \left( \left( \alpha -\frac{\gamma ^{2}}{2}\right) (t-\tau )+\gamma (B_t -B_\tau )\right) \hbox {d}B_\tau \nonumber \\&\quad =\exp \left( \left( \alpha -\frac{\gamma ^{2}}{2}\right) (t-\tau )\right) \exp \left( \gamma (B_t -B_\tau )\right) \hbox {d}B_\tau ,\nonumber \\&\quad =\exp \left( \left( \alpha -\frac{\gamma ^{2}}{2}\right) (t-\tau )\right) \left( 1+\gamma B_t -\gamma B_\tau +O((B_t -B_\tau )^{2})\right) dB_\tau ,\nonumber \\&\quad =\exp \left( \left( \alpha -\frac{\gamma ^{2}}{2}\right) (t-\tau )\right) (1+\gamma B_t -\gamma B_{t+d\tau } +\gamma B_{t+d\tau } -\gamma B_\tau \nonumber \\&\qquad +\,O((B_t -B_\tau )^{2}))\hbox {d}B_\tau ,\nonumber \\&\quad =\exp \left( \left( \alpha -\frac{\gamma ^{2}}{2}\right) (t-\tau )\right) (\hbox {d}B_\tau +\gamma \hbox {d}\tau ). \end{aligned}$$

(28)

From Eq. (27) in combination with (28), we get

$$\begin{aligned} x_t= & {} x_{t_0 } \exp \left( \left( \alpha -\frac{\gamma ^{2}}{2}\right) (t-t_0 )+\gamma (B_t -B_{t_0 } )\right) \\&+\; \mu \int _{t_0 }^t \exp \left( \left( \alpha -\frac{\gamma ^{2}}{2}\right) (t-\tau )+\gamma (B_t -B_\tau )\right) \hbox {d}\tau \\&+\; \beta \int _{t_0 }^t \exp \left( \left( \alpha -\frac{\gamma ^{2}}{2}\right) (t-\tau )\right) \hbox {d}B_\tau +\beta \gamma \int _{t_0 }^t \exp \left( \left( \alpha -\frac{\gamma ^{2}}{2}\right) (t-\tau )\right) \hbox {d}\tau . \end{aligned}$$

For the simplified analysis, consider $B_{t_0} =0$ and $t_0 =0$, we have

$$\begin{aligned} x_t= & {} x_0 \exp \left( \left( \alpha -\frac{\gamma ^{2}}{2}\right) t+\gamma B_t \right) +\mu \int _0^t \exp \left( \left( \alpha -\frac{\gamma ^{2}}{2}\right) (t-\tau )+\gamma (B_t -B_\tau )\right) d\tau \nonumber \\&+\;\beta \int _0^t \exp \left( \left( \alpha -\frac{\gamma ^{2}}{2}\right) (t-\tau )\right) \hbox {d}B_\tau + \beta \gamma \int _0^t \exp \left( \left( \alpha -\frac{\gamma ^{2}}{2}\right) (t-\tau )\right) \hbox {d}\tau .\nonumber \\ \end{aligned}$$

(29)

After taking the action of conditional expectation operator, we get

$$\begin{aligned} \left\langle {x_t } \right\rangle =\left\langle {x_0 } \right\rangle \exp (\alpha t)-\frac{\mu }{\alpha }(1-\exp (\alpha t))-\frac{\beta \gamma }{\alpha -\frac{\gamma ^{2}}{2}}\left( 1-\exp \left( \alpha -\frac{\gamma ^{2}}{2}\right) t\right) .\qquad \quad \end{aligned}$$

(30)

The conditional mean trajectory $\langle {x_t}\rangle$ would be bounded for $\alpha <0$. At $\alpha <0$, the mean trajectory $\left\langle {x_t } \right\rangle =\left\langle {x_0 } \right\rangle \exp (\alpha t)$ would be bounded. Equation (30) is quite general that will be useful for the noise analysis of scalar bilinear stochastic differential systems arising from diverse fields, e.g. noisy non-linear electronic circuits.

Another important point to worth mention is that the power spectral density $S_{xx} (\omega )=S_{v_\mathrm{d} v_\mathrm{d} } (\omega )$ of the output process can be obtained by exploiting Eq. (29) and the relation

$$\begin{aligned} S_{v_\mathrm{d} v_\mathrm{d} } (\omega )=\mathfrak {I}R_{v_\mathrm{d} v_\mathrm{d} } (\tau )= \mathfrak {I}\left\langle {v_\mathrm{d} (t+\tau )v_\mathrm{d} (t)} \right\rangle . \end{aligned}$$

Note that the notations $\mathfrak {I}$ and $\langle \,\rangle$ denote the Fourier transform operator and the expectation operator, respectively. The notation $R_{v_\mathrm{d} v_\mathrm{d} } (\tau )$ denotes the autocorrelation of a wide-sense stationary output process. The sampling mixer SDE of this paper, Eq. (3), is non-linear and time varying; the closed-form solution to the output drain voltage $v_\mathrm{d}$ in terms of the gate noise $\xi _t$ is not possible. Thus, the closed-form expression for the power spectral density $S_{v_\mathrm{d} v_\mathrm{d} } (\omega )=\mathfrak {I}R_{v_\mathrm{d} v_\mathrm{d} } (\tau )=\mathfrak {I}\left\langle {v_\mathrm{d} (t+\tau )v_\mathrm{d} (t)} \right\rangle$ is intractable.