Abstract
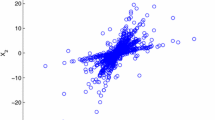
This paper considers the mixing matrix estimation in the underdetermined blind source separation. An effective estimation algorithm based on local directional density detection (LDDD) and dynamic data field clustering (DDFC) is proposed. First, argument-based time-frequency single source points detection is employed to improve signal sparsity. To overcome the limitation of traditional clustering algorithms, which depend on the preset of initial clustering centers and the number of sources, the LDDD is introduced to choose the single source points with high potential energy as representative objects to form data preliminary classification. Then DDFC algorithm is adopted to move and merge the representative objects until all column vectors of mixing matrix are estimated. Simulation results show that the proposed method can effectively estimate mixing matrix with high accuracy, especially in the real non-cooperative cases where the number of sources is unknown.









Similar content being viewed by others
References
F. Abrard, Y. Deville, A time-frequency blind signal separation method applicable to underdetermined mixtures of dependent sources. Signal Process. 85, 1389–1403 (2005)
M. Aharon, M. Elad, A. Bruckstein, K-SVD an algorithm for designing overcomplete dictionaries for sparse representation. IEEE Trans. Signal Process. 54(11), 4311–4322 (2006)
G. Bao, Z. Ye, X. Xu et al., A compressed sensing approach to blind separation of speech mixture based on a two-layer sparsity model. IEEE Trans. Audio Speech Lang. Process. 21(5), 899–906 (2013)
P. Bofill, M. Zibulevsky, Underdetermined blind source separation using sparse representations. Signal Process. 81(11), 2353–2362 (2001)
T. Dong, Y. Lei, J. Yang, An algorithm for underdetermined mixing matrix estimation. Neurocomputing 104(15), 26–34 (2013)
P. Georgiev, F. Theis, A. Cichocki, Sparse component analysis and blind source separation of underdetermined mixtures. IEEE Trans. Neural Netw. 16(4), 992–996 (2005)
G. Giachetta, L. Mangiarotti, G. Sardanashvily, Advanced Classical Field Theory (World Scientific, Singapore, 2009)
Z. He, A. Cichocki, K-hyperline clustering learning for sparse component analysis. Signal Process. 89(6), 1011–1022 (2009)
A. Jourjine, S. Rickard, Blind separation of disjoint orthogonal signals: demixing N sources from 2 mixtures. Acoust. Speech Signal Process. ICASSP 5, 2985–2988 (2000)
B. Kante, D. Germain, A. de Lustrac, Near field imaging of refraction via the magnetic field. Appl. Phys. Lett. 104(2), 021909–021909.3 (2014)
S.G. Kim, C.D. Yoo, Underdetermined blind source separation based on subspace representation. IEEE Trans. Signal Process. 57(7), 2604–2614 (2009)
Y. Li, S.I. Amari, A. Cichocki et al., Underdetermined blind source separation based on sparse representation. IEEE Trans. Signal Process. 54(2), 423–437 (2006)
Y. Li, A. Cichocki, S.I. Amari, Analysis of sparse representation and blind source separation. Neural Comput. 16(6), 1193–1234 (2004)
Y. Li, W. Nie, F. Ye, A complex mixing matrix estimation algorithm based on single source points. Circuits Syst. Signal Process. 34(11), 3709–3723 (2015)
F. Nainia, G. Mohimania et al., Estimating the mixing matrix in sparse component analysis (SCA) based on partial k-dimensional subspace clustering. Neurocomputing 71, 2330–2343 (2008)
L.T. Nguyen, A. Belouchrani, K. Abed-Meraim, Separating more sources than sensors using time-frequency distributions. EURASIP J. Appl. Signal Process. 17, 2828–2847 (2005)
T. Peng, Y. Chen, Z. Liu, A time-frequency domain blind source separation method for underdetermined instantaneous mixtures. Circuits Syst. Signal Process. 34(12), 3883–3895 (2015)
M. Puigt, Y. Deville, Time-frequency ratio-based blind separation methods for attenuated and time-delayed sources. Mech. Syst. Signal Process. 19(6), 1348–1379 (2005)
V.G. Reju, S.N. Koh, I.Y. Soon, An algorithm for mixing matrix estimation in instantaneous blind source separation. Signal Process. 89(9), 1762–1773 (2009)
J. Sun, Y. Li, J. Wen, S. Yan, Novel mixing matrix estimation approach in underdetermined blind source separation. Neurocomputing 173, 623–632 (2016)
J.J. Thiagarajan, K.N. Ramamurthy, A. Spanias, Mixing matrix estimation using discriminative clustering for blind source separation. Digital Signal Process. 23(1), 9–18 (2013)
S. Wang, W. Gan, D.Y. Li, D.R. Li, Data field for hierarchical clustering. Int. J. Data Warehous. Min. 7(4), 43–63 (2011)
S. Xie, L. Yang, J. Yang et al., Time-frequency approach to underdetermined blind source separation. IEEE Trans. Syst. Neural Netw. Learn. 23(2), 306–316 (2012)
J. Xu, X. Yun, D. Hu et al., A fast mixing matrix estimation method in the wavelet domain. Signal Process. 95, 58–66 (2014)
J. Yang, H. Liu, Blind identification of the underdetermined mixing matrix based on K-weighted hyperline clustering. Neurocomputing 149(PB), 483–489 (2015)
O. Yilmaz, S. Rickard, Blind separation of speech mixture via time-frequency masking. IEEE Trans. Signal Process. 52(7), 1830–1847 (2004)
H. Zhang, G. Wang, P. Cai et al., A fast blind source separation algorithm based on the temporal structure of signals. Neurocomputing 139(9), 261–271 (2014)
L. Zhang, J. Yang, K. Lu et al., Modified subspace method based on convex model for underdetermined blind speech separation. IEEE Trans. Consum. Electron. 60(2), 225–232 (2014)
Acknowledgements
This work is supported by the National Natural Science Foundation of China (No. 61371172), the International S&T Cooperation Program of China (ISTCP) (No. 2015DFR10220), the Ocean Engineering Project of the National Key Laboratory Foundation (No. 1213), the Fundamental Research Funds for the Central Universities (No. HEUCF1508), the Natural Science Foundation of Heilongjiang Province (No. F201337).
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Guo, Q., Ruan, G. & Nan, P. Underdetermined Mixing Matrix Estimation Algorithm Based on Single Source Points. Circuits Syst Signal Process 36, 4453–4467 (2017). https://doi.org/10.1007/s00034-017-0522-9
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00034-017-0522-9