A model of parallel contests

Abstract

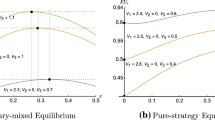
We develop a model of two parallel contests, asymmetric in quantity of homogeneous prizes open to contest, with a finite number of homogeneous risk-neutral bidders. Whether the bidder upon entry into a particular contest is aware of the realized number of competing contestants in the contest is irrelevant to the expected effort at equilibrium. At equilibrium the expected effort per capita in the larger contest (the contest with more prizes) is greater than that in the smaller one. The larger contest nonetheless does not attract enough contestants to achieve optimum in rent extraction from the bidders.

Appendix: Proof of the lemmas and propositions

Proof of Lemma 1

Take contest $A$ and suppose the agent under consideration enters $A$. At the intra-contest contest equilibrium, as is typical of the all-pay auction game, zero effort stands as one element of the continuum of effort of the agent in the mixed equilibrium. Note that any player loses to the agent when the latter exerts effort $e,$. either by going elsewhere (with probability $1 - \alpha$) or coming along but exerting less effort than ${\text{e}}$ (with probability $\alpha {\text{F}}\left( {\text{e}} \right),$ where $F$ the cumulative probability function of effort ${\text{e}}$). For the agent to win a prize in contest A by making effort ${\text{e}}$ therein, he loses no more than $q_{A} - 1$ players as such, an event that occurs with probability $\mathop \sum \limits_{{{\text{t}} = 0}}^{{q_{A} - 1}} \left( {\begin{array}{*{20}c} {{\text{n}} - 1} \\ {\text{t}} \\ \end{array} } \right)\left[ {1 - \left( {1 - \alpha + \alpha {\text{F}}\left( {\text{e}} \right)} \right)} \right]^{\text{t}} \left[ {1 - \alpha + \alpha {\text{F}}\left( {\text{e}} \right)} \right]^{{{\text{n}} - 1 - {\text{t}}}}$, hence for any possible effort ${\text{e}}$, his net payoff $\pi \left( e \right) = \pi \left( 0 \right)$, i.e.,

$$ v \cdot \mathop \sum \limits_{{{\text{t}} = 0}}^{{q_{A} - 1}} \left( {\begin{array}{*{20}c} {{\text{n}} - 1} \\ {\text{t}} \\ \end{array} } \right)\left[ {1 - \left( {1 - \alpha + \alpha {\text{F}}} \right)} \right]^{\text{t}} \left( {1 - \alpha + \alpha {\text{F}}} \right)^{{{\text{n}} - 1 - {\text{t}}}} - {\text{ce}} = v \cdot { \Pr }\left( {s_{A} \le q_{A} - 1;\alpha ,n - 1} \right)$$

Multiply by the density probability function $f\left( e \right)$ both sides of the above and integrate, $v \cdot \mathop \int \limits_{0}^{1} \mathop \sum \limits_{{{\text{t}} = 0}}^{{q_{A} - 1}} \left( {\begin{array}{*{20}c} {{\text{n}} - 1} \\ {\text{t}} \\ \end{array} } \right)\left[ {1 - \left( {1 - \alpha + \alpha {\text{F}}} \right)} \right]^{\text{t}} \left( {1 - \alpha + \alpha {\text{F}}} \right)^{{{\text{n}} - 1 - {\text{t}}}} dF - c\overline{{e_{A} }} = v \cdot { \Pr }\left( {s_{A} \le q_{A} - 1;\alpha ,n - 1} \right)$. It thus suffices to show

$$ \begin{aligned} & \mathop \sum \limits_{{{\text{t}} = 0}}^{{q_{A} - 1}} \left( {\begin{array}{*{20}c} {{\text{n}} - 1} \\ {\text{t}} \\ \end{array} } \right)\mathop \int \limits_{0}^{1} \left[ {1 - \left( {1 - \alpha + \alpha {\text{F}}} \right)} \right]^{\text{t}} \left( {1 - \alpha + \alpha {\text{F}}} \right)^{{{\text{n}} - 1 - {\text{t}}}} dF \\ & \quad = \frac{{q_{A} \mathop \sum \nolimits_{{s_{A} \ge q_{A} + 1}} \Pr \left( {s_{A} ;\alpha ,n} \right) + \mathop \sum \nolimits_{{s_{A} \le q_{A} }} s_{A} \Pr \left( {s_{A} ;\alpha ,n} \right)}}{n\alpha } \\ \end{aligned} $$

(5)

We invoke induction over $q_{A}$ to establish Eq. (5). When $q_{A} = 1,$ it can be readily shown that both sides of Eq. (5) equal $\frac{{1 - \left( {1 - \alpha } \right)^{n} }}{n\alpha }$. Now assume that Eq. (5) holds for $q_{A}$ and consider the case when there are $(q_{A} + 1)$ prizes in contest A. Letting $\sigma = \alpha \left( {1 - {\text{F}}} \right),$ we obtain $\left( {\begin{array}{*{20}c} {{\text{n}} - 1} \\ {q_{A} } \\ \end{array} } \right)\mathop \int \limits_{0}^{1} \left[ {1 - \left( {1 - \alpha + \alpha {\text{F}}} \right)} \right]^{{q_{A} }} \left( {1 - \alpha + \alpha {\text{F}}} \right)^{{{\text{n}} - 1 - q_{A} }} dF = \frac{1}{\alpha }\left( {\begin{array}{*{20}c} {{\text{n}} - 1} \\ {q_{A} } \\ \end{array} } \right)\mathop \int \limits_{0}^{\alpha } \sigma^{{q_{A} }} \left( {1 - \sigma } \right)^{{n - 1 - q_{A} }} {\text{d}}\sigma = \frac{{q_{A} + 1}}{{{\text{n}}\alpha }}\left( {\begin{array}{*{20}c} {\text{n}} \\ {q_{A} + 1} \\ \end{array} } \right)\mathop \int \limits_{0}^{{1 - \left( {1 - \alpha } \right)}} \sigma^{{q_{A} }} \left( {1 - \sigma } \right)^{{n - q_{A} - 1}} {\text{d}}\sigma ,$ which, in turn, by the well-known incomplete Beta functional form of the binomial distribution, equals $\frac{{\mathop \sum \nolimits_{{s_{A} \ge q_{A} + 1}} \Pr \left( {s_{A} ;\alpha ,n} \right)}}{n\alpha }.$ Therefore, LHS of Eq. (5) when there are $(q_{A} + 1)$ prizes in contest A equals $\frac{{\left( {q_{A} + 1} \right)\mathop \sum \nolimits_{{s_{A} \ge q_{A} + 2}} \Pr \left( {s_{A} ;\alpha ,n} \right) + \mathop \sum \nolimits_{{s_{A} \le q_{A} + 1}} s_{A} \Pr \left( {s_{A} ;\alpha ,n} \right)}}{n\alpha },$ equaling its RHS thereof.$\hfill \square$

Proof of Proposition 1

In the case that the number of bidders in a contest is known to the bidder after he enters it (say contest $A$), his effort cost will be zero if the number of participants (excluding himself) is less than $q_{A}$, and equal the expected gross surplus $\sum\nolimits_{{s_{A} \ge q_{A} }} {\frac{{vq_{A} }}{{s_{A} + 1}}} { \Pr }\left( {s_{A} ;\alpha ,n - 1} \right)$ otherwise. Thus, the equilibrium probability $\alpha$ with which the bidder goes to contest A is such that $f\left( {\alpha , n} \right) \equiv Pr\left( {s_{A} \le q_{A} - 1; \alpha , n - 1} \right) - Pr\left( {s_{B} \le q_{B} - 1;\beta , n - 1} \right) = 0,$ the same as that when the realized number of contestants in contest $A$ remains unknown to him, and the expected effort cost equals $\sum\nolimits_{{s_{A} \ge q_{A} }} {\frac{{vq_{A} }}{{s_{A} + 1}}} { \Pr }\left( {s_{A} ;\alpha ,n - 1} \right) = \frac{{vq_{A} }}{n\alpha }\sum\nolimits_{{t \ge q_{A} + 1}} { \Pr } \left( {t;\alpha ,n} \right)$, the same as that when the said realized number of contestants is unknown to him.$\hfill \square$

Proof of Lemma 2

Note $\beta Pr\left( {s_{A} = q_{A} ; \alpha , n - 1} \right) < \alpha Pr\left( {s_{B} = q_{B} ; \beta , n - 1} \right)$ amounts to

$$ \left( {\begin{array}{*{20}c} {n - 1} \\ {q_{A} } \\ \end{array} } \right)\alpha^{{q_{A} }} \left( {1 - \alpha } \right)^{{n - q_{A} }} < \left( {\begin{array}{*{20}c} {n - 1} \\ {q_{B} } \\ \end{array} } \right)\left( {1 - \alpha } \right)^{{q_{B} }} \alpha^{{n - q_{B} }} $$

(6)

which apparently holds when $n = q_{A} + q_{B} or q_{A} + q_{B} + 1$ since $\alpha > \frac{1}{2}$. When $n > q_{A} + q_{B} + 1,$$f\left( {\frac{1}{2},n} \right) > 0$ and $\frac{\partial }{\partial \alpha }f\left( {\alpha ,n} \right) <{0}, \forall \alpha >{0}$ Let $g\left( \alpha \right) \equiv \frac{{\left( {\begin{array}{*{20}c} {n - 1} \\ {q_{A} } \\ \end{array} } \right)\alpha^{{q_{A} }} \left( {1 - \alpha } \right)^{{n - q_{A} }} }}{{\left( {\begin{array}{*{20}c} {n - 1} \\ {q_{B} } \\ \end{array} } \right)\left( {1 - \alpha } \right)^{{q_{B} }} \alpha^{{n - q_{B} }} }} - 1.$ Then $g\left( {\frac{1}{2}} \right) > 0$ and $g^{\prime}\left( \alpha \right) = \left[ {g\left( \alpha \right) + 1} \right] \cdot \frac{{q_{A} + q_{B} - n}}{{\alpha \left( {1 - \alpha } \right)}} < 0,$$\forall \alpha > 0.$ Claim (6) holds if and only if for $\alpha$ satisfying $g\left( \alpha \right) = 0,$ i.e., $\left( {\frac{\alpha }{1 - \alpha }} \right)^{{n - q_{A} - q_{B} }} = \frac{{\left( {\begin{array}{*{20}c} {n - 1} \\ {q_{A} } \\ \end{array} } \right)}}{{\left( {\begin{array}{*{20}c} {n - 1} \\ {q_{B} } \\ \end{array} } \right)}}$, $f\left( {\alpha ,n} \right) > 0$ holds. Note $\alpha > 1/2$. To ensure $f\left( {\alpha ,n} \right) > 0,$ it suffices to show that $\forall k \in \left\{ {1, \ldots ,q_{B} } \right\},$$\left( {\begin{array}{*{20}c} {n - 1} \\ {q_{A} - k} \\ \end{array} } \right)\alpha^{{q_{A} - k}} \left( {1 - \alpha } \right)^{{n - 1 - (q_{A} - k)}} > \left( {\begin{array}{*{20}c} {n - 1} \\ {q_{B} - k} \\ \end{array} } \right)\left( {1 - \alpha } \right)^{{q_{B} - k}} \alpha^{{n - 1 - \left( {q_{B} - k} \right)}} ,$ which holds iff $\frac{{\left( {\begin{array}{*{20}c} {n - 1} \\ {q_{A} - k} \\ \end{array} } \right)}}{{\left( {\begin{array}{*{20}c} {n - 1} \\ {q_{B} - k} \\ \end{array} } \right)}} > \left( {\frac{\alpha }{1 - \alpha }} \right)^{{n - q_{A} - q_{B} + 2k - 1}} = \frac{{\left( {\begin{array}{*{20}c} {n - 1} \\ {q_{A} } \\ \end{array} } \right)}}{{\left( {\begin{array}{*{20}c} {n - 1} \\ {q_{B} } \\ \end{array} } \right)}}\left( {\frac{\alpha }{1 - \alpha }} \right)^{2k - 1} ,$ which in turn holds iff $\left[ {\frac{{\left( {\begin{array}{*{20}c} {n - 1} \\ {q_{A} - k} \\ \end{array} } \right)}}{{\left( {\begin{array}{*{20}c} {n - 1} \\ {q_{B} - k} \\ \end{array} } \right)}}\frac{{\left( {\begin{array}{*{20}c} {n - 1} \\ {q_{B} } \\ \end{array} } \right)}}{{\left( {\begin{array}{*{20}c} {n - 1} \\ {q_{A} } \\ \end{array} } \right)}}} \right]^{{n - q_{A} - q_{B} }} > \left[ {\frac{{\left( {\begin{array}{*{20}c} {n - 1} \\ {q_{A} } \\ \end{array} } \right)}}{{\left( {\begin{array}{*{20}c} {n - 1} \\ {q_{B} } \\ \end{array} } \right)}}} \right]^{2k - 1} .$ Since $\frac{{\left( {\begin{array}{*{20}c} {n - 1} \\ {q_{A} - k} \\ \end{array} } \right)}}{{\left( {\begin{array}{*{20}c} {n - 1} \\ {q_{B} - k} \\ \end{array} } \right)}} \cdot \frac{{\left( {\begin{array}{*{20}c} {n - 1} \\ {q_{B} } \\ \end{array} } \right)}}{{\left( {\begin{array}{*{20}c} {n - 1} \\ {q_{A} } \\ \end{array} } \right)}} = \frac{{\left( {\begin{array}{*{20}c} {q_{A} } \\ k \\ \end{array} } \right)}}{{\left( {\begin{array}{*{20}c} {q_{B} } \\ k \\ \end{array} } \right)}} \cdot \frac{{\left( {\begin{array}{*{20}c} {n - 1 - q_{B} + k} \\ k \\ \end{array} } \right)}}{{\left( {\begin{array}{*{20}c} {n - 1 - q_{A} + k} \\ k \\ \end{array} } \right)}} > 1$ and $\frac{{\left( {\begin{array}{*{20}c} {n - 1} \\ {q_{A} } \\ \end{array} } \right)}}{{\left( {\begin{array}{*{20}c} {n - 1} \\ {q_{B} } \\ \end{array} } \right)}}$ > 1, it suffices to show that $\left[ {\frac{{\left( {\begin{array}{*{20}c} {n - 1} \\ {q_{A} - k} \\ \end{array} } \right)}}{{\left( {\begin{array}{*{20}c} {n - 1} \\ {q_{B} - k} \\ \end{array} } \right)}}\frac{{\left( {\begin{array}{*{20}c} {n - 1} \\ {q_{B} } \\ \end{array} } \right)}}{{\left( {\begin{array}{*{20}c} {n - 1} \\ {q_{A} } \\ \end{array} } \right)}}} \right]^{{n - q_{A} - q_{B} - 1}} > \left[ {\frac{{\left( {\begin{array}{*{20}c} {n - 1} \\ {q_{A} } \\ \end{array} } \right)}}{{\left( {\begin{array}{*{20}c} {n - 1} \\ {q_{B} } \\ \end{array} } \right)}}} \right]^{2k} ,$ i.e., $\left[ {\mathop \prod \limits_{s = 0}^{k - 1} \frac{{(q_{A} - s)(n - q_{B} + s)}}{{(q_{B} - s)(n - q_{A} + s)}}} \right]^{{n - q_{A} - q_{B} - 1}} > \left[ {\mathop \prod \limits_{t = 1}^{{n - q_{A} - q_{B} - 1}} \frac{{(q_{A} + t)(n - q_{B} - t)}}{{(q_{B} + t)(n - q_{A} - t)}}} \right]^{k}$. For that purpose, it suffices to establish that $\forall \varvec{s} \in \left\{ {0,,1, \ldots ,k - 1} \right\}$, $\forall t \in \left\{ {1, \ldots ,n - q_{A} - q_{B} - 1} \right\}, \frac{{(q_{A} - s)(n - q_{B} + s)}}{{(q_{B} - s)(n - q_{A} + s)}} > \frac{{(q_{A} + t)(n - q_{B} - t)}}{{(q_{B} + t)(n - q_{A} - t)}},$ which clearly holds. Claim (6) is thus established.$\hfill \square$

Proof of Lemma 3

We consider separately the two scenarios, $n \le q_{A} + q_{B} + 1$ and $n > q_{A} + q_{B} + 1$.

When $n \le q_{A} + q_{B} + 1,$$f\left( {\alpha ,n} \right) = 0$ implies that $\alpha > \frac{1}{2}$, while $q_{A} Pr\left( {s_{A} = q_{A} ; \alpha , n - 1} \right) > q_{B} Pr\left( {s_{B} = q_{B} ;\beta , n - 1} \right)$ holds if and only if $\frac{{q_{A} \left( {\begin{array}{*{20}c} {n - 1} \\ {q_{A} } \\ \end{array} } \right)}}{{q_{B} \left( {\begin{array}{*{20}c} {n - 1} \\ {q_{B} } \\ \end{array} } \right)}} > \left( {\frac{\alpha }{\beta }} \right)^{{n - 1 - q_{A} - q_{B} }} .$ But $\frac{{q_{A} \left( {\begin{array}{*{20}c} {n - 1} \\ {q_{A} } \\ \end{array} } \right)}}{{q_{B} \left( {\begin{array}{*{20}c} {n - 1} \\ {q_{B} } \\ \end{array} } \right)}} = \frac{{q_{A} }}{{q_{B} }} \times \frac{{q_{A} + 1}}{{q_{B} + 1}} \times \cdots \times \frac{{n - 1 - q_{B} }}{{n - 1 - q_{A} }} > 1$, while $\left( {\frac{\alpha }{\beta }} \right)^{{n - 1 - q_{A} - q_{B} }} \le 1$. We are done.

When $n > q_{A} + q_{B} + 1,$ define ${\text{h}}\left( {\alpha ,n} \right) \equiv \frac{{q_{A} Pr\left( {s_{A} = q_{A} ; \alpha , n - 1} \right)}}{{q_{B} Pr\left( {s_{B} = q_{B} ;\alpha , n - 1} \right)}} - 1 = \frac{{q_{A} \left( {\begin{array}{*{20}c} {n - 1} \\ {q_{A} } \\ \end{array} } \right)}}{{q_{B} \left( {\begin{array}{*{20}c} {n - 1} \\ {q_{B} } \\ \end{array} } \right)}} \cdot \left( {\frac{1 - \alpha }{\alpha }} \right)^{{n - 1 - q_{A} - q_{B} }} - 1$, decreasing in $\alpha$. But $f\left( {\alpha ,n} \right)$ decreases in $\alpha$ too. Thus, the lemma holds if and only if

$$ {\text{denoting by}}\;\alpha \left( n \right)\;{\text{for}}\;\alpha \;{\text{such that}}\;{\text{h}}\left( {\alpha ,n} \right) = 0, f\left( {\alpha \left( n \right),n} \right) < 0 $$

(7)

It is useful to first establish two claims about the behavior of $\alpha \left( n \right)$:

• i. $\frac{\alpha \left( n \right)}{1 - \alpha \left( n \right)} >$$\frac{{n - q_{B} }}{{n - q_{A} }}$

• ii. $\alpha \left( n \right) > \alpha \left( {n + 1} \right)$

To verify claim (i), it suffices to note that as a consequence of $\alpha \left( n \right) > \frac{1}{2}$,

$\left[ {\frac{\alpha \left( n \right)}{1 - \alpha \left( n \right)}} \right]^{{n - q_{A} - q_{B} }} > \left[ {\frac{\alpha \left( n \right)}{1 - \alpha \left( n \right)}} \right]^{{n - 1 - q_{A} - q_{B} }} = \frac{{q_{A} }}{{q_{B} }} \times \frac{{q_{A} + 1}}{{q_{B} + 1}} \times \cdots \times \frac{{n - 1 - q_{B} }}{{n - 1 - q_{A} }} > \left( {\frac{{n - q_{B} }}{{n - q_{A} }}} \right)^{{n - q_{A} - q_{B} }}$ from which immediately follows (i). To verify claim (ii), it is easy to show simply by definition that $\left[ {\frac{{\alpha \left( {n + 1} \right)}}{{1 - \alpha \left( {n + 1} \right)}}} \right]^{{n - q_{A} - q_{B} }} = \left[ {\frac{\alpha \left( n \right)}{1 - \alpha \left( n \right)}} \right]^{{n - 1 - q_{A} - q_{B} }} \times \frac{{n - q_{B} }}{{n - q_{A} }}$, by which, in light of (i), we are led to $\left[ {\frac{{\alpha \left( {n + 1} \right)}}{{1 - \alpha \left( {n + 1} \right)}}} \right]^{{n - q_{A} - q_{B} }} < \left[ {\frac{\alpha \left( n \right)}{1 - \alpha \left( n \right)}} \right]^{{n - q_{A} - q_{B} }} ,$ hence, $\alpha \left( {n + 1} \right) < \alpha \left( n \right)$.

We now establish formula (7) by invoking induction backward over n for fixed $q_{A}$ and $q_{B}$. Note that for any $\alpha ,$

$$ \begin{aligned} f\left( {\alpha ,n} \right) = \,& \alpha^{{q_{A} - 1}} \left( {1 - \alpha } \right)^{{n - q_{A} }} \left( {\begin{array}{*{20}c} {n - 1} \\ {q_{A} - 1} \\ \end{array} } \right)\left\{ {1 + \mathop \sum \limits_{{s \le q_{A} - 2}} \left( {\frac{1 - \alpha }{\alpha }} \right)^{{q_{A} - 1 - s}} \frac{{\left( {\begin{array}{*{20}c} {n - 1} \\ s \\ \end{array} } \right)}}{{\left( {\begin{array}{*{20}c} {n - 1} \\ {q_{A} - 1} \\ \end{array} } \right)}}} \right\} \\ & - \alpha^{{n - q_{B} }} \left( {1 - \alpha } \right)^{{q_{B} - 1}} \left( {\begin{array}{*{20}c} {n - 1} \\ {q_{B} - 1} \\ \end{array} } \right)\left\{ {1 + \mathop \sum \limits_{{s \le q_{B} - 2}} \left( {\frac{\alpha }{1 - \alpha }} \right)^{{q_{B} - 1 - s}} \frac{{\left( {\begin{array}{*{20}c} {n - 1} \\ s \\ \end{array} } \right)}}{{\left( {\begin{array}{*{20}c} {n - 1} \\ {q_{B} - 1} \\ \end{array} } \right)}}} \right\} \\ \end{aligned} $$

The two sigma terms in the above are both insignificant compared to one for sufficiently large n, since $\alpha \left( n \right) \approx \frac{1}{2}$ then. Thus the sign of $f\left( {\alpha \left( n \right),n} \right)$ is the same as that of $\left( {\begin{array}{*{20}c} {n - 1} \\ {q_{A} - 1} \\ \end{array} } \right)/\left( {\begin{array}{*{20}c} {n - 1} \\ {q_{B} - 1} \\ \end{array} } \right) - \left[ {\frac{\alpha \left( n \right)}{1 - \alpha \left( n \right)}} \right]^{{n - q_{A} - q_{B} + 1}} ,$ which in turn, by definition of $\alpha \left( n \right)$, equals $\frac{{n - q_{B} }}{{n - q_{A} }} \times \left[ {\frac{\alpha \left( n \right)}{1 - \alpha \left( n \right)}} \right]^{{n - q_{A} - q_{B} - 1}} - \left[ {\frac{\alpha \left( n \right)}{1 - \alpha \left( n \right)}} \right]^{{n - q_{A} - q_{B} + 1}} = \left[ {\frac{\alpha \left( n \right)}{1 - \alpha \left( n \right)}} \right]^{{n - q_{A} - q_{B} - 1}} \left\{ {\frac{{n - q_{B} }}{{n - q_{A} }} - \left[ {\frac{\alpha \left( n \right)}{1 - \alpha \left( n \right)}} \right]^{2} } \right\}.$ In view of (i), $f\left( {\alpha \left( n \right),n} \right) < 0$. for sufficiently large n.

Now assume that inequality (7) holds for a finite $n + 1( > q_{A} + q_{B} + 2)$, and consider the scenario when the number of bidders is reduced to $n$. For any $\alpha$, $Pr\left( {s_{A} \le q_{A} - 1; \alpha , n} \right) = Pr\left( {s_{A} \le q_{A} - 1;\alpha , n - 1} \right) - \alpha Pr\left( {s_{A} = q_{A} - 1; \alpha , n - 1} \right),$ and $Pr\left( {s_{B} \le q_{B} - 1; \beta , n} \right) = Pr\left( {s_{B} \le q_{B} - 1;\beta , n - 1} \right) - \beta Pr\left( {s_{B} = q_{B} - 1; \beta , n - 1} \right).$ By claim (ii) and the assumption that $Pr\left( {s_{A} \le q_{A} - 1; \alpha \left( {n + 1} \right), n} \right) < Pr\left( {s_{B} \le q_{B} - 1; \alpha \left( {n + 1} \right), n} \right)$, we obtain that, $Pr\left( {s_{A} \le q_{A} - 1; \alpha \left( n \right), n} \right) < Pr\left( {s_{A} \le q_{A} - 1; \alpha \left( {n + 1} \right), n} \right) < Pr\left( {s_{B} \le q_{B} - 1; \alpha \left( {n + 1} \right), n} \right) < Pr\left( {s_{B} \le q_{B} - 1; \alpha \left( n \right), n} \right).$ Thus, to establish $Pr\left( {s_{A} \le q_{A} - 1;\alpha \left( n \right), n - 1} \right)$ < $Pr\left( {s_{B} \le q_{B} - 1;\alpha \left( n \right), n - 1} \right)$, it suffices to show that $\alpha Pr\left( {s_{A} = q_{A} - 1; \alpha \left( n \right), n - 1} \right) < \left( {1 - \alpha } \right)Pr\left( {s_{B} = q_{B} - 1; \alpha \left( n \right), n - 1} \right),$ which amounts to $\frac{{q_{A} \left( {\begin{array}{*{20}c} {n - 1} \\ {q_{A} } \\ \end{array} } \right)}}{{q_{B} \left( {\begin{array}{*{20}c} {n - 1} \\ {q_{B} } \\ \end{array} } \right)}} \times \frac{{n - q_{B} }}{{n - q_{A} }} < \left[ {\frac{\alpha \left( n \right)}{1 - \alpha \left( n \right)}} \right]^{{n - q_{A} - q_{B} }}$, which in turn, by the definition of $\alpha \left( n \right)$, amounts to $\frac{{n - q_{B} }}{{n - q_{A} }} < \frac{\alpha \left( n \right)}{1 - \alpha \left( n \right)}$, i.e., claim (i). Thus, formula (7) holds for any $n > q_{A} + q_{B} + 1.$$\hfill \square$

Proof of Proposition 2

It follows from Eq. (1) that $\overline{{e_{A} }} > \overline{{e_{B} }}$ if and only if for the equilibrium $\alpha$, $\left( {\frac{{q_{A} }}{\alpha }} \right)\left[ {1 - \Pr \left( {s_{A} \le q_{A} ;\alpha ,n} \right)} \right] > \left( {\frac{{q_{B} }}{\beta }} \right)\left[ {1 - \Pr \left( {s_{B} \le q_{B} ;\beta ,n} \right)} \right]$, i.e.,

$$ \begin{aligned} & \left( {\frac{{q_{A} }}{\alpha }} \right)\left[ {1 - Pr\left( {s_{A} \le q_{A} - 1;\alpha , n - 1} \right) - \beta Pr\left( {s_{A} = q_{A} ; \alpha , n - 1} \right) } \right] \\ & \quad > \left( {\frac{{q_{B} }}{\beta }} \right)\left[ {1 - Pr\left( {s_{B} \le q_{B} - 1;\beta , n - 1} \right) - \alpha Pr\left( {s_{B} = q_{B} ; \beta , n - 1} \right)} \right] \\ \end{aligned} $$

(8)

By Lemma 2, $1 - Pr\left( {s_{A} \le q_{A} - 1;\alpha , n - 1} \right) - \beta Pr\left( {s_{A} = q_{A} ; \alpha , n - 1} \right) > 1 - Pr\left( {s_{B} \le q_{B} - 1;\beta , n - 1} \right) - \alpha Pr\left( {s_{B} = q_{B} ; \beta , n - 1} \right).$ It thus suffices to show,

$$ {\text{when}}\;n \ge q_{A} + q_{B} + 1\;{\text{and}}\;q_{A} > q_{B} ,\;\frac{{q_{A} }}{\alpha } > \frac{{q_{B} }}{\beta }\;{\text{at equilibrium}}\;f\left( {\alpha ,n} \right) = 0 $$

(9)

Note that $f\left( {\alpha ,n} \right)$ decreases in $\alpha$. Thus, (9) holds if and only if

$$ f\left( {\frac{{q_{A} }}{{q_{A} + q_{B} }},n} \right) < 0 $$

(10)

We shall establish inequality (10) in a three steps.

First, we show that inequality (10) holds when $n = q_{A} + q_{B} + 1$. Our point of departure in reasoning is the case when the gap between $q_{A}$ and $q_{B}$ assumes the smallest possible number, namely $q_{A} = q_{B} + 1$. The inequality is then equivalent to

$$ \begin{aligned} & \left( {\begin{array}{*{20}c} {2q_{A} - 1} \\ {q_{A} - 1} \\ \end{array} } \right)q_{A}^{{q_{A} - 1}} \left( {q_{A} - 1} \right)^{{q_{A} }} \\ & \quad < \mathop \sum \limits_{{s \le q_{A} - 2}} \left( {\begin{array}{*{20}c} {2q_{A} - 1} \\ s \\ \end{array} } \right)(q_{A} - 1)^{s} q_{A}^{s} [q_{A}^{{2q_{A} - 1 - 2s}} - \left( {q_{A} - 1} \right)^{{2q_{A} - 1 - 2s}} ] \\ \end{aligned} $$

But the RHS of this inequality equals

$$ \begin{aligned} & \mathop \sum \limits_{{s \le q_{A} - 2}} \left( {\begin{array}{*{20}c} {2q_{A} - 1} \\ s \\ \end{array} } \right)(q_{A} - 1)^{s} q_{A}^{s} \mathop \sum \limits_{t = 0}^{{2q_{A} - 2s - 2}} q_{A}^{{2q_{A} - 2s - 2 - t}} (q_{A} - 1)^{t} \\ & \quad > \mathop \sum \limits_{{s \le q_{A} - 2}} \left( {\begin{array}{*{20}c} {2q_{A} - 1} \\ s \\ \end{array} } \right)(q_{A} - 1)^{s} q_{A}^{s} \left( {2q_{A} - 2s - 1} \right)q_{A}^{{q_{A} - s - 1}} (q_{A} - 1)^{{q_{A} - s - 1}} \\ \end{aligned} $$

which can be shown by elementary algebraic manipulation to be equaling LHS. We now induct over $q_{A}$, assuming that $f\left( {\frac{{q_{A} }}{{q_{A} + q_{B} }},n} \right) \le 0$ holds when $q_{A} + q_{B} = n - 1$ and $q_{A} \ge q_{B}$, and that the weak inequality in $f\left( {\frac{{q_{A} }}{{q_{A} + q_{B} }},n} \right) \le 0$ turns out to be equality only if $q_{A} = q_{B}$. Invoking once again the well-known incomplete Beta functional form of the binomial distribution $Pr\left( {s_{A} \le q_{A} - 1; \alpha , n - 1} \right) = \left( {n - 1} \right)\left( {\begin{array}{*{20}c} {n - 2} \\ {q_{A} - 1} \\ \end{array} } \right)\mathop \int \limits_{0}^{1 - \alpha } t^{{n - 1 - q_{A} }} \left( {1 - t} \right)^{{q_{A} - 1}} dt,$ we obtain, with some abuse of notation,¹³

$$ \begin{aligned} \Delta f \equiv & f\left( {\frac{{q_{A} + 1}}{n - 1},n} \right) - f\left( {\frac{{q_{A} }}{n - 1},n} \right) \\ = & \left( {n - 1} \right)\left( {\begin{array}{*{20}c} {n - 2} \\ {q_{A} } \\ \end{array} } \right)\mathop \int \limits_{0}^{{1 - \frac{{q_{A} + 1}}{n - 1}}} t^{{n - q_{A} - 2}} \left( {1 - t} \right)^{{q_{A} }} dt - \left( {n - 1} \right)\\ & \left( {\begin{array}{*{20}c} {n - 2} \\ {q_{A} + 1} \\ \end{array} } \right)\mathop \int \limits_{0}^{{\frac{{q_{A} + 1}}{n - 1}}} t^{{q_{A} + 1}} \left( {1 - t} \right)^{{n - q_{A} - 3}} dt \\ & - \left( {n - 1} \right)\left( {\begin{array}{*{20}c} {n - 2} \\ {q_{A} - 1} \\ \end{array} } \right)\mathop \int \limits_{0}^{{1 - \frac{{q_{A} }}{n - 1}}} t^{{n - q_{A} - 1}} \left( {1 - t} \right)^{{q_{A} - 1}} dt + \left( {n - 1} \right)\\ &\quad \left( {\begin{array}{*{20}c} {n - 2} \\ {q_{A} } \\ \end{array} } \right)\mathop \int \limits_{0}^{{\frac{{q_{A} }}{n - 1}}} t^{{q_{A} }} \left( {1 - t} \right)^{{n - q_{A} - 2}} dt. \\ \end{aligned} $$

Noting that $\mathop \int \limits_{0}^{{\frac{{q_{A} + 1}}{n - 1}}} t^{{q_{A} + 1}} \left( {1 - t} \right)^{{n - q_{A} - 3}} dt = - \frac{{t^{{q_{A} + 1}} \left( {1 - t} \right)^{{n - q_{A} - 2}} }}{{n - q_{A} - 2}}\left| {\begin{array}{*{20}c} {\frac{{q_{A} + 1}}{n - 1}} \\ {0 } \\ \end{array} } \right. + \frac{{q_{A} + 1}}{{n - q_{A} - 2}}\mathop \int \limits_{0}^{{\frac{{q_{A} + 1}}{n - 1}}} t^{{q_{A} }} \left( {1 - t} \right)^{{n - q_{A} - 2}} dt,$ and that $\mathop \int \limits_{0}^{{1 - \frac{{q_{A} }}{n - 1}}} t^{{n - q_{A} - 1}} \left( {1 - t} \right)^{{q_{A} - 1}} dt = - \frac{{t^{{n - q_{A} - 1}} \left( {1 - t} \right)^{{q_{A} }} }}{{q_{A} }}\left| {\begin{array}{*{20}c} {1 - \frac{{q_{A} }}{n - 1}} \\ {0 } \\ \end{array} } \right. + \frac{{n - q_{A} - 1}}{{q_{A} }}\mathop \int \limits_{0}^{{1 - \frac{{q_{A} }}{n - 1}}} t^{{n - q_{A} - 2}} \left( {1 - t} \right)^{{q_{A} }} dt,$ we obtain $\Delta f = - 2\left( {n - 1} \right)\left( {\begin{array}{*{20}c} {n - 2} \\ {q_{A} } \\ \end{array} } \right)\left\{ {\mathop \int \limits_{{\frac{{q_{A} }}{n - 1}}}^{{\frac{{q_{A} + 1}}{n - 1}}} t^{{q_{A} }} \left( {1 - t} \right)^{{n - 2 - q_{A} }} dt - \frac{1}{{2\left( {n - 1} \right)}}\left[ {\left( {\frac{{q_{A} + 1}}{n - 1}} \right)^{{q_{A} }} \left( {1 - \frac{{q_{A} + 1}}{n - 1}} \right)^{{n - 2 - q_{A} }} + \left( {\frac{{q_{A} }}{n - 1}} \right)^{{q_{A} }} \left( {1 {-} \frac{{q_{A} }}{n - 1}} \right)^{{n - 2 - q_{A} }} } \right]} \right\} .$ But it can be shown that $h\left( t \right) \equiv t^{{q_{A} }} \left( {1 - t} \right)^{{n - 2 - q_{A} }}$ as a function of $t$ is concave over the interval $\left[ {\frac{{q_{A} }}{n - 1},\frac{{q_{A} + 1}}{n - 1}} \right]$, as a consequence of which, by the long known Hermite–Hadamard inequality (refer to, e.g., Hardy et al. 1999, p. 98, Theorem 125), we have $\mathop \int \limits_{{\frac{{q_{A} }}{n - 1}}}^{{\frac{{q_{A} + 1}}{n - 1}}} h\left( t \right)dt > \left( {\frac{{q_{A} + 1}}{n - 1} - \frac{{q_{A} }}{n - 1}} \right) \times \frac{1}{2}\left[ {h\left( {\frac{{q_{A} + 1}}{n - 1}} \right) + h\left( {\frac{{q_{A} }}{n - 1}} \right)} \right] = \frac{1}{{2\left( {n - 1} \right)}}\left[ {h\left( {\frac{{q_{A} + 1}}{n - 1}} \right) + h\left( {\frac{{q_{A} }}{n - 1}} \right)} \right]$. That is, $f\left( {\frac{{q_{A} + 1}}{n - 1},n} \right) - f\left( {\frac{{q_{A} }}{n - 1},n} \right) < 0.$ It thus follows that $f\left( {\frac{{q_{A} + 1}}{n - 1},n} \right) < f\left( {\frac{{q_{A} }}{n - 1},n} \right) \le 0$.

Second, (10) holds when $n$ is sufficiently large for fixed $q_{A}$ and $q_{B}$. It is already shown in the proof of Lemma 3 that for such $n$ the sign of $f\left( {\frac{{q_{A} }}{{q_{A} + q_{B} }},n} \right)$ is the same as that of $\frac{{\left( {\begin{array}{*{20}c} {n - 1} \\ {q_{A} - 1} \\ \end{array} } \right)}}{{\left( {\begin{array}{*{20}c} {n - 1} \\ {q_{B} - 1} \\ \end{array} } \right)}} - \left( {\frac{{q_{A} }}{{q_{B} }}} \right)^{{n - q_{A} - q_{B} + 1}} = \frac{{q_{A} }}{{q_{B} }} \times \frac{{q_{A} + 1}}{{q_{B} + 1}} \times \cdots \times \frac{{n - q_{B} }}{{n - q_{A} }} - \left( {\frac{{q_{A} }}{{q_{B} }}} \right)^{{n - q_{A} - q_{B} + 1}} < 0$.

Third, for fixed $q_{A}$ and $q_{B}$ and the particular value of $\alpha = \frac{{q_{A} }}{{q_{A} + q_{B} }}$, we show that there exists certain integer $n^{*} > q_{A} + q_{B} + 1$ such that $s\left( {\alpha ,n} \right) \equiv f\left( {\alpha ,n + 1} \right) - f\left( {\alpha ,n} \right) > 0$ for any $n > n^{*}$ and that if there is any $n < n^{*}$ such that $s\left( {\alpha ,n} \right) < 0$ then $s\left( {\alpha ,n - 1} \right) < 0.$ Note that for any $\alpha$, $Pr\left( {s_{A} \le q_{A} - 1; \alpha , n} \right) = \, Pr\left( {s_{A} \le q_{A} - 1; \alpha , n - 1} \right) - \left( {\begin{array}{*{20}c} {n - 1} \\ {q_{A} - 1} \\ \end{array} } \right)\alpha^{{q_{A} }} (1 - \alpha )^{{n - q_{A} }} ,$ and $Pr\left( {s_{B} \le q_{B} - 1; \alpha , n} \right) = Pr\left( {s_{B} \le q_{B} - 1; \alpha , n - 1} \right) - \left( {\begin{array}{*{20}c} {n - 1} \\ {q_{B} - 1} \\ \end{array} } \right)\left( {1 - \alpha } \right)^{{q_{B} }} \alpha^{{n - q_{B} }} .$ As a consequence, $s\left( {\alpha ,n} \right) = \left( {\begin{array}{*{20}c} {n - 1} \\ {q_{B} - 1} \\ \end{array} } \right)\left( {1 - \alpha } \right)^{{q_{B} }} \alpha^{{n - q_{B} }} - \left( {\begin{array}{*{20}c} {n - 1} \\ {q_{A} - 1} \\ \end{array} } \right)\alpha^{{q_{A} }} (1 - \alpha )^{{n - q_{A} }} = \left( {\begin{array}{*{20}c} {n - 1} \\ {q_{B} - 1} \\ \end{array} } \right)\alpha^{{q_{A} }} \left( {1 - \alpha } \right)^{{n - q_{A} }} \left[ {\left( {\frac{\alpha }{1 - \alpha }} \right)^{{n - q_{A} - q_{B} }} - \frac{{\left( {\begin{array}{*{20}c} {n - 1} \\ {q_{A} - 1} \\ \end{array} } \right)}}{{\left( {\begin{array}{*{20}c} {n - 1} \\ {q_{B} - 1} \\ \end{array} } \right)}}} \right]$. Thus, the sign of $s\left( {\frac{{q_{A} }}{{q_{A} + q_{B} }},n} \right)$ is the same as that of $\left( {\frac{{q_{A} }}{{q_{B} }}} \right)^{{n - q_{A} - q_{B} }} - \frac{{\left( {\begin{array}{*{20}c} {n - 1} \\ {q_{A} - 1} \\ \end{array} } \right)}}{{\left( {\begin{array}{*{20}c} {n - 1} \\ {q_{B} - 1} \\ \end{array} } \right)}} = \left( {\frac{{q_{A} }}{{q_{B} }}} \right)^{{n - q_{A} - q_{B} }} - \underbrace {{\frac{{q_{A} }}{{q_{B} }} \times \frac{{q_{A} + 1}}{{q_{B} + 1}} \times \cdots \times \frac{{n - q_{B} }}{{n - q_{A} }}}}_{{\left( {n - q_{A} - q_{B} + 1} \right) terms}}$, which is clearly positive for sufficiently large $n$, say for any $n$ such that $\frac{{n - q_{B} - 1}}{{n - q_{A} - 1}} \times \frac{{n - q_{B} }}{{n - q_{A} }} \le \frac{{q_{A} }}{{q_{B} }}$, i.e., any $n \ge q_{A} + q_{B} + \frac{1}{2} + \sqrt {q_{A} q_{B} + \frac{1}{4}}$. We now show that for any possible $n$ such that $s\left( {\frac{{q_{A} }}{{q_{A} + q_{B} }},n} \right) < 0$, i.e., $\left( {\frac{{q_{A} }}{{q_{B} }}} \right)^{{n - q_{A} - q_{B} }} < \frac{{\left( {\begin{array}{*{20}c} {n - 1} \\ {q_{A} - 1} \\ \end{array} } \right)}}{{\left( {\begin{array}{*{20}c} {n - 1} \\ {q_{\varvec{B}} - 1} \\ \end{array} } \right)}}$, we must have $s\left( {\frac{{q_{A} }}{{q_{A} + q_{B} }},n - 1} \right) < 0,$ i.e., $\left( {\frac{{q_{A} }}{{q_{B} }}} \right)^{{n - 1 - q_{A} - q_{B} }} < \frac{{\left( {\begin{array}{*{20}c} {n - 2} \\ {q_{A} - 1} \\ \end{array} } \right)}}{{\left( {\begin{array}{*{20}c} {n - 2} \\ {q_{B} - 1} \\ \end{array} } \right)}}$. Note that $\frac{{\left( {\begin{array}{*{20}c} {n - 1} \\ {q_{A} - 1} \\ \end{array} } \right)}}{{\left( {\begin{array}{*{20}c} {n - 1} \\ {q_{B} - 1} \\ \end{array} } \right)}} = \frac{{\left( {\begin{array}{*{20}c} {n - 2} \\ {q_{A} - 1} \\ \end{array} } \right)}}{{\left( {\begin{array}{*{20}c} {n - 2} \\ {q_{B} - 1} \\ \end{array} } \right)}} \times \frac{{n - q_{B} }}{{n - q_{A} }}$, it thus follows from $s\left( {\frac{{q_{A} }}{{q_{A} + q_{B} }},n} \right) < 0$ that $\left( {\frac{{q_{A} }}{{q_{B} }}} \right)^{{n - 1 - q_{A} - q_{B} }} < \frac{{\left( {\begin{array}{*{20}c} {n - 2} \\ {q_{A} - 1} \\ \end{array} } \right)}}{{\left( {\begin{array}{*{20}c} {n - 2} \\ {q_{B} - 1} \\ \end{array} } \right)}} \times \frac{{n - q_{B} }}{{n - q_{A} }} \times \frac{{q_{B} }}{{q_{A} }} < \frac{{\left( {\begin{array}{*{20}c} {n - 2} \\ {q_{A} - 1} \\ \end{array} } \right)}}{{\left( {\begin{array}{*{20}c} {n - 2} \\ {q_{B} - 1} \\ \end{array} } \right)}}$ since $\frac{{n - q_{B} }}{{n - q_{A} }} \times \frac{{q_{B} }}{{q_{A} }} < 1$. for any $n > q_{A} + q_{B}$.

We conclude that $f\left( {\frac{{q_{A} }}{{q_{A} + q_{B} }},n} \right)$ is either U-shaped or monotonically increasing as $n \ge q_{A} + q_{B} + 1$ grows, but always negative. Proposition 2 is established.$\hfill \square$

Proof of Proposition 3

We start with the fact that $\frac{{d\Pr \left( {s_{A} \le q_{A} ;\alpha ,n} \right)}}{d\alpha } = - n\Pr \left( {s_{A} = q_{A} ;\alpha ,n - 1} \right)$ and that $\frac{{d\Pr \left( {s_{B} \le q_{B} ;\alpha ,n} \right)}}{d\alpha } = n\Pr \left( {s_{B} = q_{B} ;\alpha ,n - 1} \right)$, of which the algebraic verification is easy and omitted here to save space. By Eq. (4), we obtain,

$$ \frac{{d\bar{e}\left( \alpha \right)}}{d\alpha } = \frac{v}{c} \cdot \left[ {q_{A} \Pr \left( {s_{A} = q_{A} ;\alpha ,n - 1} \right) - q_{B} \Pr \left( {s_{B} = q_{B} ;\alpha ,n - 1} \right)} \right] $$

As a consequence of Lemma 3, $\frac{{d\bar{e}\left( \alpha \right)}}{d\alpha } > 0$ at $\alpha = \alpha^{*}$. Hence $\alpha^{*} < \tilde{\alpha }$.$\hfill \square$