Skip to main content
Springer Nature Link
Log in

Residual-based a posteriori error estimate for a mixed Reißner-Mindlin plate finite element method

  • Published:
Numerische Mathematik Aims and scope Submit manuscript

Abstract

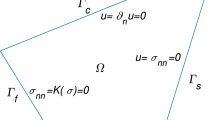
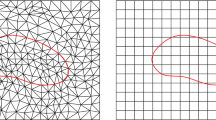
Reliable and efficient residual-based a posteriori error estimates are established for the stabilised locking-free finite element methods for the Reissner-Mindlin plate model. The error is estimated by a computable error estimator from above and below up to multiplicative constants that do neither depend on the mesh-size nor on the plate's thickness and are uniform for a wide range of stabilisation parameter. The error is controlled in norms that are known to converge to zero in a quasi-optimal way. An adaptive algorithm is suggested and run for improving the convergence rates in three numerical examples for thicknesses 0.1, .001 and .001.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Arnold, D.N., Brezzi, F.: Some new elements for the Reissner-Mindlin plate model. In: J.L. Lions, C. Baiocchi (eds.) Boundary value problems for partial differential equations and applications. Masson, 1993, pp. 287–292.

  2. Arnold, D.N., Falk, R.S.: A uniformly accurate finite element method for the Reissner-Mindlin plate model. SIAM J. Numer. Anal. 26, 1276–1290 (1989)

    Article  MATH  MathSciNet  Google Scholar 

  3. Babuška, I., Rheinboldt, W.C.: Error estimates for adaptive finite element computations. SIAM J. Numer. Anal. 15, 736–754 (1978)

    Article  MathSciNet  Google Scholar 

  4. Bathe, K.J., Brezzi, F., Fortin, M.: Mixed-interpolated elements for Reissner-Mindlin plates. Internat. J. Numer. Methods. Engrg. 28, 1787–1801 (1989)

    Article  MATH  MathSciNet  Google Scholar 

  5. Boffi, D., Lovadina, C.: Analysis of new augmented Lagrangian formulations for mixed finite element schemes. Numer. Math. 75, 405–419 (1997)

    Article  MATH  MathSciNet  Google Scholar 

  6. Braess, D.: Stability of saddle point problems with penalty. M2AN 30 No. 6 731–742 (1996)

    Google Scholar 

  7. Braess, D.: Finite Elements. Cambridge University Press, 1997

  8. Brezzi, F., Fortin, M.: Mixed and hybrid finite element methods. Springer-Verlag, 1991

  9. Brezzi, F., Fortin, M., Stenberg, R.: Error analysis of mixed-interpolated elements for Reissner-Mindlin plates. Math. Models and Methods in Appl. Sci. 1, 125–151 (1991)

    Article  MATH  MathSciNet  Google Scholar 

  10. Brenner, S.C., Scott, L.R.: The mathematical theory of finite element methods. Texts in Applied Mathematics 15 Springer New York, 1994

  11. Chapelle, D., Stenberg, R.: An optimal low-order locking-free finite element method for Reissner-Mindlin plates. Math. Mod. Meth. Appl. Sc. 8(3), 407–430 (1998)

    Article  MathSciNet  Google Scholar 

  12. Carstensen, C.: Quasi-interpolation and a posteriori error analysis in finite element method. M2AN 33, 1187–1202 (1999)

    Google Scholar 

  13. Carstensen, C.: Residual-Based A Posteriori Error Estimate for a Nonconforming Reissner-Mindlin Plate Finite Element. SIAM J. Numer. Anal. 39 2034–2044 (2002)

    Google Scholar 

  14. Ciarlet, P.G.: The finite element method for elliptic problems. North-Holland, Amsterdam, 1978

  15. Clément, P.: Approximation by finite element functions using local regularization. RAIRO Sér. Rouge Anal. Numér. R-2, 77–84 (1975)

  16. Eriksson, K., Estep, D., Hansbo, P., Johnson, C.: Introduction to adaptive methods for differential equations. Acta Numerica, 105–158 (1995)

  17. Girault, V., Raviart, P.-A.: Finite Element Methods for Navier-Stokes Equations. Berlin–Heidelberg–New York–Tokyo: Springer, 1986

  18. Liebermann, E.: A posteriori error estimator for a mixed finite element method for the Reissner-Mindlin plate. Math. Comp. (2000)

  19. Lovadina, C.: A new class of finite elements for Reissner-Mindlin plates. SIAM J. Numer. Anal. 33, 2457–2467 (1996)

    Article  MATH  MathSciNet  Google Scholar 

  20. Lions, J.L., Magenes, E.: Non-homogeneous boundary value problems and applications, Vol. I. Berlin-Heidelberg-New York: Springer, 1972

  21. Schöberl, J.: Multigrid Methods for a class of parameter dependent problems in primal variables. Technical Report 99–03 Spezialforschungsbereich F013, 1999, Johannes Kepler University, 4040-Linz, Austria

  22. Verfürth, R.: A review of a posteriori error estimation and adaptive mesh-refinement techniques. Wiley-Teubner, 1996

  23. Verfürth, R.: Robust a posteriori error estimators for singularly perturbed reaction-diffusion equations. Numer. Math. 78 479–493 (1998)

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Humboldt-Universität zu Berlin, Unter den Linden 6, 10099, Berlin, Germany

    Carsten Carstensen

  2. SFB F013 Numerical and Symbolic Scientific Computing, Johannes Kepler University Linz, Freistädterstrasse 313, 4020, Linz, Austria

    Joachim Schöberl

Authors
  1. Carsten Carstensen
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Joachim Schöberl
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Carsten Carstensen.

Additional information

Supported by the DFG Research Center MATHEON ``Mathematics for key technologies'' in Berlin and by the Austrian Science Fund Fonds zur Förderung der wissenschaftlichen Forschung, Spezialforschungsbereich F013.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Carstensen, C., Schöberl, J. Residual-based a posteriori error estimate for a mixed Reißner-Mindlin plate finite element method. Numer. Math. 103, 225–250 (2006). https://doi.org/10.1007/s00211-005-0669-3

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00211-005-0669-3

Mathematics Subject Classification (1991)