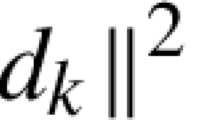Skip to main content
SpringerLink
Log in

A posteriori error estimators, gradient recovery by averaging, and superconvergence

  • Published:
Numerische Mathematik Aims and scope Submit manuscript

Abstract

For the linear finite element solution to a linear elliptic model problem, we derive an error estimator based upon appropriate gradient recovery by local averaging. In contrast to popular variants like the ZZ estimator, our estimator contains some additional terms that ensure reliability also on coarse meshes. Moreover, the enhanced estimator is proved to be (locally) efficient and asymptotically exact whenever the recovered gradient is superconvergent. We formulate an adaptive algorithm that is directed by this estimator and illustrate its aforementioned properties, as well as their importance, in numerical tests.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Ainsworth, M., Craig, A.W.: A posteriori error estimators in the finite element method. Numer. Math. 60, 429–463 (1992)

    Article  MATH  MathSciNet  Google Scholar 

  2. Ainsworth, M., Oden, J.T.: A posteriori error estimation in finite element analysis. Pure and Applied Mathematics (New York), Wiley-Interscience [John Wiley & Sons], New York, 2000

  3. Babuška, I., Strouboulis, T.: The finite element method and its reliability. Numerical Mathematics and Scientific Computation, The Clarendon Press Oxford University Press, New York, 2001.

  4. Bank, R.E., Xu, J.: Asymptotically exact a posteriori error estimators. II. General unstructured grids. SIAM J. Numer. Anal. 41, 2313–2332 (2003) (electronic)

    Article  MATH  MathSciNet  Google Scholar 

  5. Bänsch, E., Morin, P., Nochetto, R.H.: An adaptive Uzawa FEM for the Stokes problem: convergence without the inf-sup condition. SIAM J. Numer. Anal. 40, 1207–1229 (2002) (electronic)

    Article  MATH  MathSciNet  Google Scholar 

  6. Bernardi, C., Verfürth, R.: Adaptive finite element methods for elliptic equations with non-smooth coefficients. Numer. Math. 85, 579–608 (2000)

    Article  MATH  MathSciNet  Google Scholar 

  7. Brenner, S.C., Scott, L.R.: The mathematical theory of finite element methods. vol. 15 of Texts in Applied Mathematics, Springer, New York, 1994

  8. Carstensen, C.: All first-order averaging techniques for a posteriori finite element error control on unstructured grids are efficient and reliable. Math. Comp. 73, 1153–1165 (2004) (electronic)

    Article  MATH  MathSciNet  Google Scholar 

  9. Carstensen, C., Bartels, S.: Each averaging technique yields reliable a posteriori error control in FEM on unstructured grids. I. Low order conforming, nonconforming, and mixed FEM. Math. Comp. 71, 945–969 (2002) (electronic)

    MATH  MathSciNet  Google Scholar 

  10. Carstensen, C., Funken, S.A.: Fully reliable localized error control in the FEM. SIAM J. Sci. Comput., 21 1465–1484, (1999/00) (electronic)

    Google Scholar 

  11. Chen, Z., Wu, H.: Uniform convergence of multigrid V-cycle on adaptively refined finite element meshes for second order elliptic problems. Preprint No. 2003–07, Institute of Computational Mathematics, Chinese Academy of Sciences, Beijing, China, http://icmsec.cc.ac.cn/2003research_report.html

  12. Deuflhard, P., Weiser, M.: Global inexact Newton multilevel FEM for nonlinear elliptic problems. In: Multigrid methods V (Stuttgart, 1996), vol. 3 of Lect. Notes Comput. Sci. Eng., Springer, Berlin, 1998, pp. 71–89

  13. Dörfler, W.: A convergent adaptive algorithm for Poisson's equation. SIAM J. Numer. Anal. 33, 1106–1124 (1996)

    Article  MATH  MathSciNet  Google Scholar 

  14. Faermann, B.: Localization of the Aronszajn-Slobodeckij norm and application to adaptive boundary element methods. II. The three-dimensional case. Numer. Math. 92, 467–499 (2002)

    MATH  MathSciNet  Google Scholar 

  15. Fierro, F., Veeser, A.: A posteriori error estimators for regularized total variation of characteristic functions. SIAM J. Numer. Anal. 41, 2032–2055 (2003) (electronic)

    Article  MATH  MathSciNet  Google Scholar 

  16. Hackbusch, W.: Elliptic differential equations. Theory and numerical treatment. vol. 18 of Springer Series in Computational Mathematics, Springer-Verlag, Berlin, 1992

  17. Luce, R., Wohlmuth, B.I.: A local a posteriori error estimator based on equlibrated fluxes. SIAM J. Numer. Anal. 42, 1394–1414 (2004)

    Article  MATH  MathSciNet  Google Scholar 

  18. Morin, P., Nochetto, R.H., Siebert, K.G.: Data oscillation and convergence of adaptive FEM. SIAM J. Numer. Anal. 38, 466–488 (2000) (electronic)

    Article  MATH  MathSciNet  Google Scholar 

  19. Morin, P., Nochetto, R.H., Siebert, K.G.: Local problems on stars: a posteriori error estimators, convergence, and performance. Math. Comp. 72, 1067–1097 (2003) (electronic)

    Article  MATH  MathSciNet  Google Scholar 

  20. Rodríguez, R.: A posteriori error analysis in the finite element method. In: Finite element methods (Jyväskylä, 1993), vol. 164 of Lecture Notes in Pure and Appl. Math., Dekker, New York, 1994, pp. 389–397

  21. Sacchi, R., Veeser, A.: Locally efficient and reliable a posteriori error estimators for Dirichlet problems. Math. Mod. Meth. Appl. Sci. 16, 319–346 (2006)

    Article  Google Scholar 

  22. Schmidt, A., Siebert, K.G.: Design of adaptive finite element software: the finite element toolbox ALBERTA. no. 42 in Lecture Notes in Computational Sciences and Engineering, Springer, 2004

  23. Scott, L.R., Zhang, S.: Finite element interpolation of nonsmooth functions satisfying boundary conditions. Math. Comp. 54, 483–493 (1990)

    Article  MATH  MathSciNet  Google Scholar 

  24. Strouboulis, T., Babuška, I., Gangaraj, S.K.: Guaranteed computable bounds for the exact error in the finite element solution. II. Bounds for the energy norm of the error in two dimensions. Internat. J. Numer. Methods Engrg. 47, 427–475 (2000) Richard H. Gallagher Memorial Issue

    MATH  MathSciNet  Google Scholar 

  25. Veeser, A.: Convergent adaptive finite elements for the nonlinear Laplacian. Numer. Math. 92, 743–770 (2002)

    Article  MATH  MathSciNet  Google Scholar 

  26. Verfürth, R.: A review of a posteriori error estimation and adaptive mesh-refinement techniques. Adv. Numer. Math., John Wiley, Chichester, UK, 1996

  27. Xu, J.: An introduction to multilevel methods. In: Wavelets, multilevel methods and elliptic PDEs (Leicester, 1996), Numer. Math. Sci. Comput., Oxford Univ. Press, New York, 1997, pp. 213–302

  28. Xu, J., Zhang, Z.: Analysis of recovery type a posteriori error estimators for mildly structured grids. Math. Comp. 73, 1139–1152 (2004) (electronic)

    Article  MATH  MathSciNet  Google Scholar 

  29. Zienkiewicz, O.C., Zhu, J.Z.: A simple error estimator and adaptive procedure for practical engineering analysis. Internat. J. Numer. Methods Eng. 24, 337–357 (1987)

    Article  MATH  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Dipartimento di Matematica, Università degli Studi di Milano, Via C. Saldini 50, 20133, Milano, Italy

    Francesca Fierro & Andreas Veeser

Authors
  1. Francesca Fierro
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Andreas Veeser
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Francesca Fierro.

Additional information

Research partially supported by Italian MIUR Cofin 2003 ``Modellistica numerica per il calcolo scientifico e applicazioni avanzate'' and Cofin 2004 ``Metodi numerici avanzati per equazioni alle derivate parziali di interesse applicativo''.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Fierro, F., Veeser, A. A posteriori error estimators, gradient recovery by averaging, and superconvergence. Numer. Math. 103, 267–298 (2006). https://doi.org/10.1007/s00211-005-0671-9

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00211-005-0671-9

Mathematics Subject Classification (2000)

Search

Navigation