Skip to main content
Springer Nature Link
Log in

Superinfection Behaviors on Scale-Free Networks with Competing Strains

  • Published:
Journal of Nonlinear Science Aims and scope Submit manuscript

Abstract

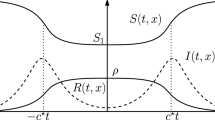
This paper considers the epidemiology of two strains (I,J) of a disease spreading through a population represented by a scale-free network. The epidemiological model is SIS and the two strains have different reproductive numbers. Superinfection means that strain I can infect individuals already infected with strain J, replacing the strain J infection. Individuals infected with strain I cannot be infected with strain J. The model is set up as a system of ordering differential equations and stability of the disease free, marginal strain I and strain J, and coexistence equilibria are assessed using linear stability analysis, supported by simulations. The main conclusion is that superinfection, as modeled in this paper, can allow strain I to coexist with strain J even when it has a lower basic reproductive number. Most strikingly, it can allow strain I to persist even when its reproductive number is less than 1.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5

Similar content being viewed by others

References

  • Ahn, Y.Y., Masuda, N., Jeong, H., Noh, J.D.: Epidemic dynamics of two species of interacting aparticles on scale-free networks. Phys. Rev. E 74, 066113 (2006)

    Article  Google Scholar 

  • Allen Linda, J.S., van den Driessche, P.: The basic reproduction number in some discrete-time epidemic models. J. Differ. Equ. Appl. 14, 1127–1147 (2008)

    Article  MATH  Google Scholar 

  • Barabási, A.L., Albert, R.: Emergence of scaling in random networks. Science 286, 509–512 (1999)

    Article  MathSciNet  Google Scholar 

  • Boldin, B., Diekmann, O.: Superinfections can induce evolutionarily stable coexistence of pathogens. J. Math. Biol. 56, 635–672 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  • Brauer, F.: The Kermack-McKendrick epidemic model revisited. Math. Biosci. 198, 119–131 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  • Cai, L.M., Li, X.Z., Yu, J.Y.: A two-strain epidemic model with super infection and vaccination. Math. Appl. 20, 328–335 (2007)

    MathSciNet  MATH  Google Scholar 

  • Castillo-Chavez, C., Huang, W., Li, J.: Competivtive exclusion and coexistence of multiple strains in an SIS STD model. SIAM J. Appl. Math. 59, 1790–1811 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  • d’Onofrio, A.: A note on the global behaviour of the network-based SIS epidemic model. Nonlinear Anal.: Real World Appl. 9, 1567–1572 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  • Fu, X.C., Small, M., Walker, D.M., Zhang, H.F.: Epidemic dynamics on scale-free networks with piecewise linear infectivity and immunization. Phys. Rev. E 77, 036113 (2008)

    Article  MathSciNet  Google Scholar 

  • Funk, S., Gilad, E., Watkins, C., Jansen, V.A.A.: The spread of awareness and its impact on epidemic outbreaks. Proc. Natl. Acad. Sci. USA 106, 6872 (2009)

    Article  MATH  Google Scholar 

  • Funk, S., Gilad, E., Jansen, V.A.A.: Endemic disease, awareness, and local behavioural response. J. Theor. Biol. 264, 501 (2010)

    Article  Google Scholar 

  • Gross, T., Dlima, C., Dommar, J., Blasius, B.: Epidemic dynamics on an adaptive network. Phys. Rev. Lett. 96, 208701 (2006)

    Article  Google Scholar 

  • Jin, Z., Zhang, J.P., Song, L.P., Sun, G.Q., Kang, J.L., Zhu, H.P.: Modelling and analysis of influenza A (H1N1) on networks. BMC Public Health 11, S9 (2011)

    Google Scholar 

  • Kamo, M., Sasaki, A.: The effect of cross-immunity and seasonal forcing in a multi-strain epidemic model. Physica D 165, 228–241 (2002)

    Article  MATH  Google Scholar 

  • Li, K., Small, M., Zhang, H.F., Fu, X.C.: Epidemic outbreaks on networks with effective contacts. Nonlinear Anal.: Real World Appl. 11, 1017–1025 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  • Lou, J., Ruggeri, T.: The dynamics of spreading and immune strategies of sexually transmitted diseases on scale-free network. J. Math. Anal. Appl. 365, 210–219 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  • Madar, M., Kalisky, T., Cohen, R., ben-Avraham, D., Havlin, S.: Immunization and epidemic dynamics in complex networks. Eur. Phys. J. B 38, 269 (2004)

    Article  Google Scholar 

  • Marceau, V., Noël, P.-A., Hébert-Dufresne, L., Allard, A., Dubé, L.J.: Modeling the dynamical interaction between epidemics on overlay networks. Phys. Rev. E 84, 026105 (2011)

    Article  Google Scholar 

  • Masuda, N., Konno, N.: Multi-sate epidemic processes on complex networks. J. Theor. Biol. 243, 64–75 (2006)

    Article  MathSciNet  Google Scholar 

  • Miller, J.C.: Epidemic size and probability in populations with heterogeneous infectivity and susceptibility. Phys. Rev. E 76, 010101 (2007)

    Article  Google Scholar 

  • Newman, M.E.J.: Threshold effects for two pathogens spreading on a network. Phys. Rev. Lett. 95, 108701 (2005)

    Article  Google Scholar 

  • Nowak, M.A., May, R.M.: Superinfection and the evolution of parasite virulence. Proc. R. Soc. Lond. B, Biol. Sci. 255, 81–89 (1994)

    Article  Google Scholar 

  • NuNo, M., Feng, Z., Martcheva, M., Castillo-Chavez, C.: Dynamics of two-strain influenza with isolation and partial cross-immunity. SIAM J. Appl. Math. 65(3), 964–982 (2005)

    Article  MathSciNet  MATH  Google Scholar 

  • Olinky, R., Stone, L.: Unexpected epidemic thresholds in heterogeneous networks: The role of disease transmission. Phys. Rev. E 70, 030902(R) (2004)

    Article  Google Scholar 

  • Pastor-Satorras, R., Vespignani, A.: Epidemic spreading in scale-free networks. Phys. Rev. Lett. 86, 3200–3203 (2001)

    Article  Google Scholar 

  • Pastor-Satorras, R., Vespignani, A.: Epidemic dynamics in finite size scale-free networks. Phys. Rev. E 65, 035108(R) (2002)

    Google Scholar 

  • Pastor-Satorras, R., Vespignani, A.: Immunization of complex networks. Phys. Rev. E 65, 036104 (2002)

    Article  Google Scholar 

  • Shaw, L.B., Schwartz, I.B.: Fluctuating epidemics on adaptive networks. Phys. Rev. E 77, 066101 (2008)

    Article  MathSciNet  Google Scholar 

  • Shrestha, S., King, A.A., Rohani, P.: Statistical inference for multi-pathogen systems. PLoS Comput. Biol. 7(8), e1002135 (2011)

    Article  MathSciNet  Google Scholar 

  • Small, M., Tse, C.K.: Small world and scale free model of transmission of SARS. Int. J. Bifurc. Chaos 15, 1745–1755 (2005)

    Article  Google Scholar 

  • Small, M., Walker, D.M., Tse, C.K.,: Scale-free distribution of avian influenza outbreaks. Phys. Rev. Lett. 99, 188702 (2007)

    Article  Google Scholar 

  • Smith, D.M., Richman, D.D., Little, S.J.: HIV superinfection. J. Infect. Dis. 192, 438–444 (2005)

    Article  Google Scholar 

  • Thomasey, D.H., Martcheva, M.: Serotype replacement of vertically transmitted disease through perfect vaccination. J. Biol. Syst. 16, 255–277 (2008)

    Article  MATH  Google Scholar 

  • Vasco, D.A., Wearing, H.J., Rohani, P.: Tracking the dynamics of pathogen interactions: Modeling ecological and immune-mediated processes in a two-pathogen single-host system. J. Theor. Biol. 245, 9 (2007)

    Article  MathSciNet  Google Scholar 

  • Wang, L., Dai, G.Z.: Global stability of virus spreading in complex heterogeneous networks. SIAM J. Appl. Math. 68, 1495–1502 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  • Wang, Y., Chakrabarti, D., Wangand, C., Faloutsos, C.: Epidemic spreading in real networks: an eigenvalue viewpoint. In: Proc. IEEE SRDS (2003)

    Google Scholar 

  • Wang, Y.B., Xiao, G.X., Liu, J.: Dynamics of competing ideas in complex social systems. New J. Phys. 14, 013015 (2012)

    Article  Google Scholar 

  • Wu, Q.C., Fu, X.C., Small, M., Zhang, H.F.: Oscillations and phase transition in the mean infection rate of a finite population. Int. J. Mod. Phys. C 21, 1207–1215 (2010)

    Article  MATH  Google Scholar 

  • Wu, Q.C., Fu, X.C., Yang, M.: Epidemic thresholds in a heterogenous population with competing strains. Chin. Phys. B 20, 046401 (2011)

    Article  Google Scholar 

  • Zou, S.F., Wu, J.H., Chen, Y.M.: Multiple epidemic waves in delayed susceptible-infected-recovered models on complex networks. Phys. Rev. E 83, 056121 (2011)

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. College of Mathematics and Information Science, Jiangxi Normal University, Nanchang, 330022, China

    Qingchu Wu

  2. School of Mathematics and Statistics, The University of Western Australia, Crawley, WA, 6009, Australia

    Michael Small

  3. Faculty of Science, Guangdong Ocean University, Zhanjiang, 524088, China

    Huaxiang Liu

Authors
  1. Qingchu Wu
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Michael Small
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. Huaxiang Liu
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Qingchu Wu.

Additional information

Communicated by P. Newton.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Wu, Q., Small, M. & Liu, H. Superinfection Behaviors on Scale-Free Networks with Competing Strains. J Nonlinear Sci 23, 113–127 (2013). https://doi.org/10.1007/s00332-012-9146-1

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00332-012-9146-1

Keywords

Mathematics Subject Classification