Skip to main content
Springer Nature Link
Log in

A Nonlinear Diffusion Equation-Based Model for Ultrasound Speckle Noise Removal

  • Published:
Journal of Nonlinear Science Aims and scope Submit manuscript

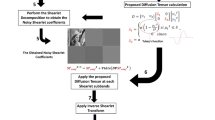
Abstract

Ultrasound images are contaminated by speckle noise, which brings difficulties in further image analysis and clinical diagnosis. In this paper, we address this problem in the view of nonlinear diffusion equation theories. We develop a nonlinear diffusion equation-based model by taking into account not only the gradient information of the image, but also the information of the gray levels of the image. By utilizing the region indicator as the variable exponent, we can adaptively control the diffusion type which alternates between the Perona–Malik diffusion and the Charbonnier diffusion according to the image gray levels. Furthermore, we analyze the proposed model with respect to the theoretical and numerical properties. Experiments show that the proposed method achieves much better speckle suppression and edge preservation when compared with the traditional despeckling methods, especially in the low gray level and low-contrast regions.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13

Similar content being viewed by others

References

  • Abd-Elmoniem, K.Z., Youssef, A.B., Kadah, Y.M.: Real-time speckle reduction and coherence enhancement in ultrasound imaging via nonlinear anisotropic diffusion. IEEE Trans. Biomed. Eng. 49(9), 997–1014 (2002)

    Article  Google Scholar 

  • Abrahim, B. A., Kadah, Y.: Speckle noise reduction method combining total variation and wavelet shrinkage for clinical ultrasound imaging. In: 2011 1st IEEE Middle East Conference on Biomedical Engineering (2011)

  • Achim, A., Bezerianos, A., Tsakalides, P.: Novel Bayesian multiscale method for speckle removal in medical ultrasound images. IEEE Trans. Med. Imaging 20(8), 772–783 (2001)

    Article  Google Scholar 

  • Aubert, G., Aujol, J.F.: A variational approach to remove multiplicative noise. SIAM J. Appl. Math. 4(68), 925–946 (2008)

    Article  MATH  Google Scholar 

  • Aubert, G., Kornprobst, P.: Mathematical Problems in Image Processing: Partial Differential Equations and the Calculus of Variations, vol. 147. Springer, Berlin (2006)

    MATH  Google Scholar 

  • Barcelos, C. A., Vieira, L. E.: Ultrasound speckle noise reduction via an adaptive edge-controlled variational method. In: 2014 IEEE International Conference on Systems, Man and Cybernetics (2014)

  • Brezis, H.: Analyse Fonctionelle. Masson, Paris (1992)

    Google Scholar 

  • Calvetti, D., Reichel, L.: Adaptive Richardson iteration based on Leja points. J. Comput. Appl. Mathe. 71, 267–286 (1996)

    Article  MathSciNet  MATH  Google Scholar 

  • Catté, F., Lions, P.L., Morel, J.M., Coll, T.: Image selective smoothing and edge detection by nonlinear diffusion. SIAM J. Numer. Anal. 29, 182–193 (1992)

    Article  MathSciNet  MATH  Google Scholar 

  • Chen, Y., Levine, S., Rao, M.: Variable exponent linear growth functionals in image restoration. SIAM J. Appl. Math. 66(4), 1383–1406 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  • Cottet, G.H., Germain, L.: Image processing through reaction combined with nonlinear diffusion. Math. Comput. 61, 659–667 (1993)

    Article  MathSciNet  MATH  Google Scholar 

  • Coupé, P., Hellier, P., Kervrann, C., Barillot, C.: Bayesian nonlocal means–based speckle filtering. In: 2008 5th IEEE International Symposium on Biomedical Imaging: From Nano to Macro (2008)

  • Coupé, P., Hellier, P., Kervrann, C., Barillot, C.: Nonlocal means-based speckle filtering for ultrasound images. IEEE Trans. Image Process. 18(10), 2221–2229 (2009)

    Article  MathSciNet  MATH  Google Scholar 

  • Durand, S., Fadili, J., Nikolova, M.: Multiplicative noise removal using L1 fidelity on frame coefficients. J. Math. Imaging Vis. 36(3), 201–226 (2010)

    Article  Google Scholar 

  • Dutt, V., Greenleaf, J.F.: Adaptive speckle reduction filter for log-compressed B-scan images. IEEE Trans. Med. Imaging 15(6), 802–813 (1996)

    Article  Google Scholar 

  • Foucher, S., Bénié, G.B., Boucher, J.M.: Multiscale MAP filtering of SAR images. IEEE Trans. Image Process. 10(1), 49–60 (2001)

    Article  MATH  Google Scholar 

  • Frost, V.S., Stiles, J.A., Shanmugan, K.S., Holtzman, J.C.: A model for radar images and its application to adaptive digital filtering of multiplicative noise. IEEE Trans. Pattern Anal. Mach. Intell. 4(2), 157–166 (1982)

    Article  Google Scholar 

  • Goodman, J.W.: Some fundamental properties of speckle. J. Opt. Soc. Am. 66(11), 1145–1150 (1976)

    Article  Google Scholar 

  • Guo, Z., Sun, J., Zhang, D., Wu, B.: Adaptive Perona–Malik model based on the variable exponent for image denoising. IEEE Trans. Image Process. 21(3), 958–967 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  • Gupta, S., Chauhan, R.C., Sexana, S.C.: Wavelet-based statistical approach for speckle reduction in medical ultrasound images. Med. Biol. Eng. Comput. 42(2), 189–192 (2004)

    Article  Google Scholar 

  • Hacini, M., Hachouf, F., Djemal, K.: A new speckle filtering method for ultrasound images based on a weighted multiplicative total variation. Signal Process. 103, 214–229 (2014)

    Article  Google Scholar 

  • Jin, Z.M., Yang, X.P.: A variational model to remove the multiplicative noise in ultrasound images. J. Math. Imaging Vis. 39(1), 62–74 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  • Karaman, M., Kutay, M.A., Bozdagi, G.: An adaptive speckle suppression filter for medical ultrasonic imaging. IEEE Trans. Med. Imaging 14(2), 283–292 (1995)

    Article  Google Scholar 

  • Khare, A., Khare, M., Jeong, Y., Kim, H., Jeon, M.: Despeckling of medical ultrasound images using Daubechies complex wavelet transform. Signal Process. 90(2), 428–439 (2010)

    Article  MATH  Google Scholar 

  • Krissian, K., KiKinis, R., Westin, C. F., Vosburgh, K.: Speckle-constrained filtering of ultrasound images. In: 2005 IEEE Computer Society Conference on Computer Vision and Pattern Recognition (2005)

  • Krissian, K., Westin, C.F., Kikinis, R., Vosburgh, K.G.: Oriented speckle reducing anisotropic diffusion. IEEE Trans. Image Process. 16(5), 1412–1424 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  • Kuan, D.T., Sawchuk, A.A., Strand, T.C., Chavel, P.: Adaptive noise smoothing filter for images with signal-dependent noise. IEEE Trans. Pattern Anal. Mach. Intell. 7(2), 165–177 (1985)

    Article  Google Scholar 

  • Lee, J.S.: Digital image enhancement and noise filtering by use of local statistics. IEEE Trans. Pattern Anal. Mach. Intell. 2(2), 165–168 (1980)

    Article  MathSciNet  Google Scholar 

  • Loupas, T., McDicken, W.N., Allan, P.L.: An adaptive weighted median filter for speckle suppression in medical ultrasonic images. IEEE Trans. Circuits Syst. 36(1), 129–135 (1989)

    Article  Google Scholar 

  • Perona, P., Malik, J.: Scale-space and edge detection using anisotropic diffusion. IEEE Trans. Pattern Anal. Mach. Intell. 12(7), 629–639 (1990)

    Article  Google Scholar 

  • Poynton, C.: Digital Video and HD: Algorithms and Interfaces, vol. 260. Elsevier, Amsterdam (2012)

    Google Scholar 

  • Ramos-Llordé, G., Vegas-Sánchez-Ferrero, G., Martin-Fernandez, M., Alberola-López, C., Aja-Fernández, S.: Anisotropic diffusion filter with memory based on speckle statistics for ultrasound Images. IEEE Trans. Image Process. 24(1), 345–358 (2015)

    Article  MathSciNet  Google Scholar 

  • Rudin, L., Osher, S., Fatemi, E.: Nonlinear total variation based noise removal algorithms. Phys. D. 60, 259–268 (1992)

    Article  MathSciNet  MATH  Google Scholar 

  • Sudeep, P.V., Palanisamy, P., Rajan, J., Baradaran, H., Saba, L., Gupta, A., Suri, J.S.: Speckle reduction in medical ultrasound images using an unbiased non-local means method. Biomed. Signal Process. Control 28, 1–8 (2016)

    Article  Google Scholar 

  • Sudha, S., Suresh, G.R., Sukanesh, R.: Speckle noise reduction in ultrasound images by wavelet thresholding based on weighted variance. Intl. J. Comput. Theory Eng. 1(1), 7 (2009)

    Article  Google Scholar 

  • Teuber, T., Lang, A.: A new similarity measure for nonlocal filtering in the presence of multiplicative noise. Comput. Stat. Data Anal. 56(12), 3821–3842 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  • Tur, M., Chin, K.C., Goodman, J.W.: When is speckle noise multiplicative. Appl. Opt. 21(7), 1157–1159 (1982)

    Article  Google Scholar 

  • Wagner, R.F., Smith, S.W., Sandrik, J.M., Lopez, H.: Statistics of speckle in ultrasound B-scans. IEEE Trans. Sonics Ultrason. 30(3), 156–163 (1983)

    Article  Google Scholar 

  • Wang, L., Xiao, L., Huang, L., Wei, Z.: Nonlocal total variation based speckle noise removal method for ultrasound image. In: 2011 4th IEEE International Congress on Image and Signal Processing (2011)

  • Wang, Z., Bovik, A.C., Sheikh, H.R., Simoncelli, E.P.: Image quality assessment: from error visibility to structural similarity. IEEE Trans. Image Process. 13(4), 600–612 (2004)

    Article  Google Scholar 

  • Weickert, J.: A Review of Nonlinear Diffusion Filtering. Scale-space theory in computer vision, pp. 1–28. Springer, Berlin (1997)

    Google Scholar 

  • Weickert, J.: Anisotropic Diffusion in Image Processing, pp. 59–60. B. G. Teubner, Stuttgart (1998)

  • Yang, J., Fan, J., Ai, D., Wang, X., Zheng, Y., Tang, S., Wang, Y.: Local statistics and non-local mean filter for speckle noise reduction in medical ultrasound image. Neurocomputing 195, 88–95 (2016)

    Article  Google Scholar 

  • Yu, Y.J., Acton, S.T.: Speckle reducing anisotropic diffusion. IEEE Trans. Image Process. 11(11), 1260–1270 (2002)

    Article  MathSciNet  Google Scholar 

  • Zhan, Y., Ding, M., Wu, L., Zhang, X.: Nonlocal means method using weight refining for despeckling of ultrasound images. Signal Process. 103, 201–213 (2014)

    Article  Google Scholar 

  • Zhang, F., Yoo, Y.M., Koh, L.M., Kim, Y.: Nonlinear diffusion in Laplacian pyramid domain for ultrasonic speckle reduction. IEEE Trans. Med. Imaging 26(2), 200–211 (2007)

    Article  Google Scholar 

  • Zhou, Z., Guo, Z., Dong, G., Sun, J., Zhang, D., Wu, B.: A doubly degenerate diffusion model based on the gray level indicator for multiplicative noise removal. IEEE Trans. Image Process. 24(1), 249–260 (2015)

    Article  MathSciNet  Google Scholar 

  • Zong, X.L., Laine, A.F., Geiser, E.A.: Speckle reduction and contrast enhancement of echocardiograms via multiscale nonlinear processing. IEEE Trans. Med. Imaging 17(4), 532–540 (1998)

    Article  Google Scholar 

Download references

Acknowledgements

This work is partially supported by the National Science Foundation of China (U1637208 and 11501149), the National Science Foundation of China (11271100 and 11301113), the Fundamental Research Funds for the Central Universities and Program for Innovation Research of Science in Harbin Institute of Technology (PIRS OF HIT 201609) and the Fundamental Research Funds for the Central Universities and Program for Innovation Research of Science in Harbin Institute of Technology (PIRS OF HIT 201601).

Author information

Authors and Affiliations

  1. Department of Mathematics, Harbin Institute of Technology, 92 West Dazhi Street, Nan Gang District, Harbin, Heilongjiang, China

    Zhenyu Zhou, Zhichang Guo, Dazhi Zhang & Boying Wu

Authors
  1. Zhenyu Zhou
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Zhichang Guo
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. Dazhi Zhang
    View author publications

    You can also search for this author inPubMed Google Scholar

  4. Boying Wu
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Zhichang Guo.

Additional information

Communicated by Andrea Bertozzi.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Zhou, Z., Guo, Z., Zhang, D. et al. A Nonlinear Diffusion Equation-Based Model for Ultrasound Speckle Noise Removal. J Nonlinear Sci 28, 443–470 (2018). https://doi.org/10.1007/s00332-017-9414-1

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00332-017-9414-1

Keywords

Mathematics Subject Classification