Modeling and Analysis of a Nonlinear Age-Structured Model for Tumor Cell Populations with Quiescence

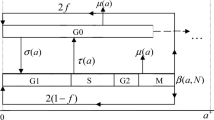
Abstract

We present a nonlinear first-order hyperbolic partial differential equation model to describe age-structured tumor cell populations with proliferating and quiescent phases at the avascular stage in vitro. The division rate of the proliferating cells is assumed to be nonlinear due to the limitation of the nutrient and space. The model includes a proportion of newborn cells that enter directly the quiescent phase with age zero. This proportion can reflect the effect of treatment by drugs such as erlotinib. The existence and uniqueness of solutions are established. The local and global stabilities of the trivial steady state are investigated. The existence and local stability of the positive steady state are also analyzed. Numerical simulations are performed to verify the results and to examine the impacts of parameters on the nonlinear dynamics of the model.

Existence and Uniqueness of Solutions

Consider a Banach space $\mathbf {X}=L^1(0,a^+)\times L^1(0,a^+)$ endowed with the norm $||\phi ||=||\phi _1||+||\phi _2||$ for $\phi (a)=(\phi _1(a),\phi _2(a))^T\in \mathbf {X}$, where $||\cdot ||$ is the norm in $L^1$ and $v^T$ is the transpose of the vector v.

Now we define a linear operator $A: D(A)\subset \mathbf {X}\rightarrow \mathbf {X}$ by

$$\begin{aligned} (A\phi )(a):=\left( -k\frac{d}{da}\phi _1(a), -k\frac{d}{da}\phi _2(a)\right) ^\mathrm{T}. \end{aligned}$$

(A.1)

The domain D(A) is given as

$$\begin{aligned} D(A)= & {} \left\{ \phi \in \mathbf {X}_+:=L_+^1(0,a^+)\times L_+^1(0,a^+):\ \phi _1,\phi _2\in AC[0,a^+], \right. \\&\left. \phi (0)=(\phi _1(0),\phi _2(0))^T\right\} , \end{aligned}$$

where $L_+^1(0,a^+)$ denotes the positive cone of $L^1(0,a^+)$ and $AC[0,a^+]$ is the set of absolutely continuous functions on $[0,a^+)$, $\phi _1(0)=2(1-f)\int _0^{a^+} \beta (a,N(t))\phi _1(a)\hbox {d}a$ and $\phi _2(0)=2f\int _0^{a^+} \beta (a,N(t))\phi _1(a)\hbox {d}a$. We also define a nonlinear operator $F: \mathbf {X}_+\rightarrow \mathbf {X}$ by

$$\begin{aligned} (F\phi )(a):= \bigg (\begin{array}{cc} -\mu (a)\phi _1(a)-\beta (a,N)\phi _1(a)+\sigma (a)\phi _2(a) \\ -\mu (a)\phi _2(a)-\sigma (a)\phi _2(a)\end{array} \bigg ). \end{aligned}$$

(A.2)

Based on Assumption ($\text{ H }_1$), it is not difficult to prove that the operator F is Lipschitz continuous and there exists a positive constant $r>0$ such that

$$\begin{aligned} (I+rF)(\mathbf {X}_+)\subset \mathbf {X}_+, \end{aligned}$$

(A.3)

where I denotes the identity operator. The proof of this result can be referred to Inaba (2006).

Set $u(t)=(P(t,\cdot ),Q(t,\cdot ))^T$. Then system (2.1)–(2.3) can be formulated as a nonlinear Cauchy problem on the Banach space $\mathbf {X}$:

$$\begin{aligned} \frac{\hbox {d}u(t)}{\hbox {d}t}=Au(t)+F(u(t)),\quad u(0)=u_0\in \mathbf {X}, \end{aligned}$$

(A.4)

where $u_0(a)=(P_0(a),Q_0(a))^\mathrm{T}$. We can see that operator A generates a $C_0$-semigroup $\{\hbox {e}^{tA}\}_{t\geqslant 0}$ and there exist numbers $M\geqslant 1$ and $\alpha >0$ such that

$$\begin{aligned} ||\hbox {e}^{tA}||\leqslant M\hbox {e}^{\alpha t}. \end{aligned}$$

(A.5)

Let $r>0$ be a constant such that (A.3) holds. Using this r and according to Busenberg et al. (1991), abstract Cauchy problem (A.4) can be rewritten as

$$\begin{aligned} \frac{\hbox {d}u(t)}{\hbox {d}t}=\left( A-\frac{1}{r}\right) u(t)+\frac{1}{r}(I+rF)u(t),\quad u(0)=u_0\in \mathbf {X}. \end{aligned}$$

(A.6)

Investigating problem (A.6), we obtain the mild solution by the solution of the integral equation

$$\begin{aligned} u(t)=\hbox {e}^{-\frac{1}{r}t}\hbox {e}^{tA}u_0+\frac{1}{r}\int _0^t\hbox {e}^{-\frac{1}{r}(t-s)}\hbox {e}^{(t-s)A}(I+rF)u(s)\hbox {d}s. \end{aligned}$$

Let $\{S(t)\}_{t\geqslant 0}$ be the semiflow defined by the solutions of the above variation of constants formula. Then, $S(t)u_0$ can be given as the limit of the iterative sequence $\{u^n\}_{n\geqslant 0}$ such that

$$\begin{aligned} \left\{ \begin{array}{ll} &{}\displaystyle u^0(t)=u_0,\\ &{}\displaystyle u^{n+1}(t)=\hbox {e}^{-\frac{1}{r}t}\hbox {e}^{tA}u_0 +\frac{1}{r}\int _0^t\hbox {e}^{-\frac{1}{r}(t-s)}\hbox {e}^{(t-s)A}(I+rF)u^n(s)\hbox {d}s. \\ \end{array}\right. \end{aligned}$$

Notice that $u^{n+1}$ is a linear convex combination of $\hbox {e}^{tA}u_0\in \mathbf {X}_+$ and $\hbox {e}^{(t-s)A}(I+rF)u^n\in \mathbf {X}_+$. Then, based on the positivity of $\hbox {e}^{tA}$ and $I+rF$, we conclude that $u^{n+1}\in \mathbf {X}_+$ if $u^n\in \mathbf {X}_+$ by applying (A.3). It follows from the Lipschitz continuity of F that $\{u^n\}$ converges to the mild solution $S(t)u_0\in \mathbf {X}_+$ uniformly. Applying (A.5), we have the estimate

$$\begin{aligned} ||u(t)||\leqslant M\hbox {e}^{(\alpha -\frac{1}{r})t}||u_0||+\frac{MK}{r}\int _0^t\hbox {e}^{(\alpha -\frac{1}{r})(t-s)}||u(s)||\hbox {d}s, \end{aligned}$$

where $K:=||I+rF||$. From the Gronwall inequality, we can estimate that:

$$\begin{aligned} ||u(t)||\leqslant ||u_0||M\hbox {e}^{(\alpha -\frac{1-MK}{r})t}. \end{aligned}$$

Because the norm of the local solution grows at most exponentially as time evolves, it can be extended to a global one. Hence, the solution $S(t)u_0, t > 0,$ is global.

Finally, we say that Cauchy problem (A.4) has a unique mild solution $S(t)u_0\in \mathbf {X}_+$ for each $u_0\in \mathbf {X}_+$, and $\mathbf {X}_+$ is positively invariant with respect to the semiflow $\{S(t)\}_{t\geqslant 0}$.