Abstract
The main purpose of this paper is to analyze the Hopf-like bifurcations in 3D piecewise linear systems. Such bifurcations are characterized by the birth of a piecewise smooth limit cycle that bifurcates from a singular point located at the discontinuity manifold. In particular, this paper concerns systems of the form \({\dot{x}}=Ax+b^{\pm }\) which are ubiquitous in control theory. For this class of systems, we show the occurrence of two distinct types of Hopf-like bifurcations, each of which gives rise to a crossing limit cycle (CLC). Conditions on the system parameters for the coexistence of two CLCs and the occurrence of a saddle-node bifurcation of these CLCs are provided. Furthermore, the local asymptotic stability of the pseudo-equilibrium point is analyzed and applications in discontinuous control systems are presented.













Similar content being viewed by others
Notes
In power electronics, this continuous qualifier makes reference to the fact that the current is not allowed to be zero, nothing to do with the continuity of the vector field. In this case, the converter operates with a non-null inductance current at any time, i.e., \(i_L>0\).
References
Cardoso, J.L., Llibre, J., Novaes, D.D., Tonon, D.J.: Simultaneous occurrence of sliding and crossing limit cycles in piecewise linear planar vector fields. Dyn. Syst. 35(3), 124818 (2020)
Castillo, J., Llibre, J., Verduzco, F.: The pseudo-Hopf bifurcation for planar discontinuous piecewise linear differential systems. Nonlinear Dyn. 90, 1829–1840 (2017)
Cristiano, R., Pagano, D.J.: Two-parameter boundary equilibrium bifurcations in 3D-Filippov systems. J. Nonlinear Sci. 29(6), 2845–2875 (2019)
Cristiano, R., Pagano, D.J., Freire, E., Ponce, E.: Revisiting the Teixeira singularity bifurcation analysis. Application to the control of power converters. Int. J. Bifurc. Chaos 28(9), 1850106 (2018)
Cristiano, R., Pagano, D.J., Carvalho, T., Tonon, D.J.: Bifurcations at a degenerate two-fold singularity and crossing limit cycles. J. Differ. Equ. 268(1), 115–140 (2019a)
Cristiano, R., Ponce, E., Pagano, D.J., Granzotto, M.: On the Teixeira singularity bifurcation in a dc-dc power electronic converter. Nonlinear Dyn. 96(2), 1243–1266 (2019b)
di Bernardo, M., Johansson, K.H., Vasca, F.: Self-oscillations and sliding in relay feedback systems: symmetry and bifurcations. Int. J. Bifurc. Chaos 11(04), 1121–1140 (2001)
de Carvalho, T., Cristiano, R., Gonçalves, L.F., Tonon, D.J.: Global analysis of the dynamics of a mathematical model to intermittent HIV treatment. Nonlinear Dyn. 101, 719–739 (2020)
de Freitas, B.R., Llibre, J., Medrado, J.C.: Limit cycles of continuous and discontinuous piecewise-linear differential systems in R3. J. Comput. Appl. Math. 338, 311–323 (2018)
Dumortier, F., Llibre, J., Artés, J.: Qualitative Theory Of Planar Differential Systems. Universitext. Springer, Berlin (2006)
Euzébio, R.D., Llibre, J.: On the number of limit cycles in discontinuous piecewise linear differential systems with two pieces separated by a straight line. J. Math. Anal. Appl. 424(1), 475–486 (2015)
Filippov, A.F.: Differential equations with discontinuous righthand sides, volume 18 of Mathematics and its Applications (Soviet Series). Kluwer Academic Publishers Group, Dordrecht, 1988. Translated from the Russian
Freire, E., Ponce, E., Torres, F.: Hopf-like bifurcations in planar piecewise linear systems. Publicacions Matemátiques 41, 135–148 (1997)
Freire, E., Ponce, E., Torres, F.: Canonical discontinuous planar piecewise linear systems. SIAM J. Appl. Dyn. Syst. 11(1), 181–211 (2012)
Freire, E., Ponce, E., Torres, F.: A general mechanism to generate three limit cycles in planar Filippov systems with two zones. Nonlinear Dyn. 78(1), 251–263 (2014)
Harris, J., Ermentrout, B.: Bifurcations in the Wilson–Cowan equations with nonsmooth firing rate. SIAM J. Appl. Dyn. Syst. 14(1), 43–72 (2015)
Jacquemard, A., Tonon, D.J.: Coupled systems of non-smooth differential equations. Bull. Sci. Math. 136(3), 239–255 (2012)
Jacquemard, A., Teixeira, M.A., Tonon, D.J.: Piecewise smooth reversible dynamical systems at a two-fold singularity. Int. J. Bifurc. Chaos Appl. Sci. Eng. 22(8), 1250192 (2012)
Jacquemard, A., Teixeira, M.A., Tonon, D.J.: Stability conditions in piecewise smooth dynamical systems at a two-fold singularity. J. Dyn. Control Syst. 19(1), 47–67 (2013)
Kuznetsov, Y.A., Rinaldi, S., Gragnani, A.: One-parameter bifurcations in planar Filippov systems. Int. J. Bifurc. Chaos 13(8), 2157–2188 (2003)
Llibre, J., Novaes, D.D., Teixeira, M.A.: Maximum number of limit cycles for certain piecewise linear dynamical systems. Nonlinear Dyn. 82, 1159–1175 (2015)
Olivar, G., Angulo, F., di Bernardo, M.: Hopf-like transitions in nonsmooth dynamical systems. In: 2004 IEEE International Symposium on Circuits and Systems (IEEE Cat. No.04CH37512), vol. 4, pp. IV–693 (2004)
Rodrigues, D.S., Mancera, P.F.A., Carvalho, T., Gonçalves, L.F.: Sliding mode control in a mathematical model to chemoimmunotherapy: The occurrence of typical singularities. Applied Mathematics and Computation, Elsevier, vol. 387, p. 124782 (2020)
Simpson, D.J.W.: A compendium of Hopf-like bifurcations in piecewise-smooth dynamical systems. Phys. Lett. A 382(35), 2439–2444 (2018)
Simpson, D.: Hopf-like boundary equilibrium bifurcations involving two foci in Filippov systems. J. Differ. Equ. 267(11), 6133–6151 (2019)
Utkin, V.: Discussion aspects of high-order sliding mode control. IEEE Trans. Autom. Control 61(3), 829–833 (2016)
Zou, F., Nossek, J.A.: Hopf-like bifurcation in cellular neural networks. In: 1993 IEEE International Symposium on Circuits and Systems, vol. 4, pp. 2391–2394 (1993)
Author information
Authors and Affiliations
Corresponding author
Additional information
Communicated by George Haller.
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Appendices
Appendix A. Proof of Proposition 1
We are assume that in hypothesis (H1) the condition \(p_{32}\ne 0\) hold, while \(p_{31}\) can be null or not. Affine transformation of the state space of system (2) on the state space of system (4), provided by Proposition 1, is obtained by applying the change of coordinates
where \({\mathbf {x}}\) denotes the state vector of (2) and \({\mathbf {y}}\) denotes the state vector of (4). In addition, \(T=T_2T_1\) is an invertible matrix (assuming \(p_{32}\ne 0\) and \(\det \left( M\right) \ne 0\), hypotheses (H1) and (H2) respectively), where
with
\(r_{12}=p_{22}+p_{33}+\frac{p_{12}p_{31}}{p_{32}},\) \(r_{13}=p_{13}p_{31}+p_{32}p_{23}-\left( p_{12}p_{31}+p_{22}p_{32}\right) \frac{p_{33}}{p_{32}}\); and \({\mathbf {x}}_p\) is the pseudo-equilibrium of (2) with coordinates
such that \(\det \left( R\right) \ne 0\) (hypothesis (H3)) and
The canonical form (4) has the vector fields
where \({\mathbf {b}}=\begin{bmatrix} \nu&\beta&\mu \end{bmatrix}^T\). Note that the switching boundary \(\Sigma \) remains the same as the original system. From (22) and (23), we can write the parameters \(a_{11}\), \(a_{12}\), \(a_{13}\), \(\mu \), \(\alpha \), \(\beta \) and \(\nu \), as functions of the parameters of the original system (2), just as stated in Proposition 1.
The proposed transformation moves the pseudo-equilibrium \({\mathbf {x}}_p\) of (2) to the origin of the new state space of (4). Therefore, it is natural that the vectors \({\mathbf {F}}^{+}({\mathbf {0}})\) and \({\mathbf {F}}^{-}({\mathbf {0}})\) are parallel and written as well as presented in canonical form, since the equation \(\alpha {\mathbf {F}}^{+}({\mathbf {0}})+(1-\alpha ){\mathbf {F}}^{-}({\mathbf {0}})={\mathbf {0}}\) must be satisfied for some value of \(\alpha \). The transformation matrices \(T_1\) and \(T_2\) are defined to obtain the tangency lines parallel to the x-axis and to decrease the number of parameters in canonical form, respectively.
Appendix B. Construction of the First Return Map
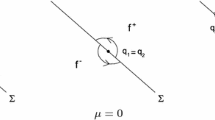
We consider a trajectory of system (4) initiated at the point \({\mathbf {x}}_0=(x_0,y_0,0)\in D_0^+\subset \Sigma _c^{+}\) such that for \(t=t_+>0\) this trajectory transversally returns to \(\Sigma \) at the point \({\mathbf {x}}_1=(x_1,y_1,0)\in D_1^+\subset \Sigma _c^{-}\). In addition, we consider a trajectory of system (4) initiated at \({\mathbf {x}}_1\) such that for \(t=t_->0\) this trajectory transversally returns to \(\Sigma \) at the point \({\mathbf {x}}_2=(x_2,y_2,0)\in D_0^-\subset \Sigma _c^{+}\). See illustration in Fig. 14. Then, we define the half-return maps \((x_1,y_1)=\Phi ^+(x_0,y_0)\) and \((x_2,y_2)=\Phi ^-(x_1,y_1)\).
Based on the conditions stated above and assuming that \(D_0^-\subset D_0^+\), the first return map is given by \(\Phi (x_0,y_0)=\Phi ^-(\Phi ^+(x_0,y_0))\) and defined for all \((x_0,y_0,0)\in D_0^+\subset \Sigma _c^{+}\). The fixed points of \(\Phi \) that are located in \(\Sigma _c^{+}\) represent CLCs, so that the stability analysis of the fixed points can be naturally extended to the CLCs. Our goal is to study the existence and stability of CLCs in the neighborhood of the origin of system (4) for \(|\mu |\) small. For that, it is necessary that, for \(\mu =0\), the orbits have invisible quadratic contact with \(\Sigma \) at (0,0,0), that is, at the origin it must have an invisible two-fold tangency, as illustrated in Fig. 14. As analyzed in Sect. 3, this scenario is obtained whenever
We then assume the above constraints on the parameters \(\beta \) and \(\alpha \).
In order to find the explicit expressions for the coefficients of each half-return map, we will determine below the solution of system (4) for \(z\ge 0\) and for \(z\le 0\). We start with the solution for \(z\ge 0\) with initial condition at \({\mathbf {x}}_0\), which can be obtained from the variation of constants formula
It will suffice to have the Taylor’s series approximation until the sixth-order terms for (24), namely
where \(M=A{\mathbf {x}}_0+{\mathbf {b}}^+\) and I is the identity matrix of order 3.
From the third component of (25), we can determine an expression for the half-return time \(t_+=t_+(x_0,y_0)\), which depends on \((x_0,y_0)\) and such that \(z(t_+)=0\). The polynomial approximation for the time \(t_+\) is given by
for \(|(x_0,y_0-(1-\alpha )\mu )|\) small, where \(y_0>(1-\alpha )\mu \) and \(\Psi _{\mu }(0,(1-\alpha )\mu )=\frac{2}{(1-\alpha )\beta }>0\). For our purpose, we must compute all the coefficients of the polynomial \(\Psi _{\mu }(x_0,y_0)\) up to the fourth-order terms. Some of these coefficients are very large expressions and therefore we do not make them explicit.
Now, substituting (26) in the first and the second component of (25), the half-return map \((x_1,y_1)=\Phi ^+(x_0,y_0)\) is expressed by
For our purpose it is sufficient to consider the expansion of \(\Phi ^+\) in \((x_0,y_0-(1-\alpha )\mu )\) up to the fifth-degree terms. The computations for the map \(\Phi ^-\) are totally similar. We compute the approximation up to fifth order of the half-return time \(t_-=t_-(x_1,y_1)\) for \(|(x_1,y_1+\alpha \mu )|\) small and \(y_1<-\alpha \mu \). This is done by solving the equation \(z(t_-)=0\) so that, after the evaluation at such a time for the other two coordinates of the solution, we determine the image of the half-return map \(\Phi ^-(x_1,y_1)\), namely
We also consider the expansion above in \((x_1,y_1+\alpha \mu )\) up to the fifth-degree terms.
Remark 3
For simplicity, we do not make explicit here all the coefficients of half-return maps \(\Phi ^-\) and \(\Phi ^+\), up to the fifth-degree terms. We make explicit only the coefficients of the first return map \(\Phi =\Phi ^-\circ \Phi ^+\).
Now, we assume |(x, y)| and \(|\mu |\) small enough, satisfying \(y>\text {Max}\left[ (1-\alpha )\mu ,-\alpha \mu \right] \). The first return map is obtained by \(\Phi =\Phi ^-\circ \Phi ^+\) and, thus, we get \(\Phi (x,y)=(f(x,y),g(x,y))\) with
In the expansion in (x, y) above, it is sufficient to consider up to the fifth-degree terms. The coefficients of these terms are:
and
Rights and permissions
About this article
Cite this article
Cristiano, R., Tonon, D.J. & Velter, M.Q. Hopf-Like Bifurcations and Asymptotic Stability in a Class of 3D Piecewise Linear Systems with Applications. J Nonlinear Sci 31, 65 (2021). https://doi.org/10.1007/s00332-021-09724-2
Received:
Accepted:
Published:
DOI: https://doi.org/10.1007/s00332-021-09724-2