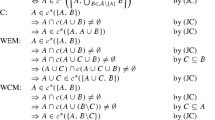Abstract
We prove a coincidence of the class of multi-preference hyper-relations and the class of decent hyper-relations (DHR), that is the class of binary relations on opportunity sets satisfying monotonicity, no-dummy, stability with respect to contraction and extension, and the union property. We study subclasses of DHR. In order to pursue our analysis, we establish a canonical bijection between DHR and the class of no-dummy heritage choice functions. From this we obtain that the no-dummy heritage choice functions have multi-criteria rationalizations with reflexive binary relations. We also prove that the restriction of this bijection to two subclasses of DHR, namely the transitive decent hyper-relations, and the ample hyper-relations, is a bijection between these subclasses and the classes of closed no-dummy choice functions and no-dummy path-independent choice functions (Plott functions), respectively.
Similar content being viewed by others
Notes
It is worth noticing here that the idea to connect hyper-relations and choice functions goes back to Puppe (1996).
Here an arrow \(x\rightarrow z\) means \(x>z\) and so on.
Here, the term ‘specification’ means that the list of comparisons \(a\preceq A\) determines a decent hyper-relation.
The reader can assume for simplicity that \(X\) is a finite set, although the finiteness of \(X\) is almost nowhere used.
Let \(\preceq \) and \(\preceq ^{\prime }\) be two binary relations, we set \(\preceq \subseteq \preceq ^{\prime }\) if, for any \(A\), \(B\subseteq X\), such that \(A\preceq B\), it holds that \( A\preceq ^{\prime }B\).
There are some other reformulation of this axiom. For instance, in the literature on stable matchings (Roth and Sotomayor (1990), Definiton 6.2 p. 173), the Heritage axiom is the Substitutability property: if a worker \(a\) is hired by a firm from a list \(B\) she will be also hired from any shorter list \(A\subseteq B\).
We provide a proof of the latter claim. Suppose that \(A\not \preceq _{f}^{\prime \prime }B\), that is there exists \(a\in f(A\cup B)\) such that \(a\not \in B\). Then, since \(f\) is a heritage choice function and \(a\in A\backslash B\), we obtain that \(a\in f(a\cup B)\) \((a\cup B\subseteq A\cup B\) and \(a\in f(A\cup B)\) ). Hence, \(A\not \preceq _{f}B\), since \(A\preceq _{f}B\) implies \(a\notin f(a\cup B)\)).
The peeling order is a variant of the peeling rank in Statistics. Namely, for a finite set of points \(X\) in an Euclidean space, let us consider the following chain of sets \(X_{0}:=X\), \(X_{1}:=X_{0}\setminus ex(X_{0})\), where \(ex(Y)\) denotes the set of points of \(Y\) which belong to the boundary of its convex hull, \(X_{2}:=X_{1}\setminus ex(X_{1})\), \(\ldots \) . Then, for a subset \(A\subseteq X\) its rank \(r\) is defined as maximal number \( r\) such that \(A\subseteq X_{r}\). The peeling rank is used in non-parametric rank tests [Eddy (1984)].
A mapping \(\mu :2^{X}\rightarrow 2^{X}\) is extensive if \(A\subseteq \mu \left( A\right) \) for all \(A\in 2^{X}\). An extensive mapping is no-dummy if \(\mu (\emptyset )=\emptyset \).
Notice that the transitive decent hyper-relations have been studied in the literature on implication systems with the name of complete implication systems, CIS, see, for example Falmagne and Doignon (2011) and Domenach and Leclerc (2004). Specifically, CIS are the dual of transitive decent relations. In 1974, Armstrong (1974) has shown that CIS are in a one-to-one correspondence with the closure operators. Kreps proves the same result [Lemma 2, Kreps (1979)].
References
Aizerman MA, Malishevskii A (1981) General theory of best variants choice: some aspects. IEEE Trans Autom Control V.AC–26(5):1030–1041
Armstrong WW (1974) Dependency structures of data base relationships. Information Processing 74, North Holland, Amsterdam, 580–583
Caspard N, Monjardet B (2003) The lattice of closure systems, closure operators and implicational systems on a finite set: a survey. Discrete Appl Math 127(2):241–269
Barberà S, Bossert W, Pattanaik PK (2004) Ranking sets of objects. In: Barberà S, Hammond P, Seidl C (eds) Handbook of utility theory, vol 2. Kluwer Academic, Dordrecht
Bourbaki N (1957) Théorie des ensembles. Hermann, Paris
Chernoff H (1954) Rational selection of decision functions. Econometrica 22:422–443
Danilov VI, Koshevoy GA (2005) Mathematics of Plott functions. Math Soc Sci 49:245–272
Danilov VI, Koshevoy GA (2006) New characterization of the path independent choice functions. Math Soc Sci 51:238–245
Danilov VI, Koshevoy GA (2009) Choice functions and extensive operators. Order 26:69–94
Falmagne JP, Doignon JP (2011) Learning spaces. Springer Verlag, New York
Domenach F, Leclerc B (2004) Closure systems, implicational systems, overhanging relations and the case of hierarchical classification. Math Soc Sci 47:349–366
Eddy WF (1984) Set-valued ordering for bivariate data. In: Ambartzumian R, Weil W (eds) Stochastic geometry, geometric statistics, stereology. Teubner, Leipzig, pp 79–90
Kreps DM (1979) Preference for flexibility. Econometrica 47:565–577
Lahiri S (2003) Justifiable preferences over opportunity sets. Soc Choice Welf 21:117–129
Malishevskii AV (1996) Structural characterization of the path independence property by set transformations. In: Diday E, Lechevallier Y, Opitz O (eds) Ordinal ad symbolic data analysis. Springer, Berlin, pp 319–327
Moulin H (1985) Choice functions over a finite set: a summary. Soc Choice Welf 2:147–160
Nehring K, Puppe C (1999) On the multi-preference approach to evaluating opportunities. Soc Choice Welf 14:403–425
Pattanaik PK, Xu Y (1990) On ranking opportunity sets in terms of freedom of choice. Recherches Economiques de Louvain 56:383–390
Plott Ch (1993) Path independence, rationality and social choice. Econometrica 41:1075–1091
Puppe C (1996) An axiomatic approach to ‘preference for freedom of choice’. J Econ Theory 68:174–199
Puppe C, Xu Y (2010) Essential alternatives and freedom rankings. Soc Choice Welf 35:669–685
Roth AE, Sotomayor MAO (1990) Two-sided matching: a study in game-theoretic modeling and analysis. Econometric Society monographs, Cambridge
Ryan M (2014) Path independent choice and the ranking of opportunity sets. Soc Choice Welf 42:193–213
Acknowledgments
We thank referees for useful remarks and suggestions. We also thank Clemens Puppe and the participants of his seminar for useful discussions and remarks.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Danilov, V., Koshevoy, G. & Savaglio, E. Hyper-relations, choice functions, and orderings of opportunity sets. Soc Choice Welf 45, 51–69 (2015). https://doi.org/10.1007/s00355-014-0844-5
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00355-014-0844-5