Abstract
For school choice with affirmative action, responsiveness is used as a measure of how a matching mechanism performs in terms of a certain type of affirmative action policy. We know that the Deferred Acceptance (DA) mechanism is not responsive to the priority-based affirmative action on the universal domain of school choice problems. As a further study, we show in this paper that, the DA mechanism is responsive to the priority-based affirmative action if and only if the exogenous school choice structure (ESCS), which is given by the set of students, the set of schools, the schools’ capacity profile, and schools’ original priority structure, satisfies an acyclicity condition characterized in this paper. This acyclicity condition is stronger than Doğan’s acyclicity, which is the necessary and sufficient condition for the DA mechanism to be responsive to the reserved-based affirmative action.
Similar content being viewed by others
Notes
As Chen and Kesten (2017) pointed out, in recent years, there are about 10 million high school seniors who compete for 6 million seats at universities in China each year. In the college admissions, students’ priority orders at colleges are determined by students’ scores in the National College Entrance Examination.
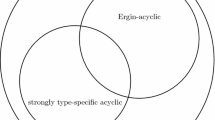
One should note that when we simply mention a “cycle” in this paper, we are actually talking about the cycle characterized by Definition 3. That is, an Ergin’s cycle is not a cycle in this paper.
Specifically, with an improvement for minority students over \(\succ\) in Definition 3, we can achieve a new priority structure \(\succ ^{\prime }\) which satisfies \(s_{k-1}\succ ^{\prime }_{c_1}m\succ ^{\prime }_{c_1}M\succ ^{\prime }_{c_2}s_1\succ ^{\prime }_{c_3}s_2\succ ^{\prime }_{c_4}\cdots \succ ^{\prime }_{c_k}s_{k-1}\). This leads to a generalized cycle.
Alternatively, since there is only one minority student, the responsiveness is actually equivalent to the property of respecting improvement introduced by Balinski and Sönmez (1999). Then, we can infer that the DA mechanism is responsive to the priority-based affirmative action, as Balinski and Sönmez (1999) show that the DA mechanism respects improvement.
Student s can either be \(s^{\prime }\) or some other majority student.
References
Abdulkadiroğlu A (2005) College admission with affirmative action. Int J Game Theory 33:535–549
Abdulkadiroğlu A, Sönmez T (2003) School choice: A mechanism design approach. Am Econ Rev 93(3):729–747
Balinski M, Sönmez T (1999) A tale of two mechanisms: Student placement. J Econ Theory 84(1):73–94
Bó I (2016) Fair implementation of diversity in school choice. Games Econ Behav 97:54–63
Chen Y, Kesten O (2017) Chinese college admissions and school choice reforms: A theoretical analysis. J Political Econ 125(1):99–139
Doğan B (2016) Responsive affirmative action in school choice. J Econ Theory 165:69–105
Echenique F, Yenmez MB (2015) How to control controlled school choice. Am Econ Rev 105(8):2679–2694
Ehlers L, Hafalir IE, Yenmez MB, Yildirim MA (2014) School choice with controlled choice constraints: Hard bounds versus soft bounds. J Econ Theory 153:648–683
Ergin HI (2002) Efficient resource allocation on the basis of priorities. Econometrica 70(6):2489–2497
Fragiadakis D, Troyan P (2017) Improving matching under hard distributional constraints. Theor Econ 12(2):863–908
Gale D, Shapley LS (1962) College admissions and the stability of marriage. Am Math Mon 69(1):9–15
Haeringer G, Klijn F (2009) Constrained school choice. J Econ Theory 144(5):1921–1947
Hafalir IE, Yenmez MB, Yildirim MA (2013) Effective affirmative action in school choice. Theor Econ 8(2):325–363
Hatfield JW, Kojima F, Narita Y (2016) Improving schools through school choice: A market design approach. J Econ Theory 166:186–211
Kesten O (2006) On two competing mechanisms for priority-based allocation problems. J Econ Theory 127(1):155–171
Kesten O (2010) School choice with consent. Quart J Econ 125(3):1297–1348
Klaus B, Klijn F (2013) Local and global consistency properties for student placement. J Math Econ 49(3):222–229
Kojima F (2012) School choice: Impossibilities for affirmative action. Games Econ Behav 75(2):685–693
Kojima F (2013) Efficient resource allocation under multi-unit demand. Games Econ Behav 82:1–14
Acknowledgements
The authors are grateful to an associate editor and an anonymous referee for their constructive comments and helpful suggestions that helped improve the exposition of the paper.
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Appendices
Appendix: Proof of Theorem 1
Only if part
Suppose that \((S,C,\succ ,q)\) has a cycle \((m, M, s_1,\ldots , s_{k-1}, c_1, \ldots ,c_k, N_1,\ldots , N_k)\). Let the students’ preferences be given as follows:
-
(1)
For each \(s \in N_i\) \((i=1,\ldots ,k),\) \(c_i P_s s\) and \(sP_s c\) for all \(c \in C{\setminus } \{c_i\}\);
-
(2)
For each \(s \in S {\setminus } [\{m, M, s_1, \ldots , s_{k-1}\}\cup N_1 \cup \cdots \cup N_k]\), \(s P_s c\) for all \(c \in C;\)
-
(3)
For each \(c \in C{\setminus } \{c_1,c_k\}\), \(s_{k-1}P_{s_{k-1}} c\) and \(c_kP_{s_{k-1}}c_1P_{s_{k-1}}{s_{k-1}}\);
-
(4)
For each \(c \in C{\setminus } \{c_1\}\), \(mP_{m} c\) and \(c_1P_mm\);
-
(5)
For each \(c \in C{\setminus } \{c_1,c_2\}\), \(MP_M c\) and \(c_1P_Mc_2P_MM\);
-
(6)
For each \(i \in \{1,2,\ldots ,k-2\}\), \(c_{i+1} P_{s_i}c_{i+2} P_{s_i} s_i\), and for each \(c \in C {\setminus } \{c_{i+1},c_{i+2}\}\), \(s_i P_{s_i}c\).
\(P_{s_{k-1}}\) | \(P_{m}\) | \(P_{M}\) | \(P_{s_1}\) | \(\cdots\) | \(P_{s_i}\) | \(\cdots\) | \(P_{s_{k-2}}\) |
|---|---|---|---|---|---|---|---|
\(c_k\) | \(c_1\) | \(c_1\) | \(c_2\) | \(\cdots\) | \(c_{i+1}\) | \(\cdots\) | \(c_{k-1}\) |
\(c_1\) | m | \(c_2\) | \(c_3\) | \(\cdots\) | \(c_{i+2}\) | \(\cdots\) | \(c_{k}\) |
\(s_{k-1}\) | \(\vdots\) | M | \(s_1\) | \(\cdots\) | \(s_i\) | \(\cdots\) | \(s_{k-2}\) |
\(\vdots\) | \(\vdots\) | \(\vdots\) | \(\cdots\) | \(\vdots\) | \(\cdots\) | \(\vdots\) |
Since \((m, M, s_1, \ldots , s_{k-1}, c_1, \ldots ,c_k, N_1,\ldots , N_k)\) is a cycle in \((S,C,\succ ,q)\), we know that \(s_{k-1} \succ _{c_1} m\), \(M \succ _{c_1} m\), and \(M \succ _{c_2}s_1\). Moreover, we have either \(s_{k-1} \succ _{c_1} M\), or \(s_{k-1}\in S^m\) and \(M\succ _{c_1}s_{k-1}\). Hence we can define \({\tilde{\succ }}\) as an improvement for minority students over \(\succ\) such that
-
(1)
\(s \ {\tilde{\succ }}_{c_1} \ m \ {\tilde{\succ }}_{c_1} \ M\) for all \(s \in \{s_{k-1}\}\cup N_1\);
-
(2)
\(s \ {\tilde{\succ }}_{c_2} \ s_1\) for all \(s \in \{M\}\cup N_2\);
-
(3)
For each \(i \in \{3,\ldots , k-1\}\), \(s \ {\tilde{\succ }}_{c_i} \ s_{i-1}\) for all \(s \in \{s_{i-2}\}\cup N_i\);
-
(4)
\(s \ {\tilde{\succ }}_{c_k} \ s_{k-1}\) for all \(s \in \{s_{k-2}\}\cup N_k\).
Let \(G=(P,\succ )\) be a problem without affirmative action, and problem \({\tilde{G}}=(P,{\tilde{\succ }})\) has a stronger priority-based affirmative action policy than G. Let \(\mu =\text{ DA }(G)\) and \({\tilde{\mu }}=\text{ DA }({\tilde{G}})\). Then it is easy to see that \(\mu ^{-1}(c_1)=\{M\}\cup N_1\), \(\mu ^{-1}(c_i)=\{s_{i-1}\}\cup N_i\) for all \(i \in \{2,\ldots , k\}\), and \(\mu ^{-1}(c)=\emptyset\) for all \(c \in C {\setminus } \{c_1,\ldots ,c_k\}\). For \({\tilde{\mu }}\), we have \({\tilde{\mu }}^{-1}(c_1)=\{s_{k-1}\}\cup N_1\), \({\tilde{\mu }}^{-1}(c_2)=\{M\}\cup N_2\), \({\tilde{\mu }}^{-1}(c_i)=\{s_{i-2}\}\cup N_i\) for all \(i \in \{3,\ldots , k\}\), and \({\tilde{\mu }}^{-1}(c)=\emptyset\) for all \(c \in C {\setminus } \{c_1,\ldots ,c_k\}\). Obviously, \(\mu (s) P_s {\tilde{\mu }}(s)\) for all \(s \in \{s_1,s_2,\ldots ,s_{k-1}\}\) and \(\mu (s) R_s {\tilde{\mu }}(s)\) for all \(s \in S\). Thus, although \({\tilde{G}}\) has a stronger priority-based affirmative action policy than G, \(\text{ DA }({\tilde{G}})\) is Pareto inferior to \(\text{ DA }(G)\) for the minority, as \(\{s_1,\ldots ,s_{k-1}\}\cap S^m \ne \emptyset\). Then the DA mechanism is not responsive to the priority-based affirmative action. This part is completed. \(\square\)
If part
1.1 Definitions
In this subsection, we need to introduce the notions of interrupters,Footnote 8rejection-chains, and rejection-cycles. All of these notions are very important to our proof.
Definition 5
For a problem G, a student \(\varvec{s}\) is an interrupter at school \(\varvec{c}\) in the DA algorithm if there exist two steps, Step \(k_1\) and Step \(k_2\) (\(k_1 < k_2\)), such that (a) at Step \(k_1\), there exists \(s^{\prime }\) who is rejected by c, while s is tentatively accepted by c; and (b) at Step \(k_2\), s is rejected by c. We call (s, c) an interrupting pair. For a problem G, a student \(\varvec{s}\) is a minority interrupter at \(\varvec{c}\) in the DA algorithm if \(s \in S^m\) and s is an interrupter at c in the DA algorithm. We call (s, c) a minority interrupting pair.
With the notion of minority interrupting pairs, we are now ready to introduce the notion of a rejection-chain. A rejection-chain in the DA algorithm is a series of rejections, initiated by a minority interrupting pair and also ended by a minority interrupting pair. Formally, we provide the following definition.
Definition 6
A list \(A=\big ((m,c),(m^{\prime },c^{\prime });s_1,s_2,\ldots ,s_n;c_1,c_2,\ldots,c_{n-1}\big )\) is a rejection-chain in the DA algorithm for \(G=(P,\rhd )\) if both (m, c) and \((m^{\prime },c^{\prime })\) are minority interrupting pairs and there exist \(k,k_1,k_2,\ldots ,k_{n-1},k^{\prime }\in \mathbb {N}\) such that \(k<k_1<k_2<\cdots<k_{n-1}<k^{\prime }\), and (a) at Step k of the DA algorithm, minority student m is tentatively accepted by c but some student \(s_1\) is rejected by c, and (b) for each \(i\in \{1,2,\ldots ,n-1\}\), at Step \(k_i\), student \(s_i\) is tentatively accepted by \(c_i\) but student \(s_{i+1}\) is rejected by \(c_i\), and (c) at Step \(k^{\prime }\), student \(s_n\) is tentatively accepted by \(c^{\prime }\) but minority student \(m^{\prime }\) is rejected by \(c^{\prime }\).
Then, the minority interrupting pair (m, c) is called the starting point of the rejection-chain A, and \((m^{\prime },c^{\prime })\) is called the ending point of A, and students \(s_1\), \(s_2\), \(\cdots\), \(s_n\) are called blocked students.
Figure 1 is provided to illustrate the notion of a rejection-chain.
An essential chain is a special type of rejection-chain, which requires that the first blocked student in the rejection-chain is a majority one, and there is at least one minority student among the blocked students. We will show in Lemma 6 that a rejection-cycle containing an essential chain in the DA algorithm is an essential signal implying a cycle in the priority structure. The formal definitions of an essential chain and a rejection-cycle are given below.
Definition 7
The list \(A=\big ((m,c),(m^{\prime },c^{\prime });s_1,s_2,\ldots ,s_n;c_1,c_2,\ldots ,c_{n-1}\big )\) is a rejection-chain in the DA algorithm for problem G. Then, A is an essential chain in the DA algorithm for problem G if \(s_1 \in S^M\) and \(\{s_2,\ldots ,s_n\} \cap S^m \ne \emptyset\).
Definition 8
For a problem G, a rejection-cycle in the DA algorithm is a finite set of rejection-chains \(\Omega =\{A_1,A_2,\ldots ,A_t\}\), where \(t \ge 1\), and each \(A_i \in \Omega\) is a rejection-chain in the DA algorithm for problem G, and the ending point of \(A_i\) is the starting point of \(A_{i+1}\) for all \(i \in \{1,2,\ldots ,t-1\}\), and the ending point of \(A_t\) is the starting point of \(A_1\).
1.2 Lemmas
Lemma 1
If \({\tilde{G}}=(P,{\tilde{\rhd }})\) has a stronger priority-based affirmative action policy than \(G=(P,\rhd )\), and \({\tilde{\mu }}=\text{ DA }({\tilde{G}})\) is Pareto inferior to \(\mu =\text{ DA }(G)\) for the minority, then \({\tilde{\mu }}\) is Pareto inferior to \(\mu\) for all students.
Proof of Lemma 1
The proof is inspired by Doğan (2016). We argue by contradiction. Suppose not, then there exists some \(M \in S^M\) such that \({\tilde{\mu }}(M) P_M \mu (M)\). Let \(c \equiv \mu (M)\) and \(c_1\equiv {\tilde{\mu }}(M)\).
Step 1. Since \(c_1P_Mc\) and \(\mu (M)=c\), there is some step of the DA algorithm for problem G, say \(k_1\), at which M is rejected by \(c_1\). Note that at this step the capacity of \(c_1\) is exhausted. Since \(M \in {\tilde{\mu }}^{-1}(c_1)\), there is a student \(s \in S{\setminus }{\tilde{\mu }}^{-1}(c_1)\) who is temporarily accepted by \(c_1\) at Step \(k_1\) of the DA algorithm for problem G.
We claim that all of such students are majority students. To see this, suppose that \(s \in S^m\). Note that since \({\tilde{\mu }}\) is Pareto inferior to \(\mu\) for the minority, \(\mu (s) R_s {\tilde{\mu }}(s)\). Also, since s is temporarily accepted by \(c_1\) at a step of the DA algorithm for problem G, we have \(c_1R_s\mu (s)\). Thus, we infer \(c_1R_s{\tilde{\mu }}(s)\), which, together with \(s \notin {\tilde{\mu }}^{-1}(c_1)\), implies \(c_1 P_s {\tilde{\mu }}(s)\). According to \(c_1 P_s {\tilde{\mu }}(s)\), \(M \in {\tilde{\mu }}^{-1}(c_1)\) and the stability of \({\tilde{\mu }}\), one can obtain that \(M {\tilde{\rhd }}_{c_1} s\). Since \({\tilde{\rhd }}_{c_1}\) is an improvement for minority students over \(\rhd _{c_1}\), we get \(M \rhd _{c_1} s\), which contradicts that \(c_1\) rejects M and temporarily accepts s at Step \(k_1\) of the DA algorithm for problem G.
We choose one such student, say, \(M_1\). Then \(M_1 \rhd _{c_1} M\) and \(M_1 \notin {\tilde{\mu }}^{-1}(c_1)\). By \(M_1 \rhd _{c_1} M\) we have \(M_1 {\tilde{\rhd }}_{c_1} M\), which, together with \(M_1 \notin {\tilde{\mu }}^{-1}(c_1)\) and \(M \in {\tilde{\mu }}^{-1}(c_1)\), implies \({\tilde{\mu }}(M_1) P_{M_1} c_1\). It is easy to see that \(c_1 R_{M_1}\mu (M_1)\). Thus, we have \({\tilde{\mu }}(M_1) P_{M_1} \mu (M_1)\).
Step 2. Let \(c_2 \equiv {\tilde{\mu }}(M_1)\). By the same arguments as in Step 1, there is \(M_2 \in S^M {\setminus } {\tilde{\mu }}^{-1}(c_2)\) such that there is a step of the DA algorithm for problem G, say \(k_2\), at which \(M_1\) is rejected by \(c_2\) and \(M_2\) is temporarily accepted by \(c_2\) at the same step. Now, since \(c_2 P_{M_1}c_1\), \(k_2<k_1\). Continuing in this way, eventually we have \(c_n \in C\) and \(M_{n-1}, M_n \in S^M\) such that at Step 1 of the DA algorithm for problem G, \(M_{n-1}\) is rejected by \(c_n\), \(M_n\) is temporarily accepted by \(c_n\), and \(M_n \notin {\tilde{\mu }}^{-1}(c_n), M_{n-1}\in {\tilde{\mu }}^{-1}(c_n)\). Note that \(c_n\) is the most preferred school of \(M_n\), and \(M_n \rhd _{c_n} M_{n-1}\) implies \(M_n {\tilde{\rhd }}_{c_n} M_{n-1}\). Yet \(M_n \notin {\tilde{\mu }}^{-1}(c_n), M_{n-1}\in {\tilde{\mu }}^{-1}(c_n)\), contradicting the stability of \({\tilde{\mu }}\). The proof is completed. \(\square\)
Lemma 2
If \({\tilde{G}}=(P,{\tilde{\rhd }})\) has a stronger priority-based affirmative action policy than \(G=(P,\rhd )\), and \({\tilde{\mu }}=\text{ DA }({\tilde{G}})\) is Pareto inferior to \(\mu =\text{ DA }(G)\) for the minority, then for each \(c \in C\), \(|\mu ^{-1}(c)|=|{\tilde{\mu }}^{-1}(c)|=q_c\) whenever \(\mu ^{-1}(c)\ne {\tilde{\mu }}^{-1}(c)\).
Proof of Lemma 2
By Lemma 1, we have \(\mu (s) R_s {\tilde{\mu }}(s)\) for all \(s \in S\). According to \(\mu (s) R_s {\tilde{\mu }}(s)\) for all \(s \in S\) and the stability of \({\tilde{\mu }}\), if there is \(s \in \mu ^{-1}(c) {\setminus } {\tilde{\mu }}^{-1}(c)\), then \(|{\tilde{\mu }}^{-1}(c)|=q_c\). Thus for each \(c \in C\), if \(\mu ^{-1}(c) {\setminus } {\tilde{\mu }}^{-1}(c)\ne \emptyset\), then \(|{\tilde{\mu }}^{-1}(c) {\setminus } \mu ^{-1}(c)| \ge |\mu ^{-1}(c) {\setminus } {\tilde{\mu }}^{-1}(c)|\). For \(c \in C\), if \(\mu ^{-1}(c) {\setminus } {\tilde{\mu }}^{-1}(c)= \emptyset\), it is obvious to infer \(|{\tilde{\mu }}^{-1}(c) {\setminus } \mu ^{-1}(c)| \ge |\mu ^{-1}(c) {\setminus } {\tilde{\mu }}^{-1}(c)|\). Thus we have \(|{\tilde{\mu }}^{-1}(c) {\setminus } {\mu }^{-1}(c)| \ge |\mu ^{-1}(c) {\setminus } {\tilde{\mu }}^{-1}(c)|\) for all \(c \in C\). Suppose that there exists \(c \in C\) such that \(|{\tilde{\mu }}^{-1}(c) {\setminus } {\mu }^{-1}(c)| > |\mu ^{-1}(c) {\setminus } {\tilde{\mu }}^{-1}(c)|\). Then there exists at least one student \(s \in S\) such that \(\mu (s)=s\) and \({\tilde{\mu }}(s) \in C\). Since we have obtained that \(\mu (s^{\prime }) R_{s^{\prime }} {\tilde{\mu }}(s^{\prime })\) for all \(s^{\prime } \in S\), one can infer that \(sP_s {\tilde{\mu }}(s)\), which contradicts the individual rationality of \(\mu ^{\prime }\). Therefore, we have \(|{\tilde{\mu }}^{-1}(c) {\setminus } {\mu }^{-1}(c)|= |\mu ^{-1}(c) {\setminus } {\tilde{\mu }}^{-1}(c)|\)for all \(c \in C\). If \(\mu ^{-1}(c)\ne {\tilde{\mu }}^{-1}(c)\), then one can infer that \(|{\tilde{\mu }}^{-1}(c) {\setminus } \mu ^{-1}(c)| = |\mu ^{-1}(c) {\setminus } {\tilde{\mu }}^{-1}(c)|\ge 1\), and consequently \(\mu ^{-1}(c) {\setminus } {\tilde{\mu }}^{-1}(c)\ne \emptyset\). Then we have \(|{\tilde{\mu }}^{-1}(c)|=q_c\). By \(|{\tilde{\mu }}^{-1}(c) {\setminus } \mu ^{-1}(c)| = |\mu ^{-1}(c) {\setminus } {\tilde{\mu }}^{-1}(c)|\) we obtain \(|\mu ^{-1}(c)|=q_c\). \(\square\)
Lemma 3
If \({\tilde{G}}=(P,{\tilde{\rhd }})\) has a stronger priority-based affirmative action policy than \(G=(P,\rhd )\), and \({\tilde{\mu }}=\text{ DA }({\tilde{G}})\) is Pareto inferior to \(\mu =\text{ DA }(G)\) for the minority, then \(\sum _{c \in C}|\mu ^{-1}(c)|=\sum _{c \in C}|{\tilde{\mu }}^{-1}(c)|\).
Proof of Lemma 3
For each \(c \in C,\) it satisfies either \(\mu ^{-1}(c)={\tilde{\mu }}^{-1}(c)\) or \(\mu ^{-1}(c)\ne {\tilde{\mu }}^{-1}(c)\). If \(\mu ^{-1}(c)={\tilde{\mu }}^{-1}(c),\) then \(|\mu ^{-1}(c)|=|{\tilde{\mu }}^{-1}(c)|\). If \(\mu ^{-1}(c)\ne {\tilde{\mu }}^{-1}(c)\), by Lemma 2 we get \(|\mu ^{-1}(c)|=|{\tilde{\mu }}^{-1}(c)|=q_c\). The proof is then completed. \(\square\)
Lemma 4
If \({\tilde{G}}=(P,{\tilde{\rhd }})\) has a stronger priority-based affirmative action policy than \(G=(P,\rhd )\), and \({\tilde{\mu }}=\text{ DA }({\tilde{G}})\) is Pareto inferior to \(\mu =\text{ DA }(G)\) for the minority, then \(\cup _{c \in C}{\tilde{\mu }}^{-1}(c) =\cup _{c \in C}\mu ^{-1}(c)\).
Proof of Lemma 4
For each \(s \in S\), if \({\tilde{\mu }}(s) \in C\), then by Lemma 1 we get \(\mu (s) R_s {\tilde{\mu }}(s)\). Since \({\tilde{\mu }}\) is individually rational, we have \({\tilde{\mu }}(s) P_s s\). Thus, we infer that \(\mu (s) P_s s\) and \(\mu (s) \in C\). On the other hand, for each \(s \in S\), if \(\mu (s) \in C\), we claim that \({\tilde{\mu }}(s) \in C\). Specifically, if \(\mu (s) \in C\) but \({\tilde{\mu }}(s) \notin C\), by Lemma 3 one can infer that there is at least one student, say \(s^{\prime } \in S\), such that \({\tilde{\mu }}(s^{\prime }) \in C\), but \(\mu (s^{\prime })=s^{\prime }\). Since \({\tilde{\mu }}\) is individually rational, we have \({\tilde{\mu }}(s^{\prime }) P_s \mu (s^{\prime })\), which contradicts the conclusion of Lemma 1. The proof is completed. \(\square\)
Lemma 5
If \({\tilde{\succ }}\) is an improvement for minority students over \(\succ\), and \((S,C,{\tilde{\succ }},q)\) has a cycle, then \((S,C,\succ ,q)\) has a cycle.
Proof of Lemma 5
Let \((m,M,s_1,\ldots ,s_{k-1};c_1,\ldots ,c_k;N_1,\ldots ,N_k)\) be a cycle of \((S,C,{\tilde{\succ }},q)\). We show that it is also a cycle of \((S,C,\succ ,q)\).
According to definition of a cycle, we have \(M \ {\tilde{\succ }}_{c_1}m\), \(s_{k-1}{\tilde{\succ }}_{c_1}m\), and \(M \ {\tilde{\succ }}_{c_2}s_1\), and if \(s_{k-1}\in S^M\), then \(s_{k-1}{\tilde{\succ }}_{c_1}M\). Then since \(M\in S^M\) and \(m\in S^m\), we can infer that \(M\succ _{c_1}m\), \(s_{k-1}\succ _{c_1}m\), and \(M\succ _{c_2}s_1\), and if \(s_{k-1}\in S^M\), then \(s_{k-1}\succ _{c_1}M\).
Next, we only need to show that for any \(s,s^{\prime }\in \{m,M,s_1,\ldots ,s_{k-1}\}\), any \(c\in \{c_1,\ldots ,c_k\}\), and any \(N\in \{N_1,\ldots ,N_k\}\), if \((s,N) \in T(s^{\prime },c)\) for \((S,C,{\tilde{\succ }},q)\), then we also have \((s,N) \in T(s^{\prime },c)\) for \((S,C,\succ ,q)\). There are two cases:
-
(1)
If \(s^{\prime } \in S^m\), since \({\tilde{\succ }}\) is an improvement for minority students over \(\succ\), then for any student \(s_0\) satisfying \(s_0{\tilde{\succ }}_c s^{\prime }\), there is \(s_0\succ _c s^{\prime }\). Thus, \((s,N) \in T(s^{\prime },c)\) for \((S,C,{\tilde{\succ }},q)\) implies \((s,N) \in T(s^{\prime },c)\) for \((S,C,\succ ,q)\).
-
(2)
If \(s^{\prime } \in S^M\), then for any student \(s_0 \in S^M\) satisfying \(s_0 {\tilde{\succ }}_c s^{\prime }\), there is also \(s_0 \succ _c s^{\prime }\). Thus, \((s,N) \in T(s^{\prime },c)\) for \((S,C,{\tilde{\succ }},q)\) still implies \((s,N) \in T(s,c)\) for \((S,C,\succ ,q)\).
The proof is then completed. \(\square\)
Lemma 6
Suppose that \({\tilde{G}}=(P,{\tilde{\rhd }})\) has a stronger priority-based affirmative action policy than \(G=(P,\rhd )\), and \(\Omega =\{A_0,A_1,A_2,\ldots ,A_t\}\) is a rejection-cycle in the DA algorithm for problem \({\tilde{G}}\), where \(t \ge 0\), and \(A_0\) is an essential chain. Suppose that for each \(i \in \{0,1,2,\ldots ,t\}\), \(A_i=\big ((m_i,c_i),(m_{i+1},c_{i+1});s^1_i,s^2_i,\ldots ,s^{u_i}_i;c^1_i,c^2_i,\ldots ,\ldots ,c^{u_i-1}_i\big )\), where \(m_0=m_{t+1}\) and \(c_0=c_{t+1}\). If \(s_0^1\rhd _{c_0}m_0\), then \((S,C,\rhd ,q)\) has a cycle.
Proof of Lemma 6
Since \(A_0\) is an essential chain, we have \(s^1_0 \in S^M\), and there exists some \(j \in \{2,\ldots ,u_0\}\) such that \(s^j_0 \in S^m\). Now we consider the DA algorithm for problem \({\tilde{G}}\). Let \({\tilde{\mu }}\equiv\) DA\(({\tilde{G}})\). Since each school in each rejection-chain must have rejected some student in the DA algorithm, we obtain that, for each \(i \in \{0,1,\ldots ,t\}\),
-
(i)
there is \(|{\tilde{\mu }}^{-1}(c_i)|=q_{c_i}\); and
-
(ii)
for each \(j \in \{1,2,\ldots ,u_i-1\}\), there is \(|{\tilde{\mu }}^{-1}(c^j_i)|=q_{c^j_i}\).
Then, there exist \(u_0+u_1+u_2+\cdots +u_t\) sets of students \(N_0\), \(N_1\), \(\cdots\), \(N_t\), \(N^1_0\), \(N^2_0\), \(\cdots\), \(N^{u_0-1}_0\), \(N^1_1\), \(N^2_1\), \(\cdots\), \(N^{u_1-1}_1\), \(N^1_2\), \(N^2_2\), \(\cdots\), \(N^{u_2-1}_2\), \(\cdots\), \(N^1_t\), \(N^2_t\), \(\cdots\), \(N^{u_t-1}_t\), such that for each \(i \in \{0,1,\ldots ,t\}\),
-
(i)
\(|N_i|=q_{c_i}-1\) and \(N_{i+1} \subseteq {\tilde{\mu }}^{-1}(c_{i+1}) {\setminus } \{s^{u_i}_i\}\) (\(N_0=N_{t+1}\)); and
-
(ii)
\(|N^j_i|=q_{c^j_i}-1\) and \(N^j_i \subseteq {\tilde{\mu }}^{-1}(c^j_i) {\setminus } \{s^j_i\}\) for each \(j \in \{1,2,\ldots ,\ldots ,u_i-1\}\).
Since \({\tilde{\mu }}\) is a stable matching, we obtain that, for each \(i \in \{0,1,\ldots ,t\}\),
-
(i)
there is \((m_i,N_i) \in T(s^1_i,c_i)\) and \((s^{u_i}_i,N_{i+1}) \in T(m_{i+1},c_{i+1})\); and
-
(ii)
for each \(j \in \{1,2,\ldots ,u_i-1\}\), there is \((s^j_i,N^j_i) \in T(s^{j+1}_i,c^j_i)\).
Moreover, since \({\tilde{\mu }}\) is a stable matching, and for each \(i \in \{0,1,\ldots ,t\}\), we have \((s^{u_i}_i,N_{i+1}) \in T(m_{i+1},c_{i+1})\) and \((m_{i+1},N_{i+1}) \in T(s^1_{i+1},c_{i+1})\), we can obtain \((s^{u_i}_i,N_{i+1}) \in T(s^1_{i+1},c_{i+1})\) for each \(i \in \{0,1,\ldots ,t\}\).
Then, we have \((m_0,N_0) \in T(s^1_0,c_0)\), \((s^1_0,N^1_0) \in T(s^2_0,c^1_0)\), \(\cdots\), \((s^{u_0-1}_0,N^{u_0-1}_0) \in T(s^{u_0}_0,c^{u_0-1}_0)\), \((s^{u_0}_0,N_1) \in T(s^1_1,c_1)\), \((s^1_1,N^1_1) \in T(s^2_1,c^1_1)\), \(\cdots\), \((s^{u_1-1}_1,N^{u_1-1}_1) \in T(s^{u_1}_1,c^{u_1-1}_1)\), \((s^{u_1}_1,N_2) \in T(s^1_2,c_2)\), \(\cdots\), \((s^{u_{t-1}}_{t-1},N_t) \in T(s^1_t,c_t)\), \((s^1_t,N^1_t) \in T(s^2_t,c^1_t)\), \(\cdots\), \((s^{u_t-1}_t,N^{u_t-1}_t) \in T(s^{u_t}_t,c^{u_t-1}_t)\), \((s^{u_t}_t,N_0) \in T(m_0,c_0)\). As we have shown in the proof of Lemma 5, since these results hold for problem \({\tilde{G}}\), they also hold for problem G.
Finally, according to the definition of rejection-chains, we have \(s_t^{u_t}{\tilde{\rhd }}_{c_0}m_0{\tilde{\rhd }}_{c_0}s^1_0\). This implies that \(s_t^{u_t}\rhd _{c_0}m_0\), and if \(s_t^{u_t}\in S^M\), then \(s_t^{u_t}\rhd _{c_0}s^1_0\). Since we also have \(s_0^1\rhd _{c_0}m_0\), we conclude that the list \((m_0,s^1_0,s^2_0,\ldots ,s^{u_0}_0,s^1_1,\ldots ,s^{u_1}_1,\ldots ,s^{u_t}_t;c_0,c^1_0,c^2_0,\ldots ,c^{u_0-1}_0,c_1,c^1_1,c^2_1,\ldots ,c^{u_1-1}_1,c_2, \ldots ,\) \(c^{u_t-1}_t;N_0,N^1_0,N^2_0,\ldots ,N^{u_0-1}_0,N_1,N^1_1,N^2_1, \ldots ,N^{u_1-1}_1,N_2,\ldots ,N^{u_t-1}_t)\) is a cycle of length \(u_0+u_1+u_2+\cdots +u_t\). The proof is completed. \(\square\)
1.3 Proof
Now we start proving the theorem. Suppose that \((S,C,\succ ,q)\) is acyclic. We want to show that the DA mechanism is responsive to the priority-based affirmative action. Suppose by contradiction that the DA mechanism is not responsive to the priority-based affirmative action. Then there are two problems \(G=(P,\rhd )\) and \({\tilde{G}}=(P,{\tilde{\rhd }})\) such that \(\rhd\) is a weak improvement for minority students over \(\succ\), and \({\tilde{G}}\) has a stronger priority-based affirmative action policy than G, and \({\tilde{\mu }}=\text{ DA }({\tilde{G}})\) is Pareto inferior to \(\mu =\text{ DA }(G)\) for the minority.
The following proof is divided into two parts. First, we show that there is an essential chain in the DA algorithm for problem \({\tilde{G}}\) (Step 1 and Step 2). Then, we show that the essential chain that we find is in a rejection-cycle (Step 3). Then, we find that Lemma 6 can be applied to this rejection-cycle, which implies that \((S,C,\rhd ,q)\) has a cycle. Since \(\rhd\) is an improvement for minority students over \(\succ\), we can further obtain that \((S,C,\succ ,q)\) has a cycle by Lemma 5. This contradicts the acyclicity of \((S,C,\succ ,q)\) and completes the proof.
Step 1.
Since \({\tilde{\mu }}\) is Pareto inferior to \(\mu\) for the minority, there exists some minority student, say \(s^m\), such that \(\mu (s^m) P_{s^m} {\tilde{\mu }}(s^m)\). By Lemma 4, we have \(\mu (s^m) \in C\) and \({\tilde{\mu }}(s^m) \in C\). Let \({c}_0\equiv \mu (s^m)\) and \(\tilde{{c}}_1\equiv {\tilde{\mu }}(s^m)\). Since \(s^m \in \mu ^{-1}({c}_0)\) and \(s^m \in {\tilde{\mu }}^{-1}(\tilde{{c}}_1)\), one can infer that \({\tilde{\mu }}^{-1}({c}_0) \ne \mu ^{-1} ({c}_0)\) and \({\tilde{\mu }}^{-1}(\tilde{{c}}_1) \ne \mu ^{-1} (\tilde{{c}}_1)\). By Lemma 2 we have \(|{\tilde{\mu }}^{-1}({c}_0)| =| \mu ^{-1} ({c}_0)|=q_{{c}_0}\) and \(|{\tilde{\mu }}^{-1}(\tilde{{c}}_1)| = |\mu ^{-1} (\tilde{{c}}_1)|=q_{\tilde{{c}}_1}\). Suppose that at Step \({\tilde{k}}_1\) of the DA algorithm for \({\tilde{G}}\), student \(s^m\) proposes to school \(\tilde{{c}}_1\).
If there exists some step of the DA algorithm for \({\tilde{G}}\), say \(k_1^{\prime } \ge {\tilde{k}}_1\), such that at this step school \(\tilde{{c}}_1\) rejects some student, say \(\tilde{{s}}_1\), satisfying \(\mu (\tilde{{s}}_1) R_{\tilde{{s}}_1} \tilde{{c}}_1\), then we obtain \(\tilde{{c}}_1 P_{\tilde{{s}}_1} {\tilde{\mu }}(\tilde{{s}}_1)\) and \(\mu (\tilde{{s}}_1) P_{\tilde{{s}}_1} {\tilde{\mu }}(\tilde{{s}}_1)\). Then, by Lemma 4, we can see that \({\tilde{\mu }}(\tilde{{s}}_1) \in C\). Let \(\tilde{{c}}_2 \equiv {\tilde{\mu }}(\tilde{{s}}_1)\). Suppose that at Step \({\tilde{k}}_2\) \(({\tilde{k}}_2 > k_1^{\prime })\) of the DA algorithm for \({\tilde{G}}\), student \(\tilde{{s}}_1\) proposes to school \(\tilde{{c}}_2\).
Again, if there exists some step of the DA algorithm for \({\tilde{G}}\), say \(k_2^{\prime } \ge {\tilde{k}}_2\), such that at this step school \(\tilde{{c}}_2\) rejects some student, say \(\tilde{{s}}_2\), satisfying \(\mu (\tilde{{s}}_2) R_{\tilde{{s}}_2} \tilde{{c}}_2\), then we repeat the above procedures and can obtain that at some step of the DA algorithm for \({\tilde{G}}\), say Step \({\tilde{k}}_3\) (\({\tilde{k}}_3 > k_2^{\prime }\)), student \(\tilde{{s}}_2\) proposes to school \(\tilde{{c}}_3\), where \(\tilde{{c}}_3 \equiv {\tilde{\mu }}(\tilde{{s}}_2)\).
After repeating the above procedures for several times, since the DA algorithm terminates in finite steps, there must exist some step, say Step \({\tilde{k}}_t\) (\(t \ge 1\)), such that, at Step \({\tilde{k}}_t\), student \({\tilde{s}}_{t-1}\) proposes to school \(\tilde{{c}}_t\), where \(\tilde{{c}}_t \equiv {\tilde{\mu }}({\tilde{s}}_{t-1})\) (\({\tilde{s}}_{t-1}\) should be \(s^m\) if \(t=1\)), and from Step \({\tilde{k}}_t\) to the final step of the DA algorithm for \({\tilde{G}}\), school \(\tilde{{c}}_t\) does not reject any student \(s \in S\) satisfying \(\mu (s) R_{s} \tilde{{c}}_t\). Then by \(|{\tilde{\mu }}^{-1}(\tilde{{c}}_t)| = |\mu ^{-1}(\tilde{{c}}_t)|=q_{\tilde{{c}}_t}\) one can infer that, in the DA algorithm for \({\tilde{G}}\), \(\tilde{{c}}_t\) rejects some student \(s^{\prime } \in \mu ^{-1}(\tilde{{c}}_t)\) before Step \({\tilde{k}}_t\), which implies that \(\tilde{{c}}_t\) has already temporarily accepted \(q_{\tilde{{c}}_t}\) students at the end of Step \({\tilde{k}}_t-1\).
Let \({\tilde{S}}\) denote the set of students who are temporarily accepted by \({\tilde{c}}_t\) at the end of Step \({\tilde{k}}_t-1\) of the DA algorithm for \({\tilde{G}}\). By \({\tilde{s}}_{t-1} \in {\tilde{\mu }}^{-1}(\tilde{{c}}_t)\), we infer that, at Step \({\tilde{k}}_t\), \(\tilde{{c}}_t\) temporarily accepts \({\tilde{s}}_{t-1}\) and rejects some student \({{\tilde{s}}_t} \in {\tilde{S}}\) satisfying \(\tilde{{c}}_t P_{{\tilde{s}}_t} \mu ({{\tilde{s}}_t})\). Since \(\mu\) is stable, we infer that \(s {\rhd }_{\tilde{{c}}_t} {{\tilde{s}}_t}\) for all \(s \in \mu ^{-1}(\tilde{{c}}_t)\) and \({s^{\prime }} \rhd _{\tilde{{c}}_t} {\tilde{s}}_t\). However, by the above arguments that \({\tilde{s}}_t \in {\tilde{S}}\) and \({\tilde{s}}_t\) is rejected by \({\tilde{c}}_t\) at a step later than the step at which \(s^{\prime }\) is rejected by \({\tilde{c}}_t\), we can get \({{\tilde{s}}_t} {\tilde{\rhd }}_{\tilde{{c}}_t} s^{\prime }\). Then we obtain that \({\tilde{\rhd }}_{\tilde{{c}}_t}\) improves the priority of \({\tilde{s}}_t\) over \(\rhd _{\tilde{{c}}_t}\). Thus, we have \({{\tilde{s}}_t} \in S^m\) and \(s^{\prime } \in S^M\), and we find that \({\tilde{s}}_t\) is a minority interrupter at \({\tilde{c}}_t\) in the DA algorithm for \({\tilde{G}}\).
Now, we get a series of rejections in the DA algorithm for \({\tilde{G}}\). Specifically, at some step of the DA algorithm for \({\tilde{G}}\), \(s^m\) is rejected by school \(c_0=\mu (s^m)\). At Step \(k^{\prime }_1\), student \(s^m\) is accepted by \({\tilde{c}}_1={\tilde{\mu }}(s^m)\) but student \({\tilde{s}}_1\) is rejected by \({\tilde{c}}_1\). At Step \(k^{\prime }_2\), student \({\tilde{s}}_1\) is accepted by \({\tilde{c}}_2={\tilde{\mu }}({\tilde{s}}_1)\) but student \({\tilde{s}}_2\) is rejected by \({\tilde{c}}_2\). \(\cdots\) At Step \(k^{\prime }_{t-1}\), \({\tilde{s}}_{t-2}\) is accepted by \({\tilde{c}}_{t-1}={\tilde{\mu }}({\tilde{s}}_{t-2})\) but \({\tilde{s}}_{t-1}\) is rejected by \({\tilde{c}}_{t-1}\), and at Step \({\tilde{k}}_t\), \({\tilde{s}}_{t-1}\) proposes to \({\tilde{c}}_t={\tilde{\mu }}({\tilde{s}}_{t-1})\) and is accepted, while student \({\tilde{s}}_t\) is rejected by \({\tilde{c}}_t\). Moreover, at some step before Step \({\tilde{k}}_t\), student \(s^{\prime }\) satisfying \(s^{\prime } \in S^M\) and \(\mu (s^{\prime })={\tilde{c}}_t\) is rejected by \(\tilde{{c}}_t\).
Let \(m^{\prime } \equiv {\tilde{s}}_t\) and \(c^{\prime } \equiv {\tilde{c}}_t\). The rejection process can be illustrated by Fig. 2.
Step 2.
Since \(s^m \in {\mu }^{-1} ({c}_0) {\setminus }{\tilde{\mu }}^{-1}({c}_0)\) and \({c}_0 P_{s^m} {\tilde{\mu }}(s^m)\), there exists a student, say \({s}_1\), such that \({s}_1 \notin \mu ^{-1}({c}_0)\) and \({c}_0\) temporarily accepts \({s}_1\) and rejects \(s^m\) at some step, say Step \(k_0\) (\(k_0<{\tilde{k}}_1\)), of the DA algorithm for \({\tilde{G}}\). It is easy to see that \({s}_1 {\tilde{\rhd }}_{{c}_0} s^m\). Since \(s^m\) is a minority student, we have \({s}_1 \rhd _{{c}_0} s^m\). Then by the stability of \(\mu\), since \(s_1 \notin \mu ^{-1}({c}_0)\) and \(s^m \in \mu ^{-1}({c}_0)\), we can infer that \(\mu (s_1) P_{s_1} {c}_0\). Let \(c_1 \equiv \mu ({s}_1)\).
Since \(c_1 P_{s_1} {c}_0\) and \(s_1\) has ever proposed to \(c_0\), there exists some step, say Step \({k}_1\) \(({k}_1<k_0)\), such that \(s_1\) is rejected by \(c_1\) at Step \({k}_1\) of the DA algorithm for \({\tilde{G}}\). By a similar argument as above, we infer that there exists some student, say \({s}_2 \in S\), such that \({s}_2 \notin \mu ^{-1}(c_1)\) and \({c}_1\) temporarily accepts \({s}_2\) at Step \({k}_1\) of the DA algorithm for \({\tilde{G}}\). One can infer that \({s}_2 {\tilde{\rhd }}_{{c}_1} s_1\). Let \(c_2 \equiv \mu ({s}_2)\).
If \(c_2 P_{s_2} {c}_1\), then we can repeat the above procedures. Specifically, since \({c}_1\) temporarily accepts \({s}_2\) at Step \({k}_1\) of the DA algorithm for \({\tilde{G}}\), there exists some step, say Step \({k}_2\) \(({k}_2<{k}_1)\), such that \(s_2\) is rejected by \(c_2\) at Step \({k}_2\) of the DA algorithm for \({\tilde{G}}\), and there exists some student, say \(s_3 \in S\), such that \(s_3 \notin \mu ^{-1}(c_2)\) and \({c}_2\) temporarily accepts \(s_3\) at Step \({k}_2\) of the DA algorithm for \({\tilde{G}}\). One can infer that \(s_3 {\tilde{\rhd }}_{{c}_2} s_2\). Let \(c_3 \equiv \mu ({s}_3)\).
After repeating the above procedures for several times, since there are a finite number of steps from Step 1 to Step \(k_0\) of the DA algorithm for \({\tilde{G}}\), there must exist some \(r \ge 1\), such that \({c}_r P_{s_{r+1}} c_{r+1}\), \(c_{r+1} \equiv \mu (s_{r+1})\), and \(s_{r+1} {\tilde{\rhd }}_{{c}_r} s_r\). By Lemma 1, there is \(\mu (s_{r+1}) R_{s_{r+1}}{\tilde{\mu }}(s_{r+1})\), and we obtain \({c}_r P_{s_{r+1}} {\tilde{\mu }}(s_{r+1})\). Since \(s_r \in \mu ^{-1}({c}_r)\), \(s_{r+1} \notin \mu ^{-1}({c}_r)\), and \({c}_r P_{s_{r+1}} \mu (s_{r+1})\), we obtain \(s_r \rhd _{{c}_r} s_{r+1}\) by the stability of \(\mu\). Then we can see that \({\tilde{\rhd }}_{{c}_r}\) improves the priority of \(s_{r+1}\) over \(\rhd _{{c}_r}\). Thus, \(s_{r+1} \in S^m\) and \(s_r \in S^M\). Furthermore, we can see that \(s_{r+1}\) is an interrupter at school \({c}_r\). That is, in the DA algorithm for \({\tilde{G}}\), \({c}_r\) temporarily accepts \(s_{r+1}\) and rejects \(s_r \in \mu ^{-1}(c_r)\) at Step \({k}_r\) of the DA algorithm for \({\tilde{G}}\), and finally \(s_{r+1}\) is rejected by \({c}_r\).
Here, we get another series of rejections. Specifically, at Step \(k_r\) of the DA algorithm for \({\tilde{G}}\), \(c_r\) tentatively accepts \(s_{r+1}\) and rejects \(s_r\) satisfying \(s_r \in S^M\). At Step \(k_{r-1}\), \(s_r\) is accepted by \(c_{r-1}\) but \(s_{r-1}\) is rejected by \(c_{r-1}\). \(\cdots\) At Step \(k_0\), \(s_1\) is accepted by \(c_0\) but \(s^m\) is rejected by \(c_0\). Let \(m \equiv s_{r+1}\) and \(c \equiv c_r\). In combination with the series of rejections in Step 1, we finally obtain a rejection-chain: \(A_0=\big ((m,c),(m^{\prime },c^{\prime });s_r,s_{r-1},\ldots ,s_1,s^m,{\tilde{s}}_1,{\tilde{s}}_2,\ldots ,{\tilde{s}}_{t-1};c_{r-1},\ldots ,c_1,c_0,{\tilde{c}}_1,{\tilde{c}}_2, \ldots ,{\tilde{c}}_{t-1}\big )\).
Rejection-chain \(A_0\) is also an essential chain, as we have \(s^r \in S^M\) and \(s^m \in S^m\). This essential chain can be illustrated by Fig. 3.
Step 3.
The starting point of essential chain \(A_0\) is (m, c) and the ending point is \((m^{\prime },c^{\prime })\). We are going to show that the essential chain above is in a rejection-cycle.
If \((m,c)=(m^{\prime },c^{\prime })\), then we get a rejection-cycle \(\Omega =\{A_0\}\).
If \((m,c) \ne (m^{\prime },c^{\prime })\), then recall that in the DA algorithm for \({\tilde{G}}\), there is a step before Step \({\tilde{k}}_t\), say Step p \((p<{\tilde{k}}_t)\), such that at Step p, student \(s^{\prime }\) satisfying \(s^{\prime } \in S^M\) and \(\mu (s^{\prime })=c^{\prime }\) is rejected by \(c^{\prime }\). Since \(m^{\prime }\) is a minority interrupter at \(c^{\prime }\) and \(m^{\prime }\) is rejected by \(c^{\prime }\) at Step \({\tilde{k}}_t\), suppose that \(m^{\prime }\) proposes to \(c^{\prime }\) at Step \(p^{\prime }\), then there are two possible cases: (i) \(p^{\prime } \le p\); (ii) \(p<p^{\prime }<{\tilde{k}}_t\). We will show that, for either case, if we do not get a rejection-cycle, then we will obtain a series of rejection-chains \(A_0,A_1,\ldots ,A_k\), where \(k\ge 0\), and \(A_1,\ldots ,A_k\) are short rejection-chains which can be written as \(A_1=\big ((m^{\prime },c^{\prime }),(s^{\prime }_1,c^{\prime }))\), \(\cdots\), \(A_k=((s^{\prime }_{k-1},c^{\prime }),(s^{\prime }_k,c^{\prime })\big )\), and there exists a step in the DA algorithm for \({\tilde{G}}\), say Step \(p_0\), such that \(p \le p_0<{\tilde{k}}_t\) and at Step \(p_0\), student \(s^{\prime }_k\) (\(s^{\prime }_k\) should be \(m^{\prime }\) if \(k=0\)) is tentatively accepted by \(c^{\prime }\) but some majority student \(s^*\) satisfying \(\mu (s^*)R_{s^*}c^{\prime }\) is rejected by \(c^{\prime }\).
Case (i): \(p^{\prime } \le p\).
For this case, we know that at Step p of the DA algorithm for \({\tilde{G}}\), \(m^{\prime }\) is tentatively accepted by \(c^{\prime }\) but \(s^{\prime }\) is rejected by \(c^{\prime }\). Then we can let \(s^* \equiv s^{\prime }\) and \(p_0 \equiv p\).
Case (ii): \(p<p^{\prime }<{\tilde{k}}_t\).
For this case, we know that at Step \(p^{\prime }\) of the DA algorithm for \({\tilde{G}}\), \(m^{\prime }\) is tentatively accepted by \(c^{\prime }\). Since \(c^{\prime }\) is already full at Step p, we can infer that some student \(s^{\prime }_1\) who is tentatively accepted by \(c^{\prime }\) at Step \(p^{\prime }-1\) is rejected by \(c^{\prime }\) at Step \(p^{\prime }\). Thus, we obtain that \(s^{\prime }_1 {\tilde{\rhd }}_{c^{\prime }} s^{\prime }\). There are two subcases:
Subcase (i): \(s^{\prime }_1 \in S^M\).
We obtain that \(s^{\prime }_1 \rhd _{c^{\prime }} s^{\prime }\). Since there is \(\mu (s^{\prime })=c^{\prime }\), by stability of \(\mu\), we know that \(\mu (s^{\prime }_1)R_{s_1}c^{\prime }\). Here we let \(s^* \equiv s^{\prime }_1\) and \(p_0 \equiv p^{\prime }\).
Subcase (ii): \(s^{\prime }_1 \in S^m\).
Note that \(s^{\prime }_1\) is a minority interrupter at \(c^{\prime }\). That is, we obtain a short rejection-chain \(A_1=((m^{\prime },c^{\prime }),(s^{\prime }_1,c^{\prime }))\).
If \((m,c)=(s^{\prime }_1,c^{\prime })\), then we get a rejection-cycle \(\Omega =\{A_0,A_1\}\).
If \((m,c)\ne (s^{\prime }_1,c^{\prime })\), what we need to do is to repeat the above procedures. Specifically, we know that \(p \le p^{\prime }-1\).
-
If \(p=p^{\prime }-1\), then we know that at Step p of the DA algorithm for \({\tilde{G}}\), \(s^{\prime }_1\) is tentatively accepted by \(c^{\prime }\) while \(s^{\prime }\) is rejected by \(c^{\prime }\). Then we can let \(s^* \equiv s^{\prime }\) and \(p_0 \equiv p\).
-
If \(p<p^{\prime }-1\), then since \(s^{\prime }_1\) is tentatively accepted by \(c^{\prime }\) at Step \(p^{\prime }-1\) and \(c^{\prime }\) is already full at Step p, there must be another student \(s^{\prime }_2\) such that \(s^{\prime }_2\) is tentatively accepted by \(c^{\prime }\) at Step \(p^{\prime }-2\) but is rejected by \(c^{\prime }\) at Step \(p^{\prime }-1\). Then we can obtain that \(s^{\prime }_1 {\tilde{\rhd }}_{c^{\prime }} s^{\prime }_2 {\tilde{\rhd }}_{c^{\prime }} s^{\prime }\). Again, we consider the student type of \(s^{\prime }_2\) and can infer that \(s^{\prime }_2\) is either a minority interrupter at \(c^{\prime }\) or a majority student satisfying \(\mu (s^{\prime }_2)R_{s^{\prime }_2}c^{\prime }\). Then, we can use similar arguments once more. Finally, if we still have not obtained a rejection-cycle, then we must have a series of rejection-chains \(A_0,A_1,\ldots ,A_k\), where \(k\ge 1\), and for each \(i \in \{1,\ldots ,k\}\), \(A_i\) is a short rejection-chain \(\big ((s^{\prime }_{i-1},c^{\prime }),(s^{\prime }_i,c^{\prime })\big )\) (\(s^{\prime }_{i-1}\) should be \(m^{\prime }\) if \(i=1\)), and at some Step \({\tilde{p}}\) \((p\le {\tilde{p}}<p^{\prime })\) of the DA algorithm for \({\tilde{G}}\), \(s^{\prime }_k\) is tentatively accepted by \(c^{\prime }\) but some majority student sFootnote 9 satisfying \(\mu (s)R_sc^{\prime }\) is rejected by \(c^{\prime }\). Here we let \(s^* \equiv s\) and \(p_0 \equiv {\tilde{p}}\).
With the above discussions, we conclude that, there is either a rejection-cycle, or a series of rejection-chains \(A_0,A_1,\ldots ,A_k\), where \(k\ge 0\), \(A_1=\big ((m^{\prime },c^{\prime }),(s^{\prime }_1,c^{\prime })\big )\), \(\cdots\), \(A_k=\big ((s^{\prime }_{k-1},c^{\prime }),(s^{\prime }_k,c^{\prime })\big )\), and at Step \(p_0\) \((p \le p_0<{\tilde{k}}_t)\) of the DA algorithm for \(G^{\prime }\), student \(s^{\prime }_k\) is tentatively accepted by \(c^{\prime }\) but some majority student \(s^*\) satisfying \(\mu (s^*)R_{s^*}c^{\prime }\) is rejected by \(c^{\prime }\). Next, we will repeat what we do in Step 1 to show that \((s^{\prime }_k,c^{\prime })\), which is the ending point of \(A_k\), is also the starting point of another rejection-chain.
Specifically, since there is \(\mu (s^*)R_{s^*}c^{\prime }\), we know there is \({\tilde{\mu }}(s^*) \in C\) by Lemma 4. Let \(c^{\prime \prime }_1 \equiv {\tilde{\mu }}(s^*)\), then we have \(\mu (s^*)R_{s^*}c^{\prime }P_{s^*}c^{\prime \prime }_1\). Suppose that at Step \(p_1\) of the DA algorithm for \({\tilde{G}}\), student \(s^*\) proposes to school \({c}_1^{\prime \prime }\).
If there exists some step of the DA algorithm for \({\tilde{G}}\), say \(p_1^{\prime } \ge p_1\), such that at this step school \({c}_1^{\prime \prime }\) rejects some student, say \({s}_1^{\prime \prime }\), satisfying \(\mu ({s}_1^{\prime \prime }) R_{{s}_1^{\prime \prime }} {c}_1^{\prime \prime }\), then we obtain \({c}_1^{\prime \prime } P_{{s}_1^{\prime \prime }} {\tilde{\mu }}({s}_1^{\prime \prime })\) and \(\mu ({s}_1^{\prime \prime }) P_{{s}_1^{\prime \prime }} {\tilde{\mu }}({s}_1^{\prime \prime })\). Then, by Lemma 4, we can see that \({\tilde{\mu }}({s}_1^{\prime \prime }) \in C\). Let \({c}_2^{\prime \prime } \equiv {\tilde{\mu }}({s}_1^{\prime \prime })\). Suppose that at Step \(p_2\) \((p_2 > p_1^{\prime })\) of the DA algorithm for \({\tilde{G}}\), student \({s}_1^{\prime \prime }\) proposes to school \({c}_2^{\prime \prime }\).
Again, if there exists some step of the DA algorithm for \(G^{\prime }\), say \(p_2^{\prime } \ge p_2\), such that at this step school \({c}_2^{\prime \prime }\) rejects some student, say \({s}_2^{\prime \prime }\), satisfying \(\mu ({s}_2^{\prime \prime }) R_{{s}_2^{\prime \prime }} {c}_2^{\prime \prime }\), then we repeat the above procedures and can obtain that at some step of the DA algorithm for \(G^{\prime }\), say Step \(p_3\) (\(p_3 > p_2^{\prime }\)), student \({s}_2^{\prime \prime }\) proposes to school \({c}_3^{\prime \prime }\), where \({c}_3^{\prime \prime } \equiv {\tilde{\mu }}({s}_2^{\prime \prime })\).
After repeating the above procedures for several times, since the DA algorithm terminates in finite steps, there must exist some step, say Step \(p_u\) (\(u \ge 1\)), such that, at Step \(p_u\), student \(s^{\prime \prime }_{u-1}\) proposes to school \({c}_u^{\prime \prime }\), where \({c}_u^{\prime \prime } \equiv {\tilde{\mu }}(s^{\prime \prime }_{u-1})\) (\(s^{\prime \prime }_{u-1}\) should be \(s^*\) if \(u=1\)), and from Step \(p_u\) to the final step of the DA algorithm for \({\tilde{G}}\), school \({c}_u^{\prime \prime }\) does not reject any student \(s \in S\) satisfying \(\mu (s) R_{s} {c}_u^{\prime \prime }\). Then by \(|{\tilde{\mu }}^{-1}({c}_u^{\prime \prime })| = |\mu ^{-1}({c}_u^{\prime \prime })|=q_{{c}_u^{\prime \prime }}\) one can infer that, in the DA algorithm for \({\tilde{G}}\), \({c}_u^{\prime \prime }\) rejects some student \(s^{\prime \prime } \in \mu ^{-1}({c}_u^{\prime \prime })\) before Step \(p_u\), which implies that \({c}_u^{\prime \prime }\) has already temporarily accepted \(q_{{c}_u^{\prime \prime }}\) students at the end of Step \(p_u-1\).
Let \({\tilde{S}}\) denote the set of students who are temporarily accepted by \(c^{\prime \prime }_u\) at the end of Step \(p_u-1\) of the DA algorithm for \({\tilde{G}}\). By \(s^{\prime \prime }_{u-1} \in {\tilde{\mu }}^{-1}({c}_u^{\prime \prime })\), we infer that, at Step \(p_u\) of the DA algorithm for \({\tilde{G}}\), \({c}_u^{\prime \prime }\) temporarily accepts \(s^{\prime \prime }_{u-1}\) and rejects some student \({s_u^{\prime \prime }} \in {\tilde{S}}\) satisfying \({c}_u^{\prime \prime } P_{s_u^{\prime \prime }} \mu ({s_u^{\prime \prime }})\). Since \(\mu\) is stable, we infer that \(s \rhd _{{c}_u^{\prime \prime }} {s_u^{\prime \prime }}\) for all \(s \in \mu ^{-1}({c}_u^{\prime \prime })\) and \(s^{\prime \prime } \rhd _{{c}_u^{\prime \prime }} s_u^{\prime \prime }\). However, by the above arguments that \(s^{\prime \prime }_u \in {\tilde{S}}\) and \(s^{\prime \prime }_u\) is rejected by \(c^{\prime \prime }_u\) at a step later than the step at which \(s^{\prime \prime }\) is rejected by \(c^{\prime \prime }_u\), we can get \({s_u^{\prime \prime }} {\tilde{\rhd }}_{{c}_u^{\prime \prime }} s^{\prime \prime }\). Then we obtain that \({\tilde{\rhd }}_{{c}_u^{\prime \prime }}\) improves the priority of \(s_u^{\prime \prime }\) over \(\rhd _{{c}_u^{\prime \prime }}\). Thus, we have \({s_u^{\prime \prime }} \in S^m\) and \(s^{\prime \prime } \in S^M\), and we find that \(s^{\prime \prime }_u\) is a minority interrupter at \(c^{\prime \prime }_u\) in the DA algorithm for \({\tilde{G}}\). Let \(c^{\prime \prime } \equiv c^{\prime \prime }_u\) and \(m^{\prime \prime } \equiv s^{\prime \prime }_u\). We get a new rejection-chain: \(A_{k+1}=\big ((s^{\prime }_k,c^{\prime }),(m^{\prime \prime },c^{\prime \prime });s^*,s^{\prime \prime }_1,s^{\prime \prime }_2,\ldots ,s^{\prime \prime }_{u-1};c^{\prime \prime }_1,c^{\prime \prime }_2,\ldots ,c^{\prime \prime }_{u-1}\big )\), which is illustrated by Figure 4. Note that if \(k=0\), then \(s^{\prime }_k\) should be \(m^{\prime }\).
Again, if \((m^{\prime \prime },c^{\prime \prime })=(m,c)\), then we get a rejection-cycle \(\Omega =\{A_0,\ldots ,A_k,A_{k+1}\}\). Otherwise, with the same arguments as above in Step 3, we can infer that if there is no rejection-cycle, then \((m^{\prime \prime },c^{\prime \prime })\) will initiate another series of rejection-chains, say \(A_{k+2},\ldots ,A_h,A_{h+1}\), where \(h \ge k+1\), and the ending point of \(A_h\) is a minority interrupting pair, say \((m^{\prime \prime \prime },c^{\prime \prime \prime })\). \(\cdots\)
Since the number of minority interrupting pairs is finite, we can eventually get a rejection-cycle \(\Omega =\{A_0,A_1,\ldots ,A_k,A_{k+1},\ldots ,A_h,A_{h+1},\ldots ,A_H,A_{H+1}\}\) containing a finite number of rejection-chains.
Finally, according to Lemma 6, since \(\Omega\) is a rejection-cycle in the DA algorithm for \({\tilde{G}}=\{P,{\tilde{\rhd }}\}\), and \(A_0 \in \Omega\) is an essential chain, and \(s_r\rhd _{c}m\), we can infer that \((S,C,\rhd ,q)\) has a cycle. Then, by Lemma 5, since \(\rhd\) is an improvement for minority students over \(\succ\), we obtain that \((S,C,\succ ,q)\) also has a cycle. The proof is completed. \(\square\)
Four examples
To better understand the proof, we provide several examples in this section, which respectively show how rejection-chains form a rejection-cycle and how a rejection-cycle implies a cycle in the priority structure.
Example 4
This example is an extension of Example 1. Let \(G=(P,\succ )\), \(G^{\prime }=(P,\succ ^{\prime })\), \(S^M=\{M_1,M_2,M_3\}\), \(S^m=\{m_1,m_2\}\), \(C=\{c_1,c_2,c_3,c_4\}\), and \(q_c=1\) for all \(c \in C\). Students’ preferences and schools’ priorities are given by the following table.
\(P_{M_1}\) | \(P_{M_2}\) | \(P_{M_3}\) | \(P_{m_1}\) | \(P_{m_2}\) | \(\succ _{c_1}\) | \(\succ ^{\prime }_{c_1}\) | \(\succ _{c_2}=\succ ^{\prime }_{c_2}\) | \(\succ _{c_3}=\succ ^{\prime }_{c_3}\) | \(\succ _{c_4}=\succ ^{\prime }_{c_4}\) |
|---|---|---|---|---|---|---|---|---|---|
\(c_1\) | \(c_2\) | \(c_4\) | \(c_1\) | \(c_3\) | \(M_3\) | \(M_3\) | \(m_1\) | \(m_1\) | \(m_1\) |
\(c_2\) | \(c_3\) | \(c_1\) | \(m_1\) | \(c_4\) | \(M_1\) | \(m_1\) | \(m_2\) | \(M_2\) | \(m_2\) |
\(M_1\) | \(M_2\) | \(M_3\) | \(m_2\) | \(m_1\) | \(M_1\) | \(M_3\) | \(m_2\) | \(M_1\) | |
\(m_2\) | \(m_2\) | \(M_1\) | \(M_3\) | \(M_3\) | |||||
\(M_2\) | \(M_2\) | \(M_2\) | \(M_1\) | \(M_2\) |
For problem G, the DA procedures are as follows:

For problem \(G^{\prime }\), the DA procedures are as follows:

The above procedures clearly show an essential chain whose ending point, \((m_1,c_1)\), coincides with the starting point. Specifically, we have \(m_1 \in T(M_1,c_1)\), \(M_1 \in T(M_2,c_2)\), \(M_2 \in T(m_2,c_3)\), \(m_2 \in T(M_3,c_4)\), and \(M_3 \in T(m_1,c_1)\). Then, the list \((m_1,M_1,M_2,m_2,M_3;c_1,c_2,c_3,c_4)\) is a cycle. We use Figure 5 to illustrate the rejection-cycle.
The above example is the most simple case of a rejection-cycle. Next, we use two different examples to show the case that two rejection-chains together constitute a rejection-cycle.
Example 5
Let \(S^M=\{M_1,M_2,M_3,M_4,M_5\}\), \(S^m=\{m_1,m_2,m_3\}\), \(C=\{c_1,c_2,c_3,c_4,c_5,c_6\}\), \(q_c=1\) for all \(c \in C\), \(G=(P,\succ )\) and \(G^{\prime }=(P,\succ ^{\prime })\). Students’ preferences and schools’ priorities are given by the following tables.
\(\succ _{c_1}\) | \(\succ ^{\prime }_{c_1}\) | \(\succ _{c_2}=\succ ^{\prime }_{c_2}\) | \(\succ _{c_3}=\succ ^{\prime }_{c_3}\) | \(\succ _{c_4}\) | \(\succ ^{\prime }_{c_4}\) | \(\succ _{c_5}=\succ ^{\prime }_{c_5}\) | \(\succ _{c_6}=\succ ^{\prime }_{c_6}\) |
|---|---|---|---|---|---|---|---|
\(M_5\) | \(M_5\) | \(M_1\) | \(m_2\) | \(M_2\) | \(M_2\) | \(M_3\) | \(M_4\) |
\(M_1\) | \(m_1\) | \(m_2\) | \(M_2\) | \(M_3\) | \(m_3\) | \(M_4\) | \(M_5\) |
\(m_1\) | \(M_1\) | \(\vdots\) | \(\vdots\) | \(m_3\) | \(M_3\) | \(\vdots\) | \(\vdots\) |
\(\vdots\) | \(\vdots\) | \(\vdots\) | \(\vdots\) |
\(P_{M_1}\) | \(P_{M_2}\) | \(P_{M_3}\) | \(P_{M_4}\) | \(P_{M_5}\) | \(P_{m_1}\) | \(P_{m_2}\) | \(P_{m_3}\) |
|---|---|---|---|---|---|---|---|
\(c_1\) | \(c_3\) | \(c_4\) | \(c_5\) | \(c_6\) | \(c_1\) | \(c_2\) | \(c_4\) |
\(c_2\) | \(c_4\) | \(c_5\) | \(c_6\) | \(c_1\) | \(m_1\) | \(c_3\) | \(m_3\) |
\(M_1\) | \(M_2\) | \(M_3\) | \(M_4\) | \(M_5\) | \(m_2\) |
For problem G, the DA procedures are as follows:

For problem \(G^{\prime }\), the DA procedures are as follows:

There are two rejection-chains in the above procedures. One of them is an essential chain, initiated by the minority interrupting pair \((m_1,c_1)\) and ended by the minority interrupting pair \((m_3,c_4)\), and the other one is a general rejection-chain. The ending point of the essential chain is the starting point of the other rejection-chain; also, the ending point of the general rejection-chain is the starting point of the essential chain. Specifically, we have \(m_1 \in T(M_1,c_1)\), \(M_1 \in T(m_2,c_2)\), \(m_2 \in T(M_2,c_3))\), \(M_2 \in T(M_3,c_4)\), \(M_3 \in T(M_4,c_5)\), \(M_4 \in T(M_5,c_6)\), and \(M_5 \in T(m_1,c_1)\), which implies a cycle \((m_1,M_1,m_2,M_2,M_3,M_4,M_5;c_1,c_2,c_3,c_4,c_5,c_6)\). We use Figure 6 to illustrate the rejection-cycle.
Example 6
Let \(S^M=\{M_1,M_2,M_3\}\), \(S^m=\{m_1,m_2,m_3\}\), \(C=\{c_1,c_2,c_3,c_4\}\), \(q_c=1\) for all \(c \in C\), \(G=(P,\succ )\) and \(G^{\prime }=(P,\succ ^{\prime })\). Students’ preferences and schools’ priorities are given by the following table.
\(P_{M_1}\) | \(P_{M_2}\) | \(P_{M_3}\) | \(P_{m_1}\) | \(P_{m_2}\) | \(P_{m_3}\) | \(\succ _{c_1}\) | \(\succ ^{\prime }_{c_1}\) | \(\succ _{c_2}=\succ ^{\prime }_{c_2}\) | \(\succ _{c_3}=\succ ^{\prime }_{c_3}\) | \(\succ _{c_4}=\succ ^{\prime }_{c_4}\) |
|---|---|---|---|---|---|---|---|---|---|---|
\(c_3\) | \(c_1\) | \(c_4\) | \(c_4\) | \(c_1\) | \(c_2\) | \(M_1\) | \(M_1\) | \(M_2\) | \(m_3\) | \(M_3\) |
\(c_1\) | \(c_2\) | \(M_3\) | \(c_1\) | \(m_2\) | \(c_3\) | \(M_2\) | \(m_1\) | \(m_3\) | \(M_1\) | \(m_1\) |
\(M_1\) | \(M_2\) | \(m_1\) | \(m_3\) | \(m_1\) | \(m_2\) | \(\vdots\) | \(\vdots\) | \(\vdots\) | ||
\(m_2\) | \(M_2\) | |||||||||
\(\vdots\) | \(\vdots\) |
For problem G, the DA procedures are as follows:

For problem \(G^{\prime }\), the DA procedures are as follows:

It is easy to see that there is an essential chain whose starting point is \((m_2,c_1)\) and ending point is \((m_1,c_1)\). Actually both \(m_1\) and \(m_2\) are minority interrupters at \(c_1\), so we can find that another rejection-chain contains no other student in addition to the interrupters \(m_1\) and \(m_2\). Then we find a rejection-cycle displayed by Fig. 7. Moreover, since we have \(m_2 \in T(M_2,c_1)\), \(M_2 \in T(m_3,c_2)\), \(m_3 \in T(M_1,c_3))\), and \(M_1 \in T(m_2,c_1)\), we infer that \((S,C,\succ ,q)\) has a cycle \((m_2,M_2,m_3,M_1;c_1,c_2,c_3)\).
Example 7
Let \(S^M=\{M_1,M_2,M_3,M_4,M_5\}\), \(S^m=\{m_1,m_2,m_3,m_4\}\), \(C=\{c_1,c_2,c_3,c_4,c_5,c_6\}\), \(q_c=1\) for all \(c \in C\), \(G=(P,\succ )\) and \(G^{\prime }=(P,\succ ^{\prime })\). Students’ preferences and schools’ priorities are given by the following tables.
\(\succ _{c_1}\) | \(\succ ^{\prime }_{c_1}\) | \(\succ _{c_2}=\succ ^{\prime }_{c_2}\) | \(\succ _{c_3}\) | \(\succ ^{\prime }_{c_3}\) | \(\succ _{c_4}=\succ ^{\prime }_{c_4}\) | \(\succ _{c_5}\) | \(\succ ^{\prime }_{c_5}\) | \(\succ _{c_6}=\succ ^{\prime }_{c_6}\) |
|---|---|---|---|---|---|---|---|---|
\(M_5\) | \(M_5\) | \(M_1\) | \(m_2\) | \(m_2\) | \(M_2\) | \(M_3\) | \(M_3\) | \(M_4\) |
\(M_1\) | \(m_1\) | \(m_2\) | \(M_2\) | \(m_3\) | \(M_3\) | \(M_4\) | \(m_4\) | \(M_5\) |
\(m_1\) | \(M_1\) | \(\vdots\) | \(m_3\) | \(M_2\) | \(\vdots\) | \(m_4\) | \(M_4\) | \(\vdots\) |
\(\vdots\) | \(\vdots\) | \(\vdots\) | \(\vdots\) | \(\vdots\) | \(\vdots\) |
\(P_{M_1}\) | \(P_{M_2}\) | \(P_{M_3}\) | \(P_{M_4}\) | \(P_{M_5}\) | \(P_{m_1}\) | \(P_{m_2}\) | \(P_{m_3}\) | \(P_{m_4}\) |
|---|---|---|---|---|---|---|---|---|
\(c_1\) | \(c_3\) | \(c_4\) | \(c_5\) | \(c_6\) | \(c_1\) | \(c_2\) | \(c_3\) | \(c_5\) |
\(c_2\) | \(c_4\) | \(c_5\) | \(c_6\) | \(c_1\) | \(m_1\) | \(c_3\) | \(m_3\) | \(m_4\) |
\(M_1\) | \(M_2\) | \(M_3\) | \(M_4\) | \(M_5\) | \(m_2\) |
For problem G, the DA procedures are as follows:

For problem \(G^{\prime }\), the DA procedures are as follows:

In the above procedures, \(m_1\) is a minority interrupter at \(c_1\), \(m_3\) is a minority interrupter at \(c_3\), and \(m_4\) is a minority interrupter at \(c_5\). And there are three rejection-chains, one of which is an essential chain started by \((m_1,c_1)\) and ended by \((m_3,c_3)\). For the other two rejection-chains, we call the one initiated by \((m_3,c_3)\) rejection-chain A, and the one initiated by \((m_4,c_5)\) rejection-chain B. The starting point of the essential chain is the ending point of B, and the ending point of the essential chain is the starting point of A. In addition, the ending point of A is the starting point of B. Thus, we have \(m_1 \in T(M_1,c_1)\), \(M_1 \in T(m_2,c_2)\), \(m_2 \in T(M_2,c_3)\), \(M_2 \in T(M_3,c_4)\), \(M_3 \in T(M_4,c_5)\), \(M_4 \in T(M_5,c_6)\), and \(M_5 \in T(m_1,c_1)\), which means that \((S,C,\succ ,q)\) has a cycle \((m_1,M_1,m_2,M_2,M_3,M_4,M_5;c_1,c_2,c_3,c_4,c_5,c_6)\). We use Figure 8 to illustrate the rejection-chains and the rejection-cycle.
Rights and permissions
About this article
Cite this article
Jiao, Z., Shen, Z. & Tian, G. When is the deferred acceptance mechanism responsive to priority-based affirmative action?. Soc Choice Welf 58, 257–282 (2022). https://doi.org/10.1007/s00355-021-01357-4
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00355-021-01357-4