Skip to main content
Springer Nature Link
Log in

Free vibration analysis of non-uniform Euler–Bernoulli beams by means of Bernstein pseudospectral collocation

  • Original Article
  • Published:
Engineering with Computers Aims and scope Submit manuscript

    We’re sorry, something doesn't seem to be working properly.

    Please try refreshing the page. If that doesn't work, please contact support so we can address the problem.

Abstract

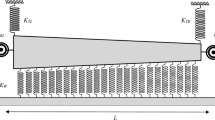
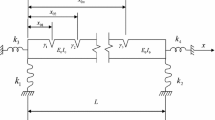
The eigenvalue problem related to the free vibration of Euler–Bernoulli beams of variable cross-section is solved using a collocation technique based on Bernstein polynomials. The properties of the non-orthogonal Bernstein basis allow the construction of the pseudospectral stiffness matrix by recursive formulation and the straightforward enforcement of essential and natural boundary conditions. The approach is tested in benchmark problems of modal analysis, showing high accuracy and exponential convergence rates.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12

Similar content being viewed by others

References

  1. Lorentz GG (1986) Bernstein Polynomials, Second edn. Chelsea Publishing Company, New York

    MATH  Google Scholar 

  2. Fries T, Matthies H (2004) Classification and overview of meshfree methods. Institute of Scientific Computing. Technical University Braunschweig, Brunswick

    Google Scholar 

  3. Doha EH, Bhrawy AH, Saker MA (2011) On the Derivatives of Bernstein Polynomials: An Application for the Solution of High Even-Order Differential Equations. Hindawi Publishing Corporation Boundary Value Problems

  4. Valencia ÓF, Gómez-Escalonilla FJ, Garijo D, López J (2011) Bernstein polynomials in EFGM. Proc IMechE Part C J Mech Eng Sci 225(8):1808–1815

    Article  Google Scholar 

  5. Bhatti MI, Bracken P (2007) Solutions of differential equations in a Bernstein polynomial basis. J Comput Appl Math 205:272–280

    Article  MathSciNet  MATH  Google Scholar 

  6. Mirkov N, Rasuo B (2012) A Bernstein polynomial collocation method for the solution of elliptic boundary value problems. Cornell University Library

  7. Liu J, Zheng Z, Xu Q (2012) Bernstein-polynomials-based highly accurate methods for one-dimensional interface problems. J Appl Math. doi:10.1155/2012/859315

  8. Garijo D, Valencia ÓF, Gómez-Escalonilla FJ, López J (2014) Bernstein–Galerkin approach in elastostatics. Proc IMechE Part C J Mech Eng Sci 228(3):391–404

    Article  Google Scholar 

  9. Garijo D, Gómez-Escalonilla FJ, Valencia ÓF (2014) A coupled FEM-Bernstein approach for computing the \(J_k\) integrals. Arch Appl Mech. doi:10.1007/s00419-014-0893-3

  10. Garijo D, Gómez-Escalonilla FJ, Valencia ÓF (2014) Compared computational performances of Galerkin approximations for perturbed variable-coefficient differential equations, one-dimensional analysis. In: Proceedings of the WCCM XI—ECCM V-ECCM VI, vol III, pp 2688–2699, Barcelona, July 20–25

  11. Hsu J-C, Lai H-Y, Chen CK (2008) Free vibration of non-uniform Euler–Bernoulli beams with general elastically end constraints using Adomian modified decomposition method. J Sound Vib 318(4–5):965–981

    Article  Google Scholar 

  12. Liu Y, Gurram CS (2009) The use of Hes variational iteration method for obtaining the free vibration of an Euler–Bernoulli beam. Math Comput Model 50(11–12):1545–1552

    Article  MathSciNet  MATH  Google Scholar 

  13. Timoshenko S (1937) Vibration problems in engineering, Second edn. D. Van Nostrand Company Inc, New York

    MATH  Google Scholar 

  14. Clough RW, Penzien J (1975) Dynamics of structures. McGraw-Hill Companies, New York

    MATH  Google Scholar 

  15. Paz M (1991) Structural dynamics, theory and computation, Third edn. Van Nostrand Reinhold Company Inc, New York

    Book  Google Scholar 

  16. Stokey WF (2002) Vibration of systems having distributed mass and elasticity. Shock and vibration Handbook, Fifth edn. McGraw-Hill, pp 7–1

  17. Taha MH, Abohadima S (2008) Mathematical model for vibrations of non-uniform flexural beams. Eng Mech 15(1):3–11

    Google Scholar 

  18. Leitão VMA, Tiago C (2002) The use of radial basis functions for one-dimensional structural analysis problems. In: Brebbia CA, Tadeu A, Popov V (eds)Proceedings of the Twenty-Fourth International Conference on the Boundary Element Method. Sintra, Portugal, pp. 165–179 (2002)

  19. Liu GR (2003) Mesh Free Methods. Moving beyond the Finite element Method. CRC Press

  20. Valencia ÓF, Gómez-Escalonilla FJ, López J (2009) The influence of selectable parameters in the element-free Galerkin method: a one-dimensional beam-in-bending problem. Proc IMechE Part C J Mech Eng Sci 223(7):1579–1590

    Article  Google Scholar 

  21. Caruntu DI (2009) Dynamic modal characteristics of transverse vibrations of cantilevers of parabolic thickness. Mech Res Commun 36:391–404

    Article  MathSciNet  MATH  Google Scholar 

  22. Atkinson KE (1988) An introduction to numerical analysis, Second edn. John Wiley & Sons Inc, New York

    Google Scholar 

  23. Chen J-S, Pan C, Wu C-T, Liu WK (1996) Reproducing Kernel Particle Methods for large deformation analysis of non-linear structures. Comput Methods Appl Mech Eng 139:195–227

    Article  MathSciNet  MATH  Google Scholar 

  24. Chen J-S, Pan C, Wu C-T (1997) Large deformation analysis of rubber based on a reproducing kernel particle method. Comput Mech 19:211–227

    Article  MathSciNet  MATH  Google Scholar 

  25. Jüttler B (1998) The dual basis functions for the Bernstein polynomials. Adv Comput Math 8(4):345–352

    Article  MathSciNet  MATH  Google Scholar 

  26. Farouki RT, Goodman TNT, Sauer T (2003) Construction of orthogonal bases for polynomials in Bernstein form on triangular and simplex domains. Comput Aided Geom Des 20:209–230

    Article  MathSciNet  MATH  Google Scholar 

  27. Spivey MZ (2007) Combinatorial sums and finite differences. Discret Math 307:3130–3146

    Article  MathSciNet  MATH  Google Scholar 

  28. Provatidis CG (2008) Free vibration analysis of elastic rods using global collocation. Arch Appl Mech 78(4):241–250

    Article  MATH  Google Scholar 

  29. Kansa EJ (1990) Multiquadrics: a scattered data approximation scheme with applications to computational fluid-dynamics—II: Solutions to parabolic, hyperbolic and elliptic partial differential equations. Comput Math Appl 19(8–9):147–161

    Article  MathSciNet  MATH  Google Scholar 

  30. Naguleswaran S (1994) A direct solution for the transverse vibration of Euler–Bernoulli wedge and cone beams. J Sound Vib 172(3):289–304

    Article  MATH  Google Scholar 

Download references

Acknowledgments

The author wishes to thank the support and collaboration of Safran Group.

Author information

Authors and Affiliations

  1. Safran Engineering Services (Safran Group), Calle Arnaldo de Vilanova, 5, 28906, Getafe, Madrid, Spain

    D. Garijo

Authors
  1. D. Garijo
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to D. Garijo.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Garijo, D. Free vibration analysis of non-uniform Euler–Bernoulli beams by means of Bernstein pseudospectral collocation. Engineering with Computers 31, 813–823 (2015). https://doi.org/10.1007/s00366-015-0401-6

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00366-015-0401-6

Keywords

Mathematics Subject Classification