Abstract
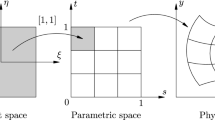
In the analysis of a plate, the geometry plays a very important role. The non-uniform rational B-spline (NURBS) basis functions are employed for the representation of the geometry and field variables in the isogeometric analysis. These basis functions are able to represent the geometry accurately. They are non-interpolating in nature, and hence do not satisfy the Kronecker-Delta property. Hence, it becomes difficult to enforce the essential boundary conditions at the control variables. A new method called NURBS-augmented finite element method (NAFEM) was proposed (Mishra and Barik, Comput Struct, https://doi.org/10.1016/j.compstruc.2017.10.011, 2017) and arbitrary shaped plates were successfully dealt for bending analysis. In the NAFEM, the authors adopted the finite element basis functions for the field variables as they satisfy the Kronecker-Delta property so that the boundary conditions were enforced with ease and the NURBS basis functions were employed for the geometry, thereby representing the shape of the plate accurately. In the present work, the same is extended for stability analysis of plates having different geometries and boundary conditions and the results are found to be in excellent agreement with the existing ones. Some new shapes have also been considered, and the new results are presented.








Similar content being viewed by others
References
Barik M, Mukhopadhyay M (1998) Finite element free flexural vibration analysis of arbitrary plates. Finite Elem Anal Des 29:137–151
Barik M, Mukhopadhyay M (2002) A new stiffened plate element for the analysis of arbitrary plates. Thin-Walled Struct 40:625–639
da Veiga Beirão L, Buffa A, Lovadina C, Martinelli M, Sangalli G (2012) An isogeometric method for the Reissner-Mindlin plate bending problem. Comput Methods Appl Mech Eng 209–212:45–53
Belytschko T, Lu Y, Gu L (1994) Element free Galerkin methods. Int J Numer Meth Engng 37:229–256
Corr RB, Jennings E (1976) A simultaneous iteration algorithm for solution of symmetric eigen value problem. Int J Numer Meth Eng 10:647–663
Embar A, Dolbow J, Harari I (2010) Imposing Dircihlet boundary conditions with Nitsche’s method and spline based finite elements. Int J Numer Meth Engng 83:877–898
Hughes TJR, Cottrell JA, Bazilevs Y (2005) Isogeometric analysis: CAD, finite elements, NURBS, exact geometry and mesh refinement. Comput Methods Appl Mech Eng 194:4135–4195
Liew KM, Xiang Y, Kitipornchai S (1996) Analytical buckling solutions for mindlin plates involving free edges. Int J Mech Sci 10(38):1127–1138
Mishra BP, Barik M (2017) NURBS-augmented finite element method for static analysis of arbitrary plates. Comput Struct. https://doi.org/10.1016/j.compstruc.2017.10.011
Mitchell TJ, Govindjee S, Taylor RL (2011) A method for enforcement of dirichlet boundary conditions in isogeometric analysis. In: Mueller-Hoeppe D, Loehnert S, Reese S (eds) Recent developments and innovative applications in computational mechanics. Springer, Berlin, pp 283–293
Nguyen VP, Anitescu C, Bordas SPA, Rabczuk T (2015) Isogeometric analysis: An overview and computer implementation aspects. Math Comput Simul 117:89–116
Sevilla R, Fernández S, Huerta A (2008) NURBS-enhanced finite element method (NEFEM). Int J Numer Meth Engng 76:56–83
Shojaee S, Izadpanah E, Valizadeh N, Kiendl J (2012) Free vibration analysis of thin plates by using a NURBS-based Isogeometric approach. Finite Elem Anal Des 61:23–34
Shojaee S, Valizadeh N, Izadpanah E, Bui T, Vu TV (2012) Free vibration and buckling analysis of laminated composite plates using the NURBS-based Isogeometric finite element method. Compos Struct 94:1677–1693
Thai CH, Ferreira AJM, Bordas SPA, Rabczuk T, Nguyen-Xuan H (2014) Isogeometric analysis of laminated composite and sandwich plates using a new inverse trigonometric shear deformation theory. Eur J Mech A Solids 43:89–108
Thai CH, Ferreira AJM, Carrera E, Nguyen-Xuan H (2013) Isogeometric analysis of laminated composite and sandwich plates using a layerwise deformation theory. Compos Struct 104:196–214
Thai CH, Nguyen-Xuan H, Bordas SPA, Nguyen-Thanh N, Rabczuk T (2015) Isogeometric analysis of laminated composite plates using the higher-order shear deformation theory. Mech Adv Mater Struct 22(6):451–469
Thai CH, Nguyen-Xuan H, Nguyen-Thanh N, Le TH, Nguyen-Thoi T, Rabczuk T (2012) Static, free vibration, and buckling analysis of laminated composite ReissnerMindlin plates using NURBS-based isogeometric approach. Int J Numer Methods Eng 91(6):571–603
Timoshenko SP, Gere JM (1963) Theroy of elastic stability, 2nd edn. McGraw-Hill International, New York
Valizadeh N, Natrajan S, Gonzalez-Estrada OA, Rabczuk T, Bui TQ, Bordas SPA (2013) NURBS-based finite element analysis of functionally graded plates: Static bending, vibration, buckling and flutter. Compos Struct 99:309–326
Wang X, Zhu X, Hu P (2015) Isogeometric finite element method for buckling analysis of generally laminated composite beams with different boundary conditions. Int J Mech Sci 104:190–199
Woinowsky-Krieger S (1937) The stability of a clamped elliptic plate under uniform compression. J Appl Mech 4(4):177–178
Zhou Y, Zheng X, Harik IE (1995) Buckling of triangular plates under uniform compression. J Appl Mech 5(57):847–854
Zhu T, Atluri SN (1998) A modified collocation method and a penalty formulation for enforcing the essential boundary conditions in Element free Galerkin method. Comp Mech 21:211–222
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Mishra, B.P., Barik, M. NURBS-augmented finite element method for stability analysis of arbitrary thin plates. Engineering with Computers 35, 351–362 (2019). https://doi.org/10.1007/s00366-018-0603-9
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00366-018-0603-9