A variational iteration method (VIM) for nonlinear dynamic response of a cracked plate interacting with a fluid media

Abstract

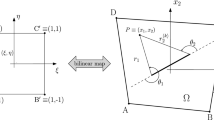
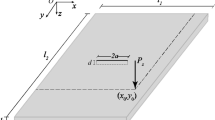
This paper deals with analyzing the nonlinear vibration of an isotropic cracked plate interacting with an air cavity. A part-through surface crack with variable orientations and positions is considered and modeled using the modified line spring model. In the first step, based on the Von Karman theory, the governing equation of the nonlinear vibration related to the cracked plate–cavity is presented. Then, by employing the Euler equation along with the Galerkin method, the coupling effect between the fluid–solid media inside the enclosure is eliminated. In the next step, the variational iteration method (VIM) is introduced as an appropriate method for nonlinear analysis of the mentioned system. To this end, the convergence of the nonlinear coupled natural frequencies with high precision is proved by performing four iterations of VIM. Finally, the effect of the length, angle, and position corresponding to the crack as well as the cavity depth on the frequency ratio is inspected for various boundary conditions by plotting three and four-dimensional backbone curves. It is revealed that the crack angle is the most effective parameter on the frequency ratio.

Appendices

Appendix 1

The coefficients related to the crack in Eq. (1), namely J_n (n = 1…23), are defined as below [7]:

$$J_{1} = 1 - \varphi_{1} \sin^{4} \left( \theta \right) - \frac{1}{2}\varphi_{5} \sin^{2} \left( {2\theta } \right)$$

(31)

$$J_{2} = - \varphi_{1} \sin^{2} \left( \theta \right)\cos^{2} \left( \theta \right) + \frac{1}{2}\varphi_{5} \sin^{2} \left( {2\theta } \right)$$

(32)

$$J_{3} = \frac{1}{2}\varphi_{1} \sin^{2} \left( \theta \right) \sin \left( {2\theta } \right) + \frac{1}{4}\varphi_{5} \sin \left( {4\theta } \right)$$

(33)

$$J_{4} = 1 - \varphi_{4} \cos^{4} \left( \theta \right) - \frac{1}{2}\varphi_{5} \sin^{2} \left( {2\theta } \right)$$

(34)

$$J_{5} = J_{2}$$

(35)

$$J_{6} = \frac{1}{2}\varphi_{1} \cos^{2} \left( \theta \right) \sin^{2} \left( {2\theta } \right) - \frac{1}{4}\varphi_{5} \sin \left( {4\theta } \right)$$

(36)

$$J_{7} = 1 - \frac{1}{2}\varphi_{1} \sin^{2} \left( {2\theta } \right) - \varphi_{5} \cos^{2} \left( {2\theta } \right)$$

(37)

$$J_{8} = \varphi_{1} \sin^{2} \left( \theta \right) \sin \left( {2\theta } \right) + \frac{1}{2}\varphi_{5} \sin \left( {4\theta } \right)$$

(38)

$$J_{9} = \varphi_{1} \cos^{2} \left( \theta \right)\sin \left( {2\theta } \right) - \frac{1}{2}\varphi_{5} \sin \left( {4\theta } \right)$$

(39)

$$J_{10} = \varphi_{4} \left[ {\sin^{2} \left( \theta \right) + \upsilon \cos^{2} \left( \theta \right)} \right]\sin^{2} \left( \theta \right) + \varphi_{8} \left( {1 - \upsilon } \right)\frac{{\sin^{2} \left( {2\theta } \right)}}{2}$$

(40)

$$J_{11} = \varphi_{4} \left[ {\upsilon \sin^{2} \left( \theta \right) + \cos^{2} \left( \theta \right)} \right]\cos^{2} \left( \theta \right) + \varphi_{8} \left( {1 - \upsilon } \right)\frac{{\sin^{2} \left( {2\theta } \right)}}{2}$$

(41)

$$J_{12} = \varphi_{4} \left[ {\upsilon [\sin^{4} \left( \theta \right) + \cos^{4} \left( \theta \right)] + \frac{{\sin^{2} \left( {2\theta } \right)}}{2}} \right] + (\varphi_{4} - \varphi_{8} )\left( {1 - \upsilon } \right)\sin^{2} \left( {2\theta } \right)$$

(42)

$$J_{13} = \varphi_{2} \sin^{4} \left( \theta \right) + \frac{1}{2}\varphi_{6} \sin^{2} \left( {2\theta } \right)$$

(43)

$$J_{14} = \varphi_{2} \sin^{2} \left( \theta \right)\cos^{2} \left( \theta \right) - \frac{1}{2}\varphi_{6} \sin^{2} \left( {2\theta } \right)$$

(44)

$$J_{15} = - \frac{1}{2}\varphi_{2} \sin^{2} \left( \theta \right) \sin \left( {2\theta } \right) - \frac{1}{4}\varphi_{6} \sin \left( {4\theta } \right)$$

(45)

$$J_{16} = J_{14}$$

(46)

$$J_{17} = \varphi_{2} \cos^{4} \left( \theta \right) + \frac{1}{2}\varphi_{6} \sin^{2} \left( {2\theta } \right)$$

(47)

$$J_{18} = - \varphi_{2} \cos^{2} \left( \theta \right) + \frac{1}{4}\varphi_{6} \sin \left( {4\theta } \right)$$

(48)

$$J_{19} = 2J_{15}$$

(49)

$$J_{20} = - \varphi_{2} \sin \left( {2\theta } \right)\cos^{2} \left( \theta \right) + \frac{1}{2}\varphi_{6} \sin \left( {4\theta } \right)$$

(50)

$$J_{21} = \frac{1}{2}\varphi_{2} \sin^{2} \left( {2\theta } \right) + \varphi_{6} \cos^{2} \left( {2\theta } \right)$$

(51)

$$\begin{aligned} J_{22} & = - \left( {1 - \upsilon } \right)\sin \left( {2\theta } \right)\left[ {\varphi_{4} \sin^{2} \left( \theta \right) - \varphi_{8} \left\{ {\cos \left( {2\theta } \right) + \sin \left( {2\theta } \right)} \right\}} \right] \\ & \quad - 2\varphi_{4} \left[ {\sin^{2} \left( \theta \right) + \upsilon \cos^{2} \left( \theta \right)} \right] \\ \end{aligned}$$

(52)

$$J_{23} = \varphi_{8} \left( {1 - \upsilon } \right)\sin \left( {2\theta } \right)\left[ {\cos \left( {2\theta } \right) + \sin \left( {2\theta } \right)} \right] - \varphi_{4} \left[ {\left( {3 - \upsilon } \right)\cos^{2} \left( \theta \right) + 2\upsilon \sin^{2} \left( \theta \right)} \right]$$

(53)

where φ_n (n = 1…8) are expressed as follows:

$$\varphi_{1} = \frac{{1 + \frac{{3\left( {3 + \upsilon } \right)\left( {1 - \upsilon } \right)}}{2}\frac{\gamma }{\varGamma }\alpha_{bb} }}{R}$$

(54)

$$\varphi_{2} = \frac{{\frac{{3\left( {1 - \upsilon^{2} } \right)}}{{\varGamma L_{1} }}\alpha_{bt} }}{R}$$

(55)

$$\varphi_{3} = \frac{{\frac{{\left( {3 + \upsilon } \right)\left( {1 - \upsilon } \right)}}{4}\frac{{\gamma^{2} L_{1} }}{\varGamma }\alpha_{bt} }}{R}$$

(56)

$$\varphi_{4} = \frac{{1 + \frac{{1 - \upsilon^{2} }}{2}\frac{\gamma }{\varGamma }\alpha_{tt} }}{R}$$

(57)

$$\varphi_{5} = \frac{{1 + \frac{{3\left( {1 + \upsilon } \right)}}{2}\frac{\gamma }{\varGamma }c_{bb} }}{T}$$

(58)

$$\varphi_{6} = \frac{{\frac{{3\left( {1 + \upsilon } \right)}}{2}\frac{\gamma }{\varGamma }c_{bt} }}{T}$$

(59)

$$\varphi_{7} = \frac{{\frac{{\left( {1 + \upsilon } \right)}}{4}\frac{{\gamma^{2} L_{1} }}{\varGamma }c_{bt} }}{T}$$

(60)

$$\varphi_{8} = \frac{{1 + \frac{{\left( {1 + \upsilon } \right)}}{2}\frac{\gamma }{\varGamma }c_{tt} }}{T}$$

(61)

where γ and Γ are the non-dimensional plate thickness and crack length, respectively. In addition, R and T are defined as follows:

$$R = \left[ {1 + \frac{{1 - \upsilon^{2} }}{2}\frac{\gamma }{\varGamma }\alpha_{tt} } \right]\left[ {1 + \frac{{3\left( {3 + \upsilon } \right)\left( {1 - \upsilon } \right)}}{2}\frac{\gamma }{\varGamma }\alpha_{bb} } \right] - \frac{{3\left( {1 - \upsilon } \right)\left( {3 + \upsilon } \right)\left( {1 - \upsilon^{2} } \right)}}{4}\left( {\frac{\gamma }{\varGamma }} \right)^{2} \alpha_{bt}^{2}$$

(62)

$$T = \left[ {1 + \frac{{3\left( {1 + \upsilon } \right)}}{2}\frac{\gamma }{\varGamma }C_{bb} } \right]\left[ {1 + \frac{1 + \upsilon }{2}\frac{\gamma }{\varGamma }C_{tt} } \right] - \frac{{3\left( {1 + \upsilon } \right)^{2} }}{4}\left( {\frac{\gamma }{\varGamma }} \right)^{2} C_{bt}^{2}$$

(63)

In (62) and (63), non-dimensional coefficients α_tt, α_bb, α_bt = α_tb are called compliance coefficients defined for symmetric loading (mode I) and introduced by Rice and Levi [1]. Subscripts t and b are corresponding stretching loading and bending loading, respectively. Likewise, the other non-dimensional coefficients C_tt, C_bb, C_bt = C_tb, are employed for anti-symmetric loading. These coefficients, which are a function of the non-dimensional depth of the crack (ξ), have been reported for ξ = 0.7 at the center of the plate as below [7]:

$$\begin{aligned} & \alpha_{tt} = 9.8181,\quad \alpha_{bb} = 2.4367,\quad \alpha_{bt} = \alpha_{tb} = 4.8758 \\ & C_{tt} = 0.503067,\quad C_{bb} = - 0.00395861,\quad C_{bt} = C_{tb} = - 0.045906 \\ \end{aligned}$$

(64)

As the crack is not located at the center of the plate, such coefficients, based on the modified line spring model [62], are multiplied by $2\sqrt {\frac{\varGamma }{\pi \gamma }} \exp \left( { - \frac{{\left( {\varGamma - \xi_{c} } \right)^{2} L_{1}^{2} }}{\gamma /\varGamma }} \right)$ in which $\xi_{c} = \frac{{d_{c} }}{{L_{1} }}$ is called eccentricity ratio or non-dimensional distance between the crack center and the plate center.

Appendix 2

ψ₁₁, ψ₁₂, and ψ₂₁ in Eq. (14) for each kind of boundary conditions are defined in Tables 6, 7 and 8.

Table 6 Acoustic coefficients of simply supported plate

Table 7 Acoustic coefficients of clamped plate

Table 8 Acoustic coefficients of simply supported-clamped plate