Skip to main content
Springer Nature Link
Log in

Peridynamic modeling of dual-phase-lag thermal-moisture coupling in a finite element framework

  • Original Article
  • Published:
Engineering with Computers Aims and scope Submit manuscript

Abstract

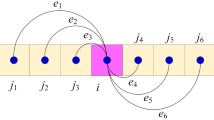
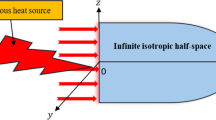
This study presents a peridynamic (PD) modeling approach for non-Fourier heat conduction and non-Fickian moisture concentration in a finite element framework by considering MATRIX27 elements native to ANSYS. The thermal and moisture fields are coupled in the form of Dual-Phase-Lag (DPL) models to resolve the issue of propagation of thermal wave and mass of moisture with infinite speed. The nonlocal effects arising from thermal inertia, moisture inertia and microstructural interaction are included through the PD form of coupled thermal and moisture field equations. The nonlocal PD theory involves integral equations without smoothness requirement of the field variable. The PD thermal and hygro bonds enable the exchange of thermal energy and moisture concentration between nodes. The efficacy of this approach is established by considering heat conduction in a nanoscale metal film and a plate with an insulated crack, and a bar of homogeneous and nonhomogeneous material subjected to sudden pulse of coupled thermal and moisture conditions.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
$34.99 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price excludes VAT (USA)
Tax calculation will be finalised during checkout.

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17
Fig. 18
Fig. 19
Fig. 20
Fig. 21
Fig. 22
Fig. 23

Similar content being viewed by others

References

  1. Sobolev SL (1994) Equations of transfer in non-local media. Int J Heat Mass Transf 37(14):2175–2182

    Article  MATH  Google Scholar 

  2. Chen Z, Akbarzadeh A (2020) Advanced thermal stress analysis of smart materials and structures. Springer, Berlin

    Book  Google Scholar 

  3. Cattaneo C (2011) Sulla conduzione del calore. In: Pignedoli A (eds) Some aspects of diffusion theory. C.I.M.E. Summer Schools, vol 42. Springer, Berlin, pp 485–485

  4. Vernotte P (1958) Les paradoxes de la theorie continue de l’equation de la chaleur. Comptes Rend Acad Bulgare Sci 246:3154–3155

    MATH  Google Scholar 

  5. Tzou DY (1995) The generalized lagging response in small-scale and high-rate heating. Int J Heat Mass Transf 38(17):3231–3240

    Article  Google Scholar 

  6. Tzou DY (1995) A unified field approach for heat conduction from macro-to micro-scales. J Heat Transfer 117(1):8–16

    Article  Google Scholar 

  7. Akbarzadeh AH (2013) Multiphysical behaviour of functionally graded smart structures. In: Department of Mechanical Engineering, University of New Brunswick, Fredericton, NB, Canada

  8. Silling SA (2000) Reformulation of elasticity theory for discontinuities and long-range forces. J Mech Phys Solids 48(1):175–209

    Article  MathSciNet  MATH  Google Scholar 

  9. Bobaru F, Duangpanya M (2010) The peridynamic formulation for transient heat conduction. Int J Heat Mass Transf 53(19–20):4047–4059

    Article  MATH  Google Scholar 

  10. Oterkus S, Madenci E, Agwai A (2014) Peridynamic thermal diffusion. J Comput Phys 265:71–96

    Article  MathSciNet  MATH  Google Scholar 

  11. Oterkus S, Madenci E, Agwai A (2014) Fully coupled peridynamic thermomechanics. J Mech Phys Solids 64:1–23

    Article  MathSciNet  MATH  Google Scholar 

  12. Gerstle W, Silling S, Read D, Tewary V, Lehoucq R (2008) Peridynamic simulation of electromigration. Comput Mater Continua 8(2):75–92

    Google Scholar 

  13. Oterkus S, Madenci E, Oterkus E, Hwang Y, Bae J, Han S (2014) Hygro-thermo-mechanical analysis and failure prediction in electronic packages by using peridynamics. In: 2014 IEEE 64th electronic components and technology conference (ECTC) (pp 973–982), IEEE

  14. Oterkus S, Fox J, Madenci E (2013) Simulation of electro-migration through peridynamics. In: 2013 IEEE 63rd electronic components and technology conference (pp 1488–1493), IEEE

  15. Madenci E, Oterkus E (2014) Peridynamic theory and its applications. Springer, New York

    Book  MATH  Google Scholar 

  16. Madenci E, Barut A, Dorduncu M (2019) Peridynamic differential operator for numerical analysis. Springer International Publishing, Berlin

    Book  MATH  Google Scholar 

  17. Wang L, Xu J, Wang J (2018) A peridynamic framework and simulation of non-Fourier and nonlocal heat conduction. Int J Heat Mass Transf 118:1284–1292

    Article  Google Scholar 

  18. Diyaroglu C, Oterkus S, Oterkus E, Madenci E, Han S, Hwang Y (2017) Peridynamic wetness approach for moisture concentration analysis in electronic packages. Microelectron Reliab 70:103–111

    Article  Google Scholar 

  19. Xue Z, Tian X, Liu J (2020) Hygrothermoelastic response in a hollow cylinder considering dual-phase-lag heat-moisture coupling. Z Angew Math Phys 71(1):1–16

    Article  MathSciNet  MATH  Google Scholar 

  20. Madenci E, Barut A, Futch M (2016) Peridynamic differential operator and its applications. Comput Methods Appl Mech Eng 304:408–451

    Article  MathSciNet  MATH  Google Scholar 

  21. Madenci E, Dorduncu M, Barut A, Futch M (2017) Numerical solution of linear and nonlinear partial differential equations using the peridynamic differential operator. Numer Methods Part Differ Equ 33:1726–1753

    Article  MathSciNet  MATH  Google Scholar 

  22. Macek RW, Silling SA (2007) Peridynamics via finite element analysis. Finite Elem Anal Des 43(15):1169–1178

    Article  MathSciNet  Google Scholar 

  23. Madenci E, Oterkus S (2016) Ordinary state-based peridynamics for plastic deformation according to von Mises yield criteria with isotropic hardening. J Mech Phys Solids 86:192–219

    Article  MathSciNet  Google Scholar 

  24. ANSYS.2 (2017) Mechanical User’s Guide.

  25. Diyaroglu C, Madenci E, Phan N (2019) Peridynamic homogenization of microstructures with orthotropic constituents in a finite element framework. Compos Struct 227:111334

    Article  Google Scholar 

  26. Brorson SD, Fujimoto JG, Ippen EP (1987) Femtosecond electronic heat-transport dynamics in thin gold films. Phys Rev Lett 59(17):1962

    Article  Google Scholar 

  27. Qiu TQ, Tien CL (1994) Femtosecond laser heating of multi-layer metals—I. Analysis. Int J Heat Mass Transfer 37(17):2789–2797

    Article  Google Scholar 

Download references

Acknowledgements

This study was performed as part of the ongoing research at the MURI Center for Material Failure Prediction through Peridynamics at the University of Arizona (AFOSR Grant no. FA9550-14-1-0073)

Author information

Authors and Affiliations

  1. Department of Aerospace and Mechanical Engineering, The University of Arizona, Tucson, AZ, 85721, USA

    Sundaram Vinod K. Anicode & Erdogan Madenci

Authors
  1. Sundaram Vinod K. Anicode
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Erdogan Madenci
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Erdogan Madenci.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Anicode, S.V.K., Madenci, E. Peridynamic modeling of dual-phase-lag thermal-moisture coupling in a finite element framework. Engineering with Computers 39, 911–923 (2023). https://doi.org/10.1007/s00366-022-01698-3

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00366-022-01698-3

Keywords