Abstract
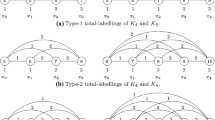
A labelling of a graph G is a mapping \(\pi :S \rightarrow {\mathcal {L}}\), where \({\mathcal {L}}\subset {\mathbb {R}}\) and \(S\in \{E(G), V(G)\cup E(G)\}\). If \(S=E(G)\), \(\pi\) is an \({\mathcal {L}}\)-edge-labelling and, if \(S=V(G)\cup E(G)\), \(\pi\) is an \({\mathcal {L}}\)-total-labelling. For each \(v\in V(G)\), the colour of v under \(\pi\) is defined as \(c_{\pi }(v) = \sum _{uv \in E(G)}{\pi (uv)}\) if \(\pi\) is an \({\mathcal {L}}\)-edge-labelling; and \(c_{\pi }(v) = \pi (v)+\sum _{uv \in E(G)}{\pi (uv)}\) if \(\pi\) is an \({\mathcal {L}}\)-total-labelling. The pair \((\pi ,c_{\pi })\) is a neighbour-distinguishing \({\mathcal {L}}\)-edge-labelling (neighbour-distinguishing \({\mathcal {L}}\)-total-labelling) if \(\pi\) is an \({\mathcal {L}}\)-edge-labelling (\({\mathcal {L}}\)-total-labelling) and \(c_{\pi }(u)\ne c_{\pi }(v)\) for every edge \(uv \in E(G)\). In this work, we show that split graphs, regular cobipartite graphs, complete multipartite graphs and cubic graphs have neighbour-distinguishing \(\{a,b,c\}\)-edge-labellings, for distinct \(a,b,c \in {\mathbb {R}}\) (in some cases \(a,b,c \ge 0\)). For split graphs and regular cobipartite graphs we also prove they admit neighbour-distinguishing \(\{a,b\}\)-total-labellings. Furthermore, we show that flower snarks and some subfamilies of split graphs and regular cobipartite graphs have neighbour-distinguishing \(\{a,b\}\)-edge-labellings and prove that some families of split graphs do not have neighbour-distinguishing \({\mathcal {L}}\)-edge-labellings, for \({\mathcal {L}} = \{a,2a\}\) and \({\mathcal {L}}=\{0,a\}\), \(a,b \in {\mathbb {R}}\backslash \{0\}\), \(a \ne b\).




Similar content being viewed by others
References
Addario-Berry, L., Aldred, R.E.L., Dalal, K., Reed, B.A.: Vertex colouring edge partitions. J. Comb. Theory Ser. B 94(2), 237–244 (2005)
Addario-Berry, L., Dalal, K., McDiarmid, C., Reed, B.A., Thomason, A.: Vertex-coloring edge-weightings. Combinatorica 27(1), 1–12 (2007)
Bensmail, J.: The vertex-colouring \(\{a,b\}\)-edge-weighting problem is NP-complete for every pair of weights. Technical report. University of Bordeaux, LABRI. (2013). https://hal.archives-ouvertes.fr/hal-00826346/document. Accessed 12 May 2022
Chang, G.J., Lu, C., Wu, J., Yu, Q.: Vertex-coloring edge-weightings of graphs. Taiwan. J. Math. 15(4), 1807–1813 (2011)
Davoodi, A., Omoomi, B.: On the 1-2-3-conjecture. Discrete Math. Theor. Comput. Sci. 17(1), 67–78 (2015)
Dudek, A., Wajc, D.: On the complexity of vertex-coloring edge-weightings. Discrete Math. Theor. Comput. Sci. 13(3), 45–50 (2011)
Escuadro, H., Okamoto, F., Zhang, P.: A three-color problem in graph theory. Bull. ICA 52, 68–82 (2008)
Gao, Y., Wang, G., Wu, J.: A relaxed case on 1-2-3 conjecture. Graphs Comb. 32(4), 1415–1421 (2016)
Hulgan, J., Lehel, J., Ozeki, K., Yoshimoto, K.: Vertex Coloring of Graphs by Total 2-Weightings. Graphs Comb. 32(6), 2461–2471 (2016)
Kalkowski, M.: Metody algorytmiczne w badaniach siły nieregularności grafów. Ph.D. thesis, Uniwersytet im. Adama Mickiewicza, Wydział Matematyki i Informatyki (2010) (in Polish)
Kalkowski, M., Karoński, M., Pfender, F.: Vertex-coloring edge-weightings: toward the 1-2-3-conjecture. J. Comb. Theory Ser. B 100(3), 347–349 (2010)
Karoński, M., Łuczak, T., Thomason, A.: Edge weights and vertex colours. J. Comb. Theory Ser. B 91(1), 151–157 (2004)
Khatirinejad, M., Naserasr, R., Newman, M., Seamone, B., Stevens, B.: Vertex-colouring edge-weightings with two edge weights. Discrete Math. Theor. Comput. Sci. 14(1), 1–20 (2012)
Lu, H., Yu, Q., Zhang, C.Q.: Vertex-coloring 2-edge-weighting of graphs. Eur. J. Combin. 32(1), 21–27 (2011)
Paramaguru, N., Sampathkumar, R.: Graphs with vertex-coloring and detectable \(2\)-edge-weighting. AKCE Int. J. Graphs Comb. 13, 146–156 (2016)
Przybyło, J., Woźniak, M.: On a 1,2 conjecture. Discrete Math. Theor. Comput. Sci. 12(1), 101–108 (2010)
Seymour, P.D.: Nowhere-zero flows. In: Graham, R.L., Grotschel, M., Lovasz, L. (eds.) Handbook of Combinatorics, pp. 289–299. North-Holand, Amsterdam (1995)
Tait, P.G.: Remarks on the colouring of maps. Proc. R. Soc. Edinb. 10(4), 501–503 (1880)
Thomassen, C., Wu, Y., Zhang, C.Q.: The 3-flow conjecture, factors modulo k, and the 1–2-3-conjecture. J. Comb. Theory Ser. B 121, 308–325 (2016). https://doi.org/10.1016/j.jctb.2016.06.010
Vučković, B.: Multi-set neighbour-distinguishing 3-edge coloring. Discrete Math. 341(3), 820–824 (2018)
Acknowledgements
This work was partially supported by São Paulo Research Foundation (FAPESP) grant 2014/16861-8; Conselho Nacional de Desenvolvimento Científico e Tecnológico - CNPq grants 423833/2018-9 and 422912/2021-2. We also thank Sheila M. de Almeida for fruitful discussions that contributed to this work.
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
About this article
Cite this article
Luiz, A.G., Campos, C.N. Neighbour-Distinguishing Labellings of Families of Graphs. Graphs and Combinatorics 38, 95 (2022). https://doi.org/10.1007/s00373-022-02492-0
Received:
Revised:
Accepted:
Published:
DOI: https://doi.org/10.1007/s00373-022-02492-0